10.6: Horizontal Motion
- Page ID
- 9594
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)10.6.1. Equations of Motion — Again
The geostrophic wind can be used as a surrogate for the pressure-gradient force, based on the definitions in eqs. (10.26). Thus, the equations of horizontal motion (10.23) become:
\(\ \begin{align} \frac{\Delta U}{\Delta t}=-U \frac{\Delta U}{\Delta x}-V \frac{\Delta U}{\Delta y}-W \frac{\Delta U}{\Delta z}+f_{c} \cdot\left(V-V_{g}\right)-w_{T} \cdot \frac{U}{z_{i}}\tag{10.51a}\end{align}\)
\(\ \begin{align} \frac{\Delta V}{\Delta t}=-U \frac{\Delta V}{\Delta x}-V \frac{\Delta V}{\Delta y}-W \frac{\Delta V}{\Delta z}-f_{c} \cdot\left(U-U_{g}\right)-w_{T} \cdot \frac{V}{z_{i}}\tag{10.51b}\end{align}\)

For winds turning around a circle, you can add a term for centrifugal force, which is an artifice to account for the continual changing of wind direction caused by an imbalance of the other forces (where the imbalance is the centripetal force).
The difference between the actual and geostrophic winds is the ageostrophic wind (Uag, Vag). The term in eqs. (10.51) containing these differences indicates the geostrophic departure.
\(\ \begin{align} U_{\mathrm{ag}}=U-U_{\mathrm{g}}\tag{10.52a}\end{align}\)
\(\ \begin{align} V_{\mathrm{ag}}=V-V_{\mathrm{g}}\tag{10.52b}\end{align}\)
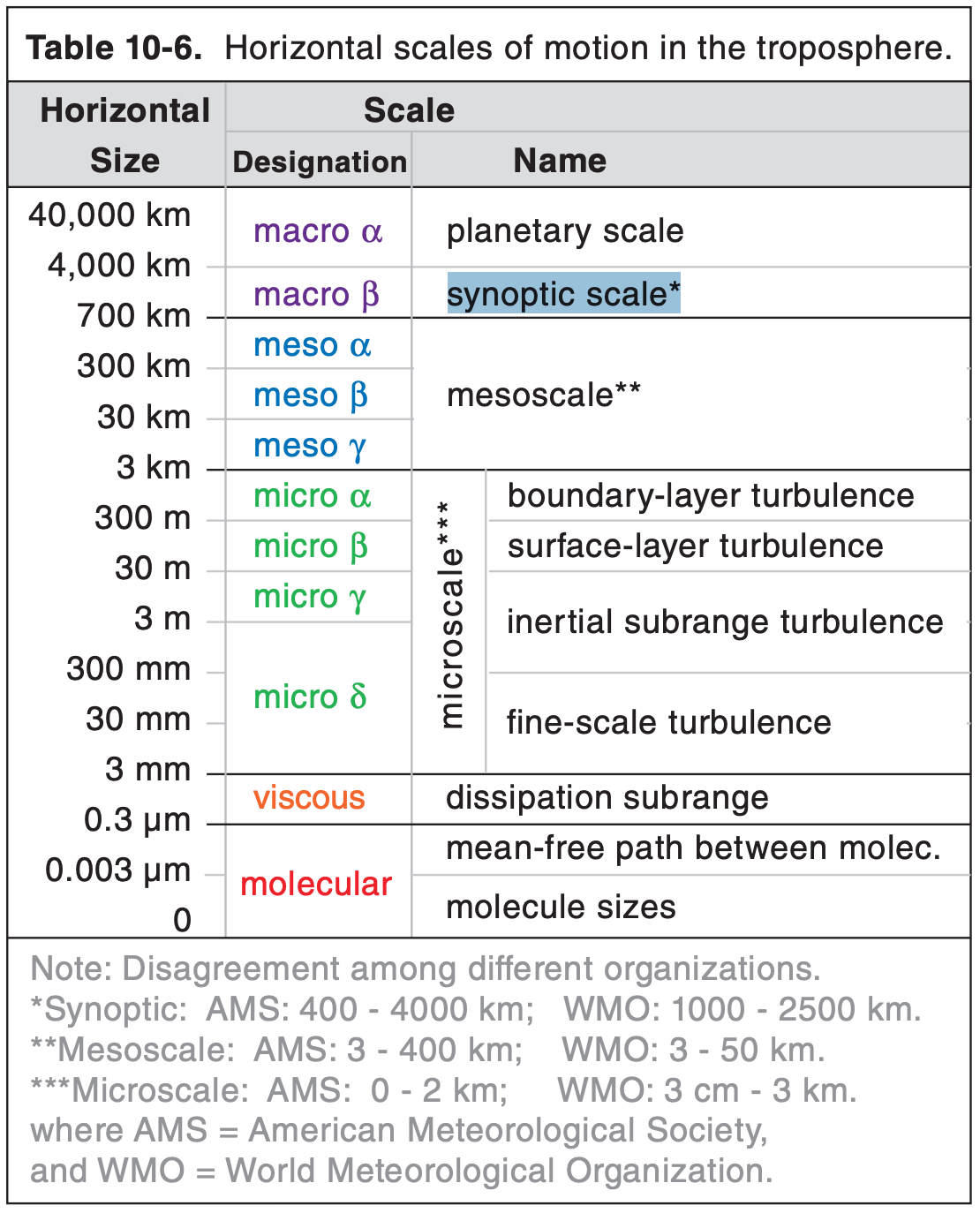
10.6.2. Scales of Horizontal Motion
A wide range of horizontal scales of motion (Table 10-6) are superimposed in the atmosphere: from large global-scale circulations through extra-tropical cyclones, thunderstorms, and down to swirls of turbulence.
The troposphere is roughly 10 km thick, and this constrains the vertical scale of most weather phenomena. Thus, phenomena of large horizontal scale will have a constrained vertical scale, causing them to be similar to a pancake. However, phenomena with smaller horizontal scale can have aspect ratios (width/height) of about one; namely, their characteristics are isotropic.

Larger-scale meteorological phenomena tend to exist for longer durations than smaller-scale ones. Fig. 10.24 shows that time scales τ and horizontal length scales λ of many meteorological phenomena nearly follow a straight line on a log-log plot. This implies that
\(\ \begin{align} \tau / \tau_{0}=\left(\lambda / \lambda_{o}\right)^{b}\tag{10.53}\end{align}\)
where \(\ \tau_o\) ≈ 10–3 h, λo ≈ 10–3 km, and b ≈ 7/8.
In the next several chapters, we cover weather phenomena from largest to smallest horiz. scales:
- Chapter 11 General Circulation (planetary)
- Chapter 12 Fronts & Airmasses (synoptic)
- Chapter 13 Extratropical Cyclones (synoptic)
- Chapter 14 Thunderstorm Fundam. (meso β)
- Chapter 15 Thunderstorm Hazards (meso γ)
- Chapter 16 Tropical Cyclones (meso α & β)
- Chapter 17 Regional Winds (meso β & γ)
- Chapter 18 Atm. Boundary Layers (microscale)
Although hurricanes are larger than thunderstorms, we cover thunderstorms first because they are the building blocks of hurricanes. Similarly, midlatitude cyclones often contain fronts, so fronts are covered before extratropical cyclones.


