9.3: Balance of Forces and Dimensional Analysis
- Page ID
- 4206
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)We could take either of two approaches at this point: make a dimensional analysis to develop a graphical framework for expressing and rationally organizing observational results, or try to develop an analytical solution. First I will outline the classical analytical approach, to see where it leads. That approach involves assuming that the particle is pivoted out of its position, around its two downstream contact points, when the moment due to the fluid force finally becomes larger than the opposing moment due to the weight of the particle. Unfortunately this analytical approach turns out to be of little more help than a simple dimensional analysis, for two reasons: irregularity of geometry, and complexities of the fluid force itself.
Balance of Forces
Particles begin to move on the bed when the combined lift and drag forces produced by the fluid become large enough to counteract the gravity and frictional forces that hold the particle in place. It is impossible to define the balance of forces or moments acting on particles uniquely for all grains: some particles lie in positions from which they are more easily lifted, slid, or rolled than others. It is equally impossible to define a single fluid force that applies to all particles: some particles are more exposed to the flow and subjected to larger fluid forces than other particles, and the fluid forces at the bed fluctuate with time because of turbulence in the flow.
We will begin by considering an average particle, in an average position on the bed, subjected to an average fluid force; we will return later to the problem of an appropriate definition of these averages. To simplify matters further, assume that friction prevents sliding of one particle past another and that the moving particle simply pivots about an axis normal to the flow direction. The condition for the beginning of motion then is that the moments tending to rotate the particle downstream are just balanced by the moments (in the opposite sense) that tend to hold the grain in place (Figure 9.2.4).
To determine the fluid-force moment exactly, we would have to sum all the products of the forces times their normal distances from the lines of action to the pivot axis. We can simplify further by assuming that the bed is horizontal and by considering, at first, only drag forces. Then it is convenient to consider only those components of the gravity and drag forces that act normal to the line joining the pivot to the center of gravity of the particle.
The total moment produced by summing body forces (like the gravity forces acting on each element of volume making up the particle) is the same as the total force times the distance of the center of gravity from the pivot. You can readily see that if we divide the gravity force into two components, normal to and parallel to the line joining the pivot to the center of gravity, then the moment due to the second of these components must be equal to zero, because that component has a line of action passing through the pivot itself. So we can write the condition for the beginning of movement as
\[a_{1}\left(F_{G} \sin \alpha\right)=a_{2}(F_{D} \cos \alpha) \label{9.1} \]
The left side of Equation \ref{9.1} is the total moment due to gravity, which tends to rotate the grain upstream about the pivot or to hold it in place against the moment due to fluid drag forces tending to rotate the particle downstream. The right side represents this fluid-drag moment in a purely conventional way. The drag moment must actually be calculated as the integral of all the products of the drag forces acting on each element of the surface, times the normal distance of each of these forces from the pivot. But because we do not know the distribution of the drag forces over the surface of the particle, there is no way we can actually evaluate that integral, so it is represented conventionally simply as a product of the total component of drag, \(F_{D} \cos \alpha\), which opposes the total component of gravity, \(F_{G} \sin \alpha\), times a normal distance \(a_{2}\). The value of \(a_{2}\) cannot be determined analytically, so \(a_{2}\) is actually a “fudge factor” that is chosen to make the equation balance.
The gravity force \(F_{G}\) can be written
\[F_{G}=c_{1} D^{3} \gamma^{\prime} \label{9.2} \]
where \(c_{1}\) is a coefficient that takes account of the particle shape. The fluid drag force \(F_{D}\) can be assumed equal to the average boundary shear stress to times the area of the grain, and can be written
\[F_{D}=c_{2} D^{2} \tau_{\mathrm{o}} \label{9.3} \]
where the coefficient \(c_{2}\) takes into account not only the geometry and packing of the grains (which determines the “area of the grain”) but also the variation of the drag coefficient. Thus \(c_{2}\) can be expected to vary with boundary Reynolds number. Substituting Equations \ref{9.2} and \ref{9.3} for \(F_{G}\) and \(F_{D}\) into Equation \ref{9.1} and writing \(\tau_{\text{o}} = \tau_{c}\) for the critical condition gives
\[a_{1} c_{1} D^{3} \gamma^{\prime} \sin \alpha=a_{2} c_{2} D^{2} \tau_{c} \cos \alpha label{9.4} \]
or, solving for \(\tau_{c}\),
\[\tau_{c}=\frac{a_{1} c_{1}}{a_{2} c_{2}} \gamma^{\prime} D \tan \alpha \label{9.5} \]
Equation \ref{9.5} can be made dimensionless by dividing both sides by \(\gamma^{\prime} D\):
\[\beta_{c}=\frac{\tau_{c}}{\gamma^{\prime} D}=\frac{a_{1} c_{1}}{a_{2} c_{2}} \tan \alpha \label{9.6}\]
where \(\beta_{c}\), the critical value of a dimensionless variable \(\tau_{0} / \gamma^{\prime} D\), called Shields’ \(\bf{\beta}\) or the Shields' parameter, should be expected to be a function of grain geometry and boundary Reynolds number. (The Shields parameter is named after an American engineer who first put the study of incipient movement on a rational basis in the 1930s while working at a hydraulics laboratory in Germany.)
What Equation \ref{9.6} tells us is that the Shields parameter is a function of a term that itself depends on various effects, both geometrical and dynamical.
The quantities \(a_{1}\), \(c_{1}\), and \(\tan \alpha\) are geometrical, and depend on the grain shape and grain packing. The quantities \(a_{2}\) and \(c_{2}\) are partly also geometrical, but they also include a dependence on the details of flow around the grains and the resulting distributions of pressure forces and viscous forces, and they are therefore a function of the boundary Reynolds number. We cannot proceed any further than Equation \ref{9.6} without knowing more about the details of this \(Re_{*}\) dependence, to say nothing of the problem of taking account of particle shape and packing.
The foregoing analysis is not much different if lift is considered as well as drag, because there should be a proportionality between the two forces which also depends only on grain geometry and boundary Reynolds number.
In deriving Equation \ref{9.6} it was assumed that the bed slope is negligibly small. If this is not the case, then it is easily shown that \(\sin \alpha\) in the Equation \ref{9.6} should be replaced by \(\sin (\alpha-\phi)\), where \(\phi\) is the slope angle (positive in the downstream direction). So, if other conditions remain the same, increasing bed slope decreases the critical value of \(\beta\).
Many other theoretical approaches to incipient motion, along the same lines as that above but taking other effects, like lift forces and bed slope, into consideration as well, have appeared in the literature. None takes us much further than the foregoing simplified analysis.
Dimensional Analysis
The list of variables that should describe the condition of incipient movement is fairly straightforward (Figure \(\PageIndex{1}\)): \(\tau_{\text{o}}\), \(D\), \(\rho\), \(\mu\), \(\rho_{s}\), and \(\gamma^{\prime}\). Flow depth should not be important, because the particles are in the inner layer of a turbulent boundary layer (see Chapter 4 of Part I), in which only the local structure of the flow governs the forces felt by the bed particles.
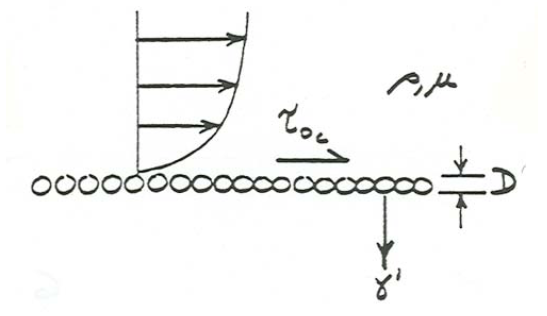
You might think that the sediment density \(\rho_{s}\) has no business here, because the sediment is not moving (by definition). In reality it might be important, however, because it affects the time scale of the response of the particle to a sudden acceleration of the flow: other things being equal, the denser the particle the less rapidly it would accelerate in response to a sudden increase in resultant fluid force to a value large enough to move the particle. And that is important for incipient movement, because the particle might be rocked out of its position of rest by a passing unusually strong eddy, only to fall back to it and undergo no permanent displacement.
Two points about the list of variables above deserve further comment. The first has to do with the choice of \(\tau_{\text{o}}\) as the variable that characterizes the strength of the flow. Because in the material in earlier chapters on flow around a sphere the drag force was related to a velocity, you might reasonably ask why not use a velocity rather than \(\tau_{\text{o}}\). One answer is that, after all, what is moving the grains is basically a force acting on the bed, so the boundary shear stress is a more logical choice than any velocity. (You might reasonably respond that the force itself is caused by the local velocity of flow around the grains.) Another answer is that it is difficult to specify exactly which velocity should be used. The most easily measured velocities (the mean velocity of flow \(U\) or the surface velocity \(U_{s}\)) are not, in any clear or straightforward way, related to the velocity measured near the bed, which is what determines the force that tends to move the grains. If we were to use the mean velocity we would have to add another variable, the depth of flow, because the same mean velocities may give rise to different near-bed velocities, or shear stresses, if the flow depth is different. To get around these problems it has always seemed most natural to use \(\tau_{\text{o}}\) instead of a velocity—but remember that a graph or criterion for incipient movement in terms of \(\tau_{\text{o}}\) (like the famous Shields diagram, introduced below) can always be recast into a form that involves flow velocity and flow depth, if it is velocity that you are most interested in.
The second point is that in listing the variables I have chosen to combine the gravity \(g\) and the sediment density \(\rho_{s}\) into a single variable with the fluid density: \(\gamma^{\prime}=g\left(\rho_{s}-\rho\right)\). This is equivalent to assuming that the only important effect of both gravity and particle density is in controlling the submerged weight of the particle. We assume that surface gravity waves in the fluid are not important—which is equivalent to assuming that the flow is not shallow enough so that the motion of the fluid over the grains affects the free surface. This is clearly an invalid assumption for very shallow, gravel-bed rivers.
So you should expect to deal with three independent dimensionless variables, and therefore to be able to express the condition for incipient movement as a surface in a three-dimensional graph. One of these can be the density ratio \(\rho_{S} / \rho\). The other two then have to involve \(\tau_{\text{o}}\), \(D\), \(\mu\), and \(\gamma^{\prime}\). The traditional variables have been the boundary Reynolds number \(\rho u_{*} D / \mu\) and the Shields parameter \(\tau_{0} / \gamma^{\prime} D\), already introduced above.
\[\text{threshold} =f\left(\rho, \mu, \gamma, D, \tau_{0}\right) \label{9.7} \]
and, nondimensionalizing,
\[\frac{\tau_{c}}{\gamma^{\prime} D}=f\left(\frac{\rho u_{*} D}{\mu}\right) \label{9.8} \]
where \(\tau_{c}\) is the threshold value of the bed shear stress.
You already know about the hydraulic significance of the boundary Reynolds number: it characterizes the nature or structure of the flow near the bed. And recall from Chapter 8 that the Shields parameter also has a real physical meaning: by multiplying the top and bottom of the Shields parameter by \(D^{2}\) you can see that it is proportional to the ratio of fluid force on the particle to the weight of the particle. The effect of the density ratio \(\rho_{s}/ \rho\) is still unclear, but is known not to be large, and anyway most sediment problems involve quartz-density sediment in water.
So just by looking at the dimensional structure of the problem of incipient movement, we have arrived at the same conclusion as from the force-balance analysis, expressed by Equation \ref{9.6}, in the preceding section.


