22.1: Ray Geometry

When a monochromatic (single color) light ray reaches an interface between two media such as air and water, a portion of the incident light from the air can be reflected back into the air, some can be refracted as it enters the water (Fig. 22.1), and some can be absorbed and changed into heat (not sketched). Similar processes occur across an air-ice interface.

22.1.1. Reflection

The angle θ₃ of the reflected ray always equals the angle θ₁ of the incident ray, measured with respect to a line normal (perpendicular) to the interface:

\begin{align}\theta_{1}=\theta_{3}\tag{22.1}\end{align}

Figure 22.1 Geometric optics at an air-water interface. Black arrows are light-ray paths.

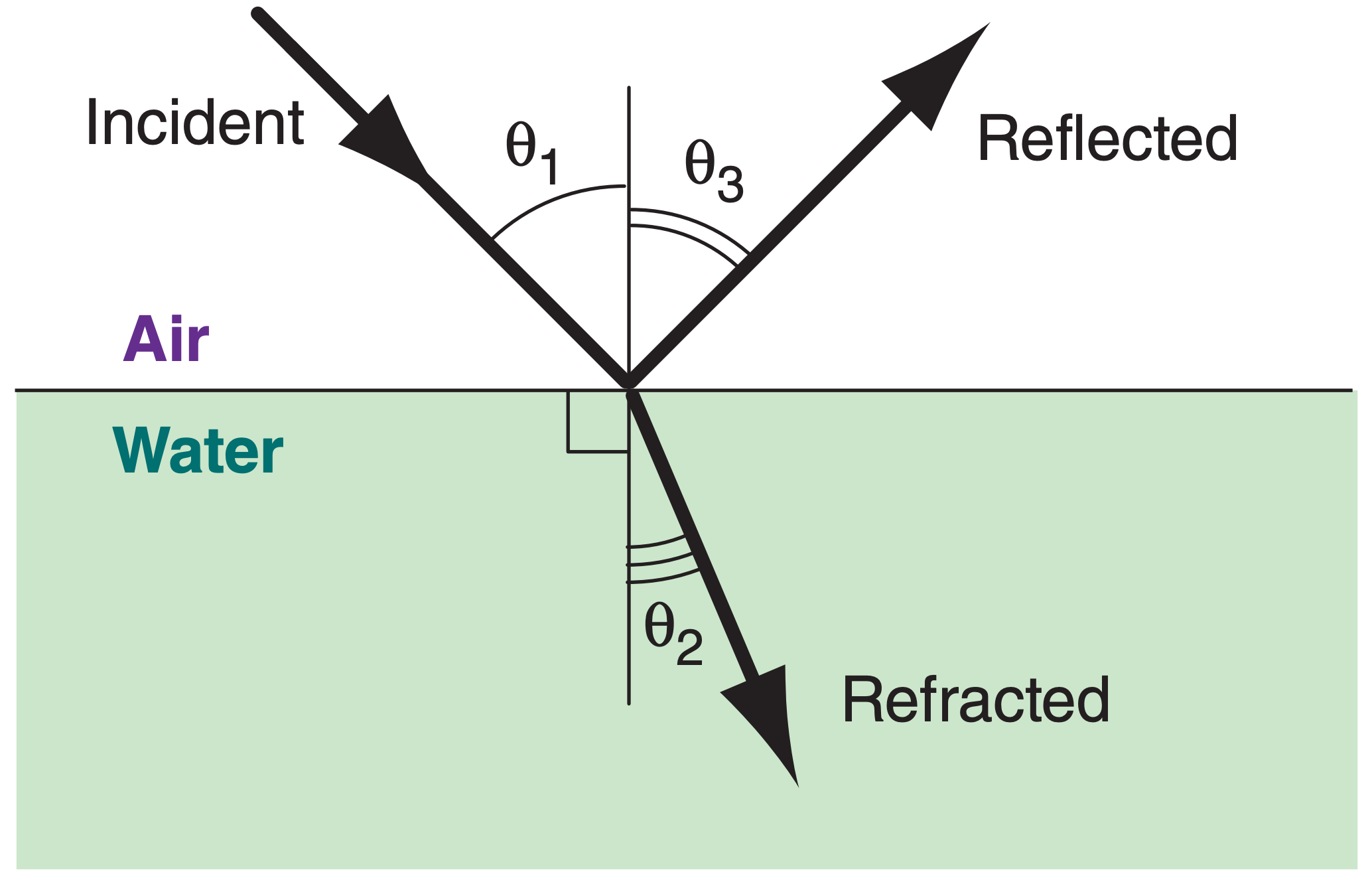
The reflected angle does not depend on color (i.e., is not a function of the wavelength of light).

22.1.2. Refraction

22.1.2.1. Refractive index

The refractive index n_i for medium i relative to a vacuum is defined as:

\begin{align}n_{i}=\dfrac{c_{o}}{c_{i}}\tag{22.2}\end{align}

where c_i is the speed of light through medium i and co = 3 x 10⁸ m s^–1 is the speed of light in a vacuum.

The ratio of refractive indices is sometimes defined as:

\begin{align}\mu_{12}=\dfrac{n_{1}}{n_{2}}\tag{22.3}\end{align}

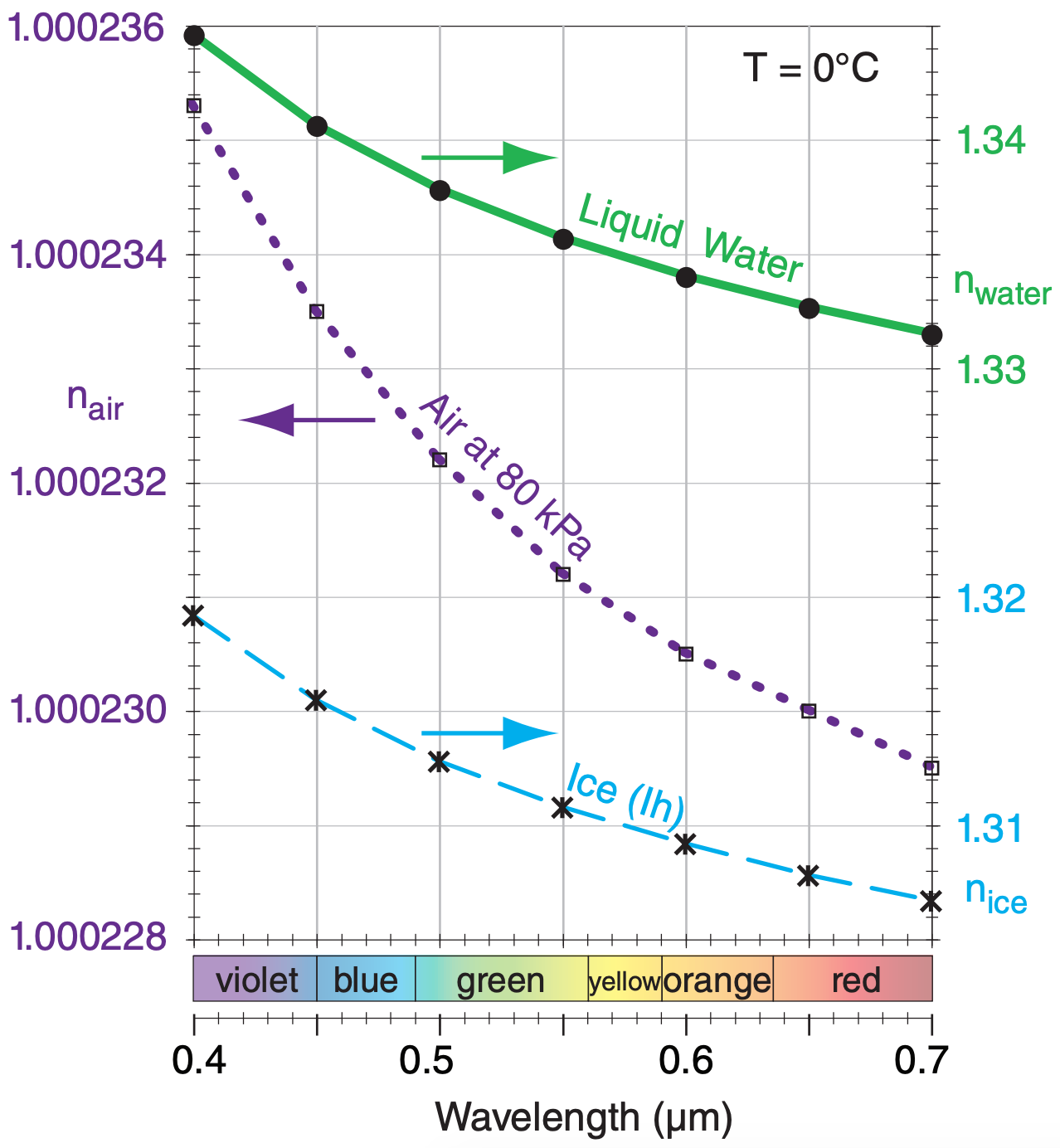
where subscripts 1 and 2 refer to the media containing the incident ray and refracted rays, respectively. Different colors and different media have different refractive indices, as indicated in Table 22-1 and Fig. 22.2.

Figure 22.2 Refractive index n for various wavelengths of visible light at T = 0°C. See Table 22-1 for details. The wavelength bands that we perceive as different colors is approximate, and is slightly different for each person. In this chapter we will use red ≈ 0.7 µm, orange ≈ 0.62 µm, yellow ≈ 0.58 µm, green ≈ 0.53 µm, blue ≈ 0.47 µm, and violet ≈ 0.4 µm wavelength.

22.1.2.2. Snell’s Law

The relationship between the incident angle θ₁ and refracted angle θ₂ (Fig. 22.1) is called Snell’s Law:

\begin{align}\dfrac{\sin \theta_{1}}{\sin \theta_{2}}=\dfrac{c_{1}}{c_{2}}=\dfrac{n_{2}}{n_{1}}=\dfrac{1}{\mu_{12}}\tag{22.4}\end{align}

Incident and refracted rays are always in the same plane. This plane includes the line that is normal to the surface. It is the plane that gives the smallest angle between the incident ray and the surface.

Solving Snell’s Law for the refracted ray angle gives:

\begin{align}\theta_{2}=\arcsin \left[\mu_{12} \cdot \sin \left(\theta_{1}\right)\right]\tag{22.5}\end{align}

Red light is bent less than violet light as it passes through an interface. Thus, refraction causes white light to be spread into a spectrum of colors. This phenomenon is called dispersion.

A viewer looking toward the incoming light ray (Fig. 22.3) would see a light point in the celestial sphere overhead at angle θ₂ from normal.

Sample Application

Rays of red and violet light in air strike a water surface, both with incident angle of 60°. Find the angle of refraction for each color, given T = 20°C, P = 101 kPa?

Find the Answer

Given: θ₁ = 60° , T = 20°C , P = 101 kPa

Find: θ₂ = ?° for red (λ=0.7 µm) and violet (λ=0.4 µm).

Use eq. (22.5): θ₂ = arcsin[(n₁/n₂)·sin(θ₁)] = arcsin[ µ₁₂ ·sin(θ₁)]

with µ₁₂ from eq. (22.3) and refractive indices from Table 22-1.

For red: µ₁₂ = n₁/n₂ = 1.0002704/1.3305 = 0.7518

θ₂ = arcsin[0.7518·sin(60°)]

θ₂ = 40.62°

Similarly for violet: θ₂ = 40.15°

Check: Units OK. Physics OK.

Exposition: Had there been no bending, then both answers would have been 60°. Angles closer to 60° for this example correspond to less bending. The answers above confirm the statement that red light is bent less than violet. The amount of bending (difference between incident and refracted angles) is large: 60° – 40° = 20°.

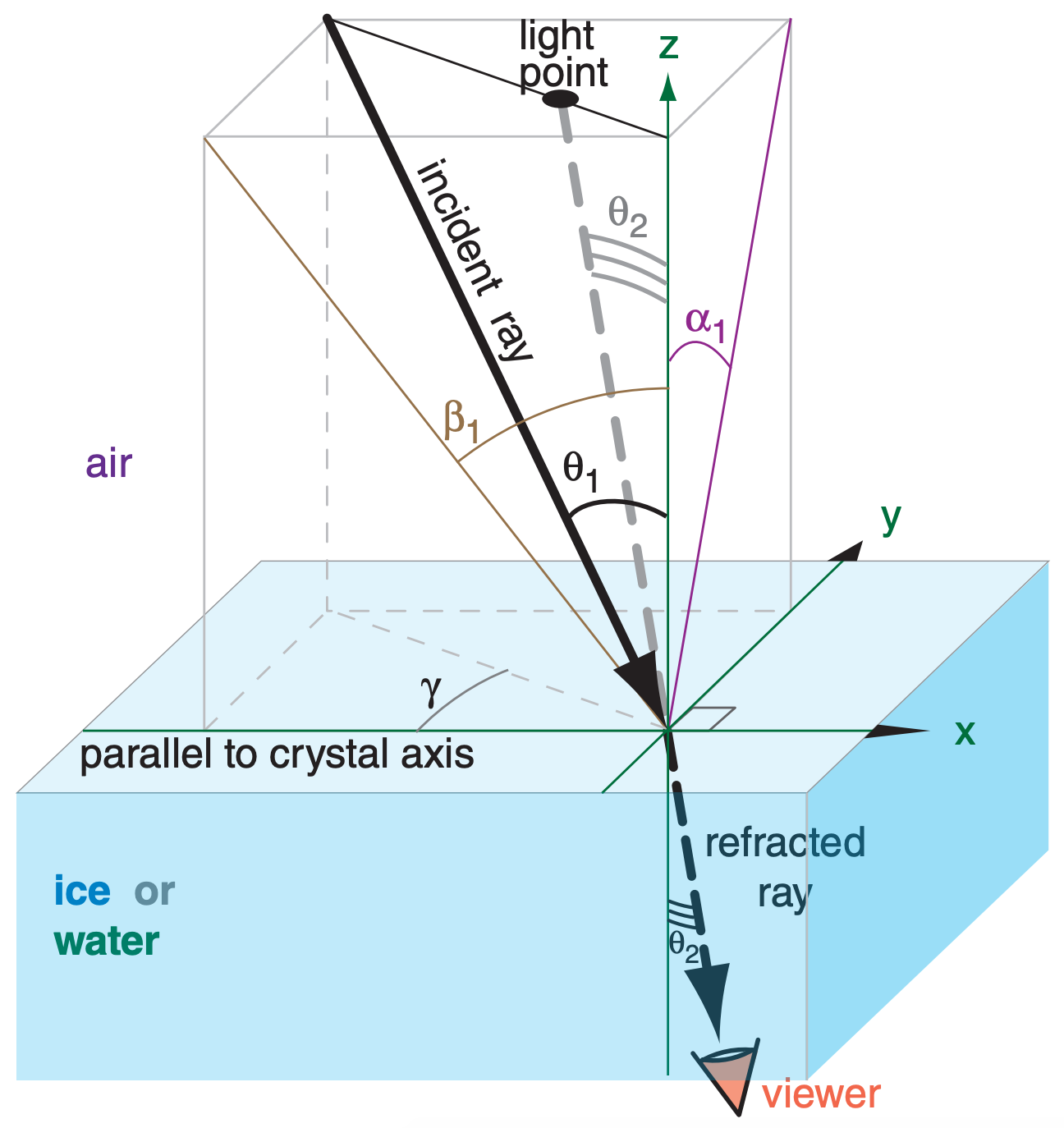
22.1.2.3. Snell’s Law in 3 Dimensions

Figure 22.3 Components of refraction geometry. Incident ray is thick black solid arrow. Refracted ray is thick black dashed arrow. The tail (thick grey dashed line) of the refracted ray is extended to show that it lies in the same plane as the incident ray.

Table 22-1a. Refractive index (nair) for air with pressure P = 101.325 kPa and relative humidity RH = 75%. From NIST.
λ (µm)	Temperature (°C)
	–40	–20	0	20	40
0.4 violet 0.45	1.0003496 1.0003469	1.0003219 1.0003194	1.0002982 1.0002958	1.0002773 1.0002751	1.0002583 1.0002562
0.5 0.55 0.6	1.0003450 1.0003436 1.0003425	1.0003176 1.0003163 1.0003154	1.0002942 1.0002930 1.0002921	1.0002736 1.0002725 1.0002716	1.0002548 1.0002537 1.0002529
0.65 0.7 red	1.0003417 1.0003410	1.0003146 1.0003140	1.0002914 1.0002908	1.0002710 1.0002704	1.0002523 1.0002523

Table 22-1b. Refractive index (nair) for air with P = 80 kPa and RH = 75%. Based on data from NIST modified Edlen Eq. calculator.
λ (µm)	Temperature (°C)
	–40	–20	0	20	40
0.4 violet 0.45	1.0002760 1.0002738	1.0002541 1.0002521	1.0002353 1.0002335	1.0002188 1.0002171	1.0002036 1.0002019
0.5 0.55 0.6	1.0002723 1.0002712 1.0002704	1.0002507 1.0002497 1.0002489	1.0002322 1.0002312 1.0002305	1.0002158 1.0002150 1.0002143	1.0002008 1.0001999 1.0001993
0.65 0.7 red	1.0002697 1.0002692	1.0002483 1.0002479	1.0002300 1.0002295	1.0002138 1.0002134	1.0001988 1.0001984

Table 22-1c. Refractive index (nair) for air with P = 40 kPa and RH = 75%. Based on data from NIST modified Edlen Eq. calculator.
λ (µm)	Temperature (°C)
	–40	–20	0	20	40
0.4 violet 0.45	1.0001379 1.0001369	1.0001270 1.0001260	1.0001176 1.0001166	1.0001091 1.0001082	1.0001009 1.0001000
0.5 0.55 0.6	1.0001361 1.0001355 1.0001351	1.0001253 1.0001248 1.0001244	1.0001160 1.0001155 1.0001151	1.0001076 1.0001071 1.0001068	1.0000994 1.0000990 1.0000987
0.65 0.7 red	1.0001348 1.0001346	1.0001241 1.0001239	1.0001149 1.0001147	1.0001066 1.0001064	1.0000985 1.0000983

Table 22-1d. Refractive index (nwater) for liquid water. Based on data from IAPWS 1997 release and CRC Handbook.
λ (µm)	Temperature (°C)
	–40	–20	0	20	40
0.4 violet 0.45	(values below 0°C are for supercooled water)	1.3429 1.3390	1.3446 1.3406	1.3436 1.3396	1.3411 1.3371
0.5 0.55 0.6		1.3362 1.3341 1.3324	1.3378 1.3357 1.3340	1.3368 1.3347 1.3330	1.3344 1.3323 1.3306
0.65 0.7 red		1.3310 1.3299	1.3326 1.3315	1.3317 1.3305	1.3293 1.3281
density (kg m^–3):		993.547	999.840	998.207	992.200

Table 22-1e. Refractive index (nice) for ice (Ih). Based on data from S.G. Warren (1984) and V.F. Petrenko & R.W. Whitworth (1999).
λ (µm)	Temperature (°C)
	–40	–20	0	20	40
0.4 violet 0.45	1.3206 1.3169	1.3199 1.3162	1.3192 1.3155	(values at 0°C were extrapolated) (See INFO Box in Precipitation chapter for info on ice phases such as Ih.)
0.5 0.55 0.6	1.3142 1.3122 1.3106	1.3135 1.3115 1.3099	1.3128 1.3108 1.3092
0.65 0.7 red	1.3092 1.3081	1.3085 1.3074	1.3078 1.3067

Sometimes it is easier to work with x and y components of the incident ray, where the x-axis might be aligned with the axis of a columnar ice crystal, for example, and the y-axis might be on the crystal prism surface (Fig. 22.3). The relationship between the component angles and the incident angle is:

\begin{align}\tan ^{2} \theta_{1}=\tan ^{2} \alpha_{1}+\tan ^{2} \beta_{1}\tag{22.6}\end{align}

where θ, α and β are as illustrated in Fig. 22.3. This relationship also applies to refracted angles (θ₂, α₂, β₂), and will be used extensively later in this chapter to discuss ice-crystal optics.

Sample Application

Find the speed of red light through liquid water and through air at T = 20°C and P = 101.325 kPa.

Find the Answer

Given: λ = 0.7 µm, T = 20°C and P = 101.325 kPa.

Find: c_air = ? m s^–1 , c_water = ? m s^–1.

Use eq. (22.2) with refractive indices from Table 22-1:

c_air = c_o/n_air =(3x10⁸m s^–1)/1.0002704 =2.999x10⁸ m s^–1

c_water = c_o/n_water =(3x10⁸m s^–1)/1.3305 =2.255x10⁸ m s^–1

Check: Speeds reasonable. Units OK.

Exposition: The difference in speeds of light is useful for understanding Huygens’ principle.

Component angles α and β of the refracted and incident rays do NOT individually obey Snell’s law (eq. 22.4). Nevertheless, Snell’s law can be reformulated in terms of components as follows:

\begin{align}\tan ^{2} \alpha_{2}=b_{\alpha \beta} \cdot \tan ^{2} \alpha_{1}\tag{22.7a}\end{align}

\begin{align}\tan ^{2} \beta_{2}=b_{\alpha \beta} \cdot \tan ^{2} \beta_{1}\tag{22.7b}\end{align}

where α₂ and β₂ are the components of the refracted ray (analogous to α₁ and β₁), and

\begin{align}b_{\alpha \beta}=\dfrac{\mu_{12}^{2}}{1+\left(1-\mu_{12}^{2}\right) \cdot\left\{\tan ^{2} \alpha_{1}+\tan ^{2} \beta_{1}\right\}}\tag{22.8}\end{align}

These equations are abbreviated as

\begin{align}\alpha_{2}=S_{\alpha}\left(\alpha_{1}, \beta_{1,}, \mu_{12}\right)=\arctan \left[\left(b_{\alpha \beta} \cdot \tan ^{2} \alpha_{1}\right)^{1 / 2}\right]\tag{22.9b}\end{align}

\begin{align}\beta_{2}=S_{\beta}\left(\alpha_{1}, \beta_{1,}, \mu_{12}\right)=\arctan \left[\left(b_{\alpha \beta} \cdot \tan ^{2} \beta_{1}\right)^{1 / 2}\right]\tag{22.9b}\end{align}

where S represents Snell’s law for components.

Sample Application

A ray of red light in air strikes a water surface, with incidence angle components of α₁ = 45° and β₁ = 54.74°. What are the corresponding component angles of the refracted ray? Assume T = 20°C and P = 101.325 kPa.

Find the Answer

Given: α₁ = 45°, β₁ = 54.74°.

Find: α₂ = ?° , β₂ = ?°

From Table 22-1 for red light:

µ₁₂² = (1.0002704/1.3305)² = (0.7518)² = 0.5652

Also: tan(45°) = 1, and tan(54.74°) = 1.414

Next, solve eq. (22.8):

\(\ b_{\alpha \beta}=\dfrac{0.5652}{1+(1-0.5652) \cdot(1+2)}=0.2453\)

Then use eqs. (22.7):

tan²(α₂) = 0.2453·[tan²(45°)] = 0.2453

tan²(β₂) = 0.2453·[tan²(54.74°)] = 0.4906

Thus: α₂ = arctan[(0.2453)^0.5] = 26.35°

β₂ = arctan[(0.4906)^0.5] = 35.01°

Check: Units OK. Physics OK.

Exposition: Using eq. (22.6) with the incident angle components of 45° and 54.74°, we find that the incident ray angle is θ₁=arctan[(1+2)^0.5] = 60°. This is the same as a previous Sample Application. Using eq. (22.6) on the answers above, we find θ₂ = arctan[ (0.2453+0.4906)^0.5] = 40.62°. This is also the same as a previous Sample Application, which verifies eqs. (22.6 - 22.9).

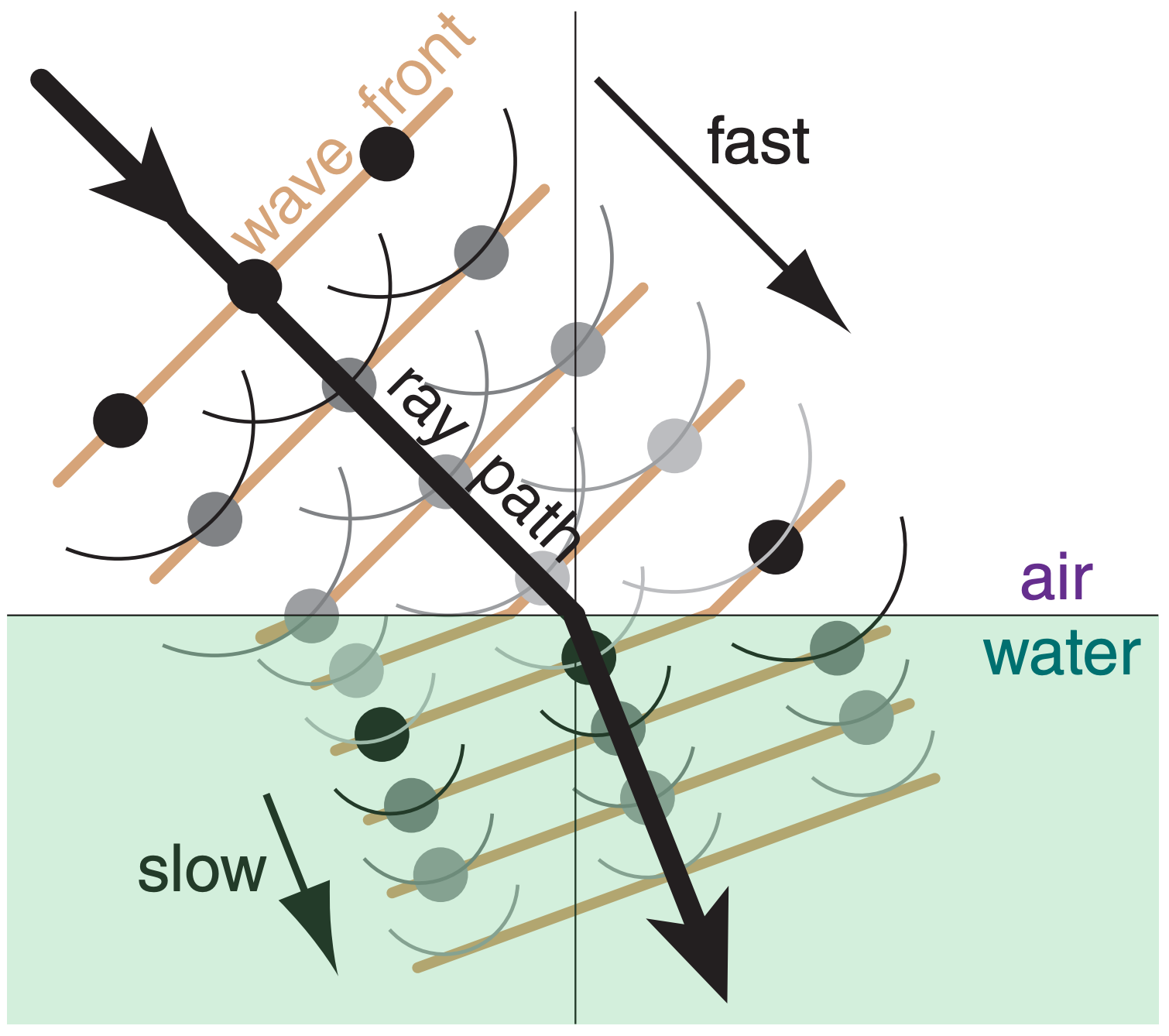
22.1.3. Huygens’ Principle

Huygens suggested that every point along a wave front acts like a generator of new spherical secondary wavelets. Wave-front position after some time interval is located at the tangent to all of the new wavelets. Thus, when a portion of a wave front encounters a medium with a slower light velocity, then that portion of the wave slows, causing the whole wave front to turn into the medium (Fig. 22.4).

Figure 22.4 Propagation of wave fronts (tan color) across an air-water interface. The wave fronts are analogous to ocean wave crests, and the thick black arrow shows the direction that the waves are moving. This movement direction is called the ray path.

22.1.4. Critical Angle

When Snell’s law is applied to light rays moving from a denser medium (having slower light velocity) to a less-dense medium (having faster light velocity), there is a critical angle at which light is bent so much that it follows the interface. At angles greater than this critical incidence angle, light cannot refract out of the dense medium at all. Instead, all of the light reflects (Fig. 22.5).

Figure 22.5 When light travels from a dense toward a less-dense medium (e.g., from water to air), angles greater than critical θ_c cannot escape from the denser medium.

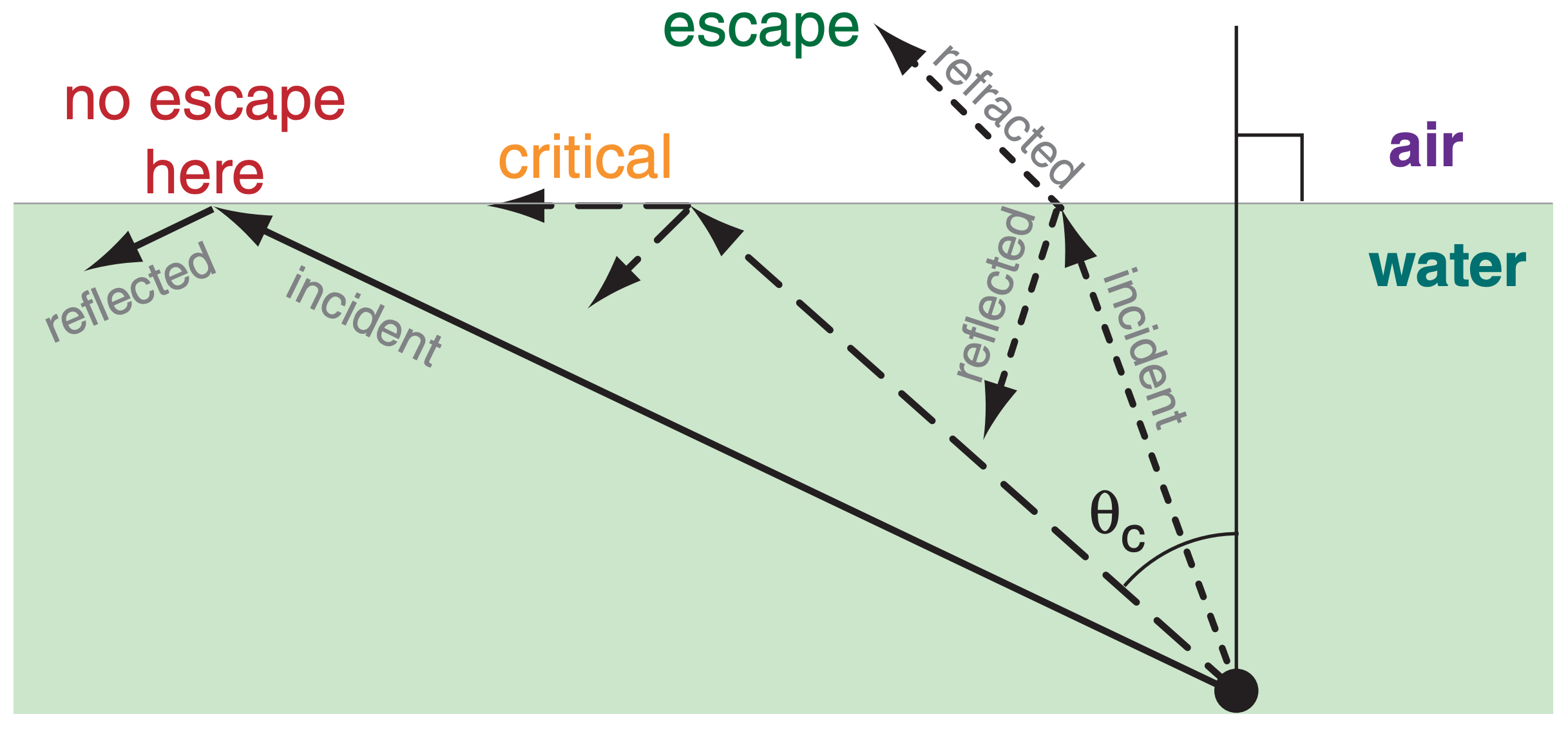
The critical angle θ_c is found from:

\begin{align}\sin \left(\theta_{c}\right)=\dfrac{n_{2}}{n_{1}}\tag{22.10}\end{align}

where n₁ is the refractive index for the incident ray (i.e., in the denser medium with slower light velocity). An example is a light ray moving out of water and into air (i.e., n₂ < n₁). For red light the critical angle is about 48.7°, while for violet light the it is about 48.1°. There is no critical angle for light moving into a denser medium (i.e., n₂ > n₁).

Sample Application

Find the critical angle for red light going from a cirrus-cloud ice crystal to air, where the air state is T = –20°C & P = 40 kPa.

Find the Answer

Given: red light λ = 0.7 µm, T = –20°C & P = 40 kPa.

Find: θ_c = ? °

From Table 22-1: n_ice = 1.3074 and n_air = 1.0001239.

Use eq. (22.10):

θ_c = arcsin(1.0001239/1.3074) = 49.9°.

Check: Magnitude OK. Units OK.

Exposition: This critical angle is close to that for liquid water, because the refractive indices for liquid and solid water are nearly the same.