14.5: Wind Shear in the Environment
- Page ID
- 9621
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Wind shear, the change of horizontal wind speed and/or direction with height, is the third requirement for thunderstorm formation. The wind shear across a layer of air is the vector difference between the winds at the top of the layer and winds at the bottom (Fig. 14.46), divided by layer thickness ∆z. Shear has units of (s–1).
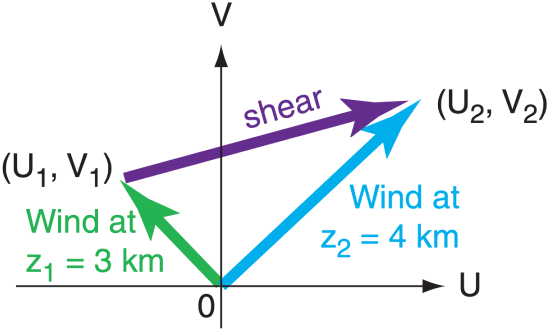
Using geometry, shear can be expressed via its components as:
\(\ \begin{align} \frac{\Delta U}{\Delta z}=\frac{U_{2}-U_{1}}{z_{2}-z_{1}}\tag{14.9}\end{align}\)
\(\ \begin{align} \frac{\Delta V}{\Delta z}=\frac{V_{2}-V_{1}}{z_{2}-z_{1}}\tag{14.10}\end{align}\)
or
\(\ \begin{align} \text{Shear Magnitude} =\frac{\left[(\Delta U)^{2}+(\Delta V)^{2}\right]^{1 / 2}}{\Delta z}\tag{14.11a}\end{align}\)
Shear Direction: \(\ \begin{align} \alpha_{\text {shear}}=90^{\circ}-\frac{360^{\circ}}{C} \arctan \left(\frac{\Delta V}{\Delta U}\right)+\alpha_{o}\tag{14.11b}\end{align}\)
where subscript 2 is the layer top, and subscript 1 is layer bottom. Eq. (1.2) was used to find the direction α of the shear vector, but with ∆U in place of U, and ∆V in place of V. αo = 180° if ∆U > 0, but is zero otherwise. C is the angular rotation in a circle (360° or 2π radians, depending on how your calculator or spreadsheet calculates the “arctan” function).
For thunderstorm forecasting, meteorologists look at shear across many layers at different heights in the atmosphere. To make this easier, you can use layers of equal thickness, such as ∆z = 1 km. Namely, look at the shear across the bottom layer from z = 0 to z = 1 km, and the shear across the next layer from z = 1 to z = 2 km, and so forth.
When studying shear across layers of equal thickness, meteorologists often use the vector wind difference (∆U, ∆V) as a surrogate measure of vector wind shear. We will use this surrogate here. This surrogate, and its corresponding wind-difference magnitude
\(\ \begin{align} \Delta M=\left(\Delta U^{2}+\Delta V^{2}\right)^{1 / 2}\tag{14.12}\end{align}\)
have units of wind speed (m s–1).
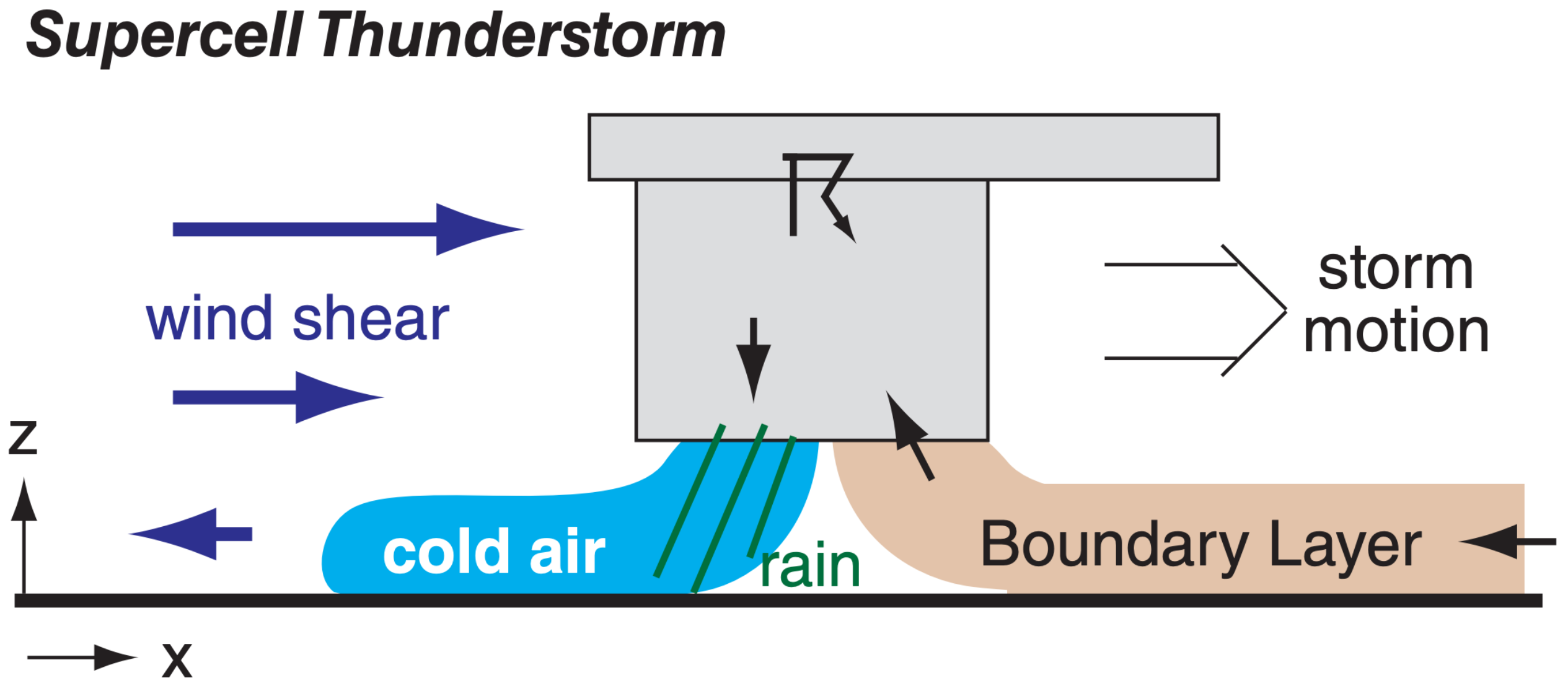
Thunderstorms can become intense and longlasting given favorable wind shear in the lower atmosphere. Under such conditions, humid boundary-layer air (thunderstorm fuel) can be fed into the moving storm (Fig. 14.47). Another way to picture this process is that wind shear causes the storm to move away from locations of depleted boundary layer fuel into locations with warm, humid boundary layers. The storm behaves similar to an upright vacuum cleaner, sucking up fresh boundary-layer air as it moves, and leaving behind an exhaust of colder air that is more stable.
Sample Application
Given a horizontal wind of (U, V) = (1, 2) m s–1 at 2 km altitude, and a wind of (5, –3) m s–1 at 3 km, what are the wind-difference & shear magnitudes & direction?
Find the Answer
Given: (U, V) = (1, 2) m s–1 at z = 2 km, (U, V) = (5, –3) m s–1 at z = 3 km
Find: ∆M = ? (m s–1), α = ? (°), Shear Magnitude = ? (s–1),
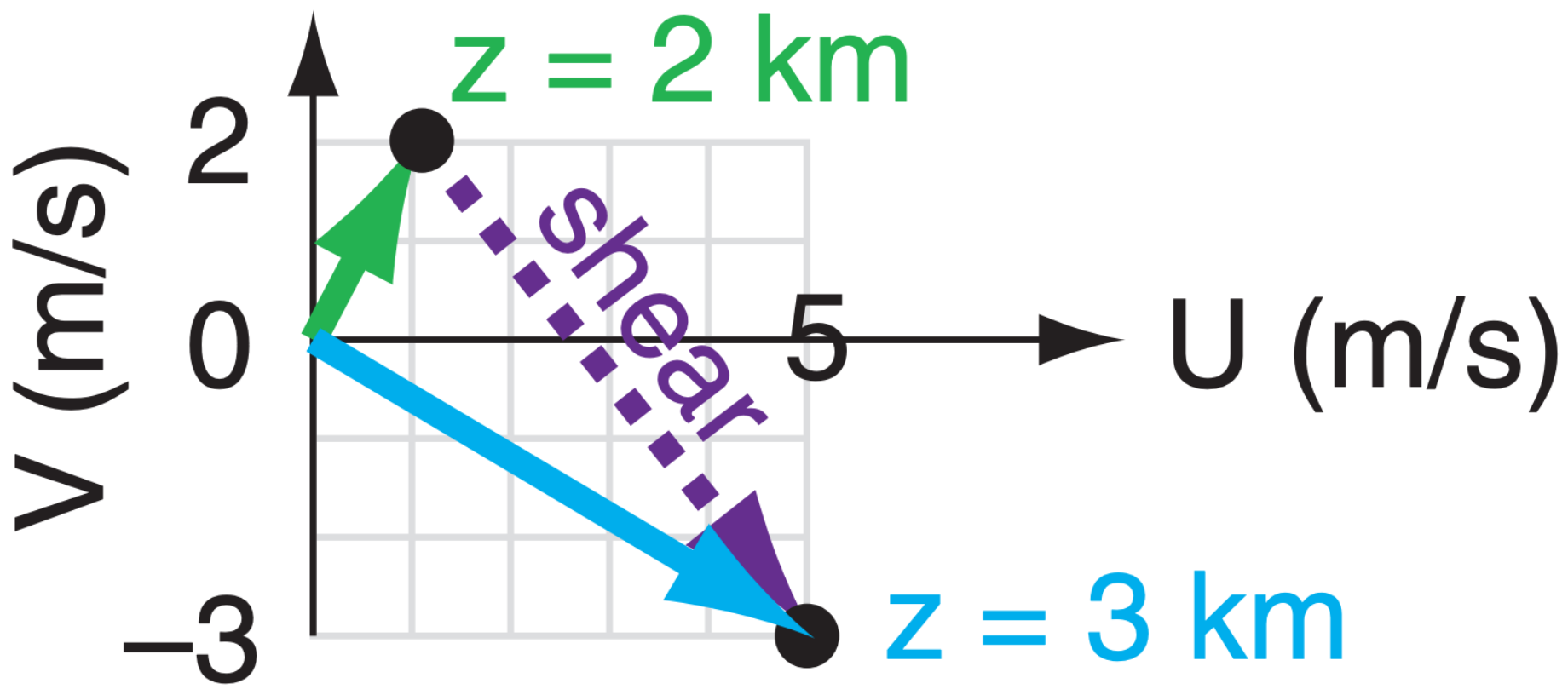
Use eq. (14.12) to find the wind difference magnitude:
∆U = (5 – 1) = 4 m s–1. ∆V = (–3 – 2) = –5 m s–1.
∆M = [ (4 m s–1)2 + (–5 m s–1)2 ]1/2 = [16+25 m2 s–2] 1/2 = 6.4 m s–1
Use eq. (14.11b) to find shear direction:
α = 90°–arctan(–5/4)+180° = 90°–(–51.3°)+180° = 321.3° which is the direction that the wind shear is coming from.
Layer thickness is ∆z = 3 km – 2 km = 1 km = 1000 m.
Use eq. (14.11a) to find shear magnitude, rewritten as: Shear Mag.= ∆M/∆z = (6.4 m s–1)/(1000 m) = 0.0064 s–1.
Check: Units OK. Magnitude & direction agree with figure.
Exposition: There is both speed shear and directional shear in this example. It always helps to draw a figure, to check your answer.
In an environment with wind but no wind shear, the thunderstorms would last only about 15 minutes to 1 hour, because the thunderstorm and boundary-layer air would move together. The storm dies after it depletes the fuel in its accompanying boundary layer (Fig. 14.6).
Shear can also control the direction that a thunderstorm moves. As will be shown later, a clockwise turning wind shear vector can create a dynamic vertical pressure gradient that favors thunderstorms that move to the right of the mean steering-level wind direction.
Mesocyclones can also be created by wind shear. A mesocyclone is where the whole thunderstorm rotates. A mesocyclone can be a precursor to strong tornadoes, and is discussed in detail in the next chapter. Strong shear also enhances MCSs such as bow-echo thunderstorms. Thus, there are many ways that upper-air wind (winds above the surface) and wind shear affect thunderstorms.
14.5.1. Hodograph Basics
One way to plot upper-air wind vs. height is to draw wind symbols along the side of a thermo diagram (Fig. 14.48). Recall from the Atmospheric Forces and Winds chapter that wind direction is from the tail end (with the feathers) toward the other end of the shaft, and more barbs (feathers or pennants) indicate greater speed. The tip of the arrow is at the altitude of that wind.
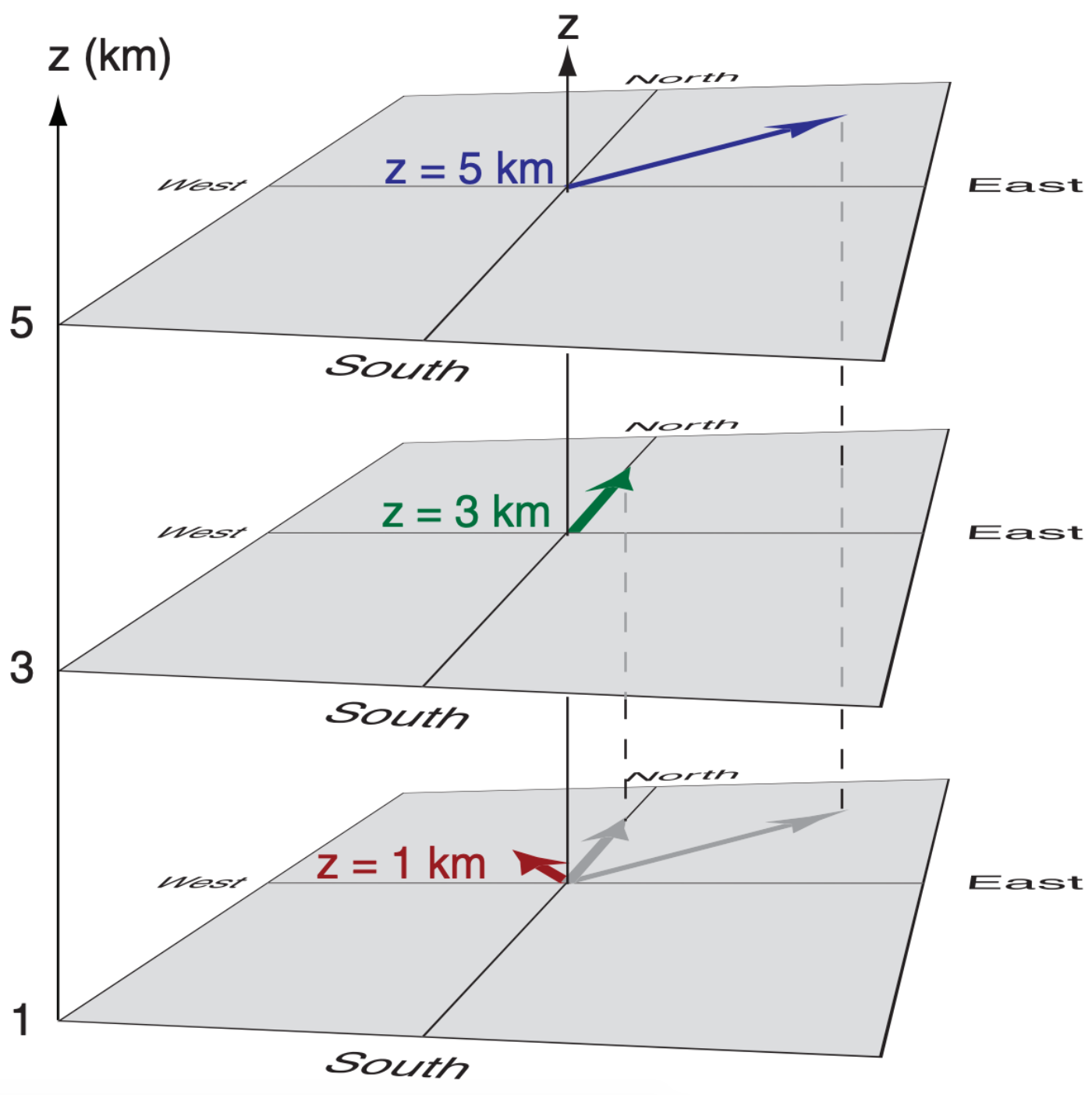
Another way to plot winds is to collapse all the wind vectors from different heights into a single polar graph, which is called a hodograph. For example, Fig. 14.49 shows winds at 1 km altitude blowing from the southeast at 15 m s–1, winds at 3 km altitude blowing from the south at 25 m s–1, and winds at 5 km altitude blowing from the southwest at 35 m s–1. Both directional shear and speed shear exist.
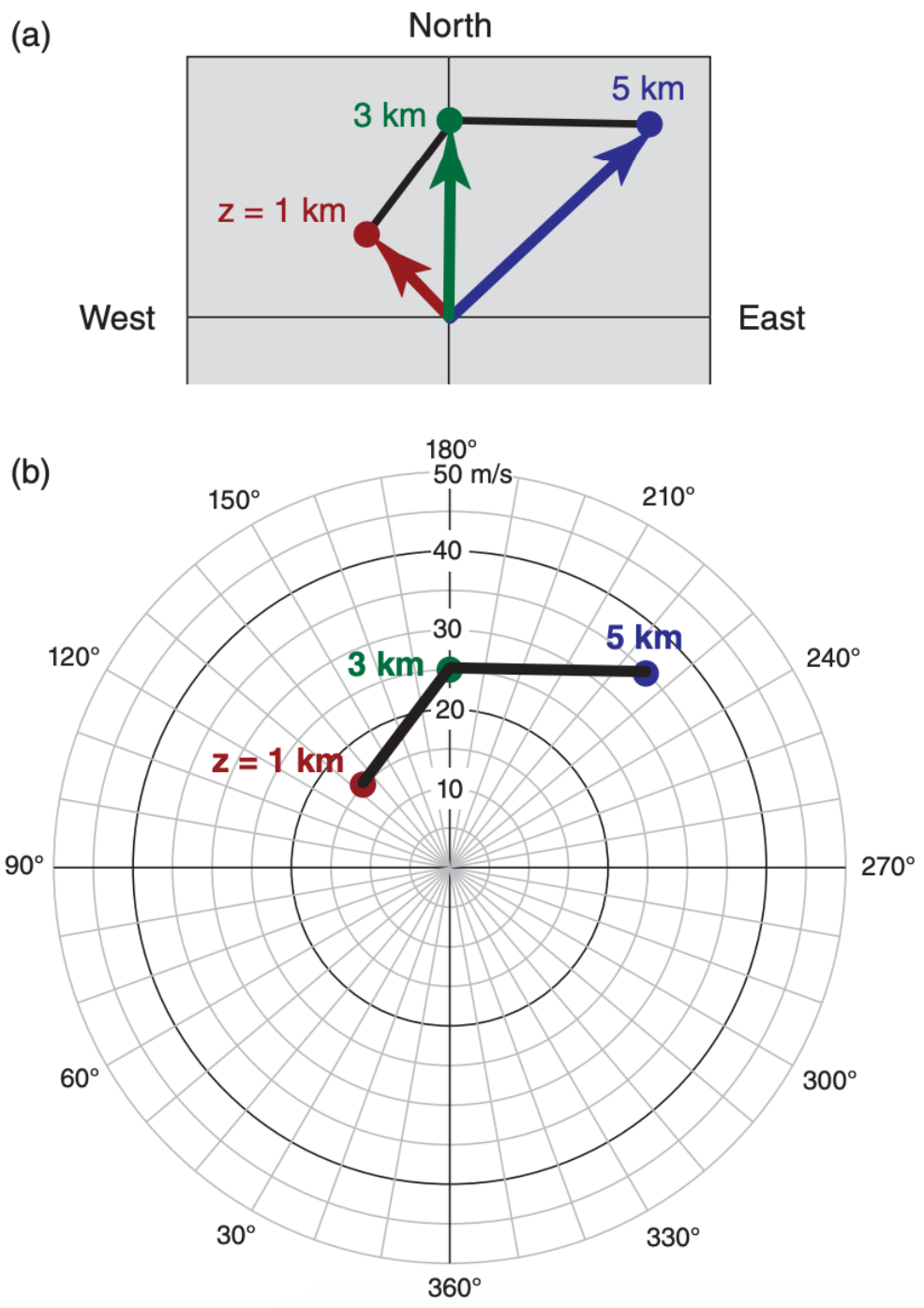
If you project those wind vectors onto a single plane, you will get a graph that looks like the bottom of Fig. 14.49. Next, draw a dot at the end of each projected vector (Fig. 14.50a), and connect the dots with straight lines, going sequentially from the lowest to the highest altitude. Label the altitudes above ground level (AGL) next to each dot.
Plot the resulting dots and connecting lines on polar graph paper, but omit the vectors (because they are implied by the positions of the dots). The result is called a hodograph (Fig. 14.50b). Fig. 14.51 is a large blank hodograph that you can copy and use for plotting your own wind profiles. Coordinates in this graph are given by radial lines for wind direction, and circles are wind speed.
Sample Application
Use the table of winds below to plot a hodograph.
| z (km) | dir. (°) | M (m s–1) | z (km) | dir. (°) | M (m s–1) |
|---|---|---|---|---|---|
| 0 | 0 | 0 | 4 | 190 | 15 |
| 1 | 130 | 5 | 5 | 220 | 20 |
| 2 | 150 | 10 | 6 | 240 | 30 |
| 3 | 170 | 13 |
Find the Answer
Plot these points on a copy of Fig. 14.51, and connect with straight lines. Label each point with its altitude.
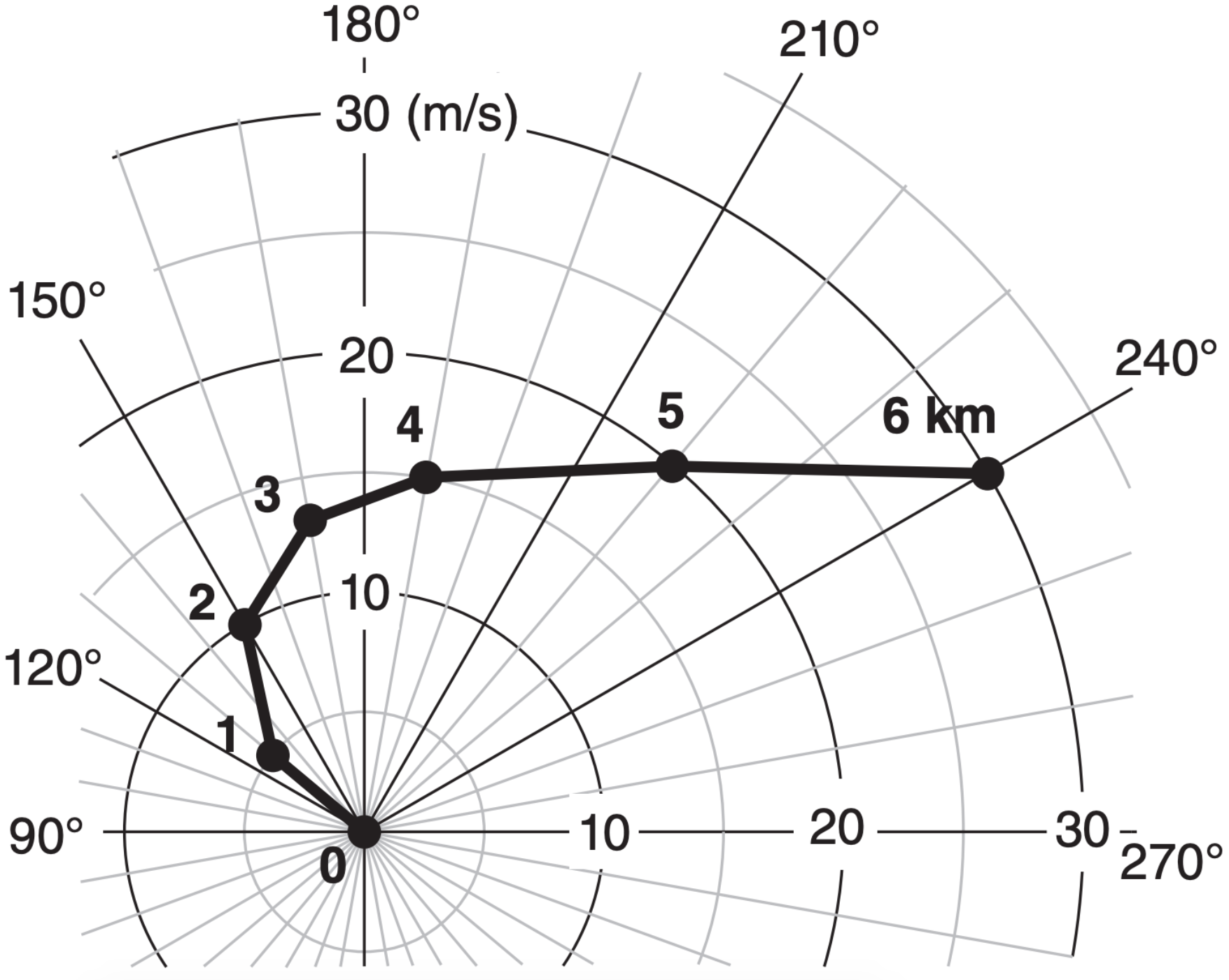
Check: The wind at 6 km, for example, is from the west southwest (240°), implying that the tip of the wind vector would be pointing toward the east northeast. This agrees with the location of the 6 km dot.
Exposition: This profile shows both speed and directional shear. Also, these winds veer (turn clockwise) with increasing altitude. From the thermal wind Exposition in Chapter 11, recall that veering winds are associated with warm-air advection, which is useful for bringing warmer air into a thunderstorm.
From “Practical Meteorology” Copyright © 2017 by Roland Stull Free copies of this page permitted.

Notice that the compass angles labeled on the blank hodograph appear to be backwards. This is not a mistake. The reason is that winds are specified by where they come from. For example, the wind at 1 km altitude is from the southeast; namely, from a compass direction of 135° (Fig. 14.50). The south wind at 3 km is from 180°, and the southwest wind at 5 km is from 235°. Using the “from” direction and the wind speed to specify points, the result is a hodograph that implies wind vectors pointing from the origin to the correct directions in physical space. As another example, a wind from the west (direction 270° on the hodograph) gives a vector pointing from the origin toward the right (which is toward the east in physical space). We define physical space in this context as having north at the top of the page, east at the right, south at the bottom, and west at the left.
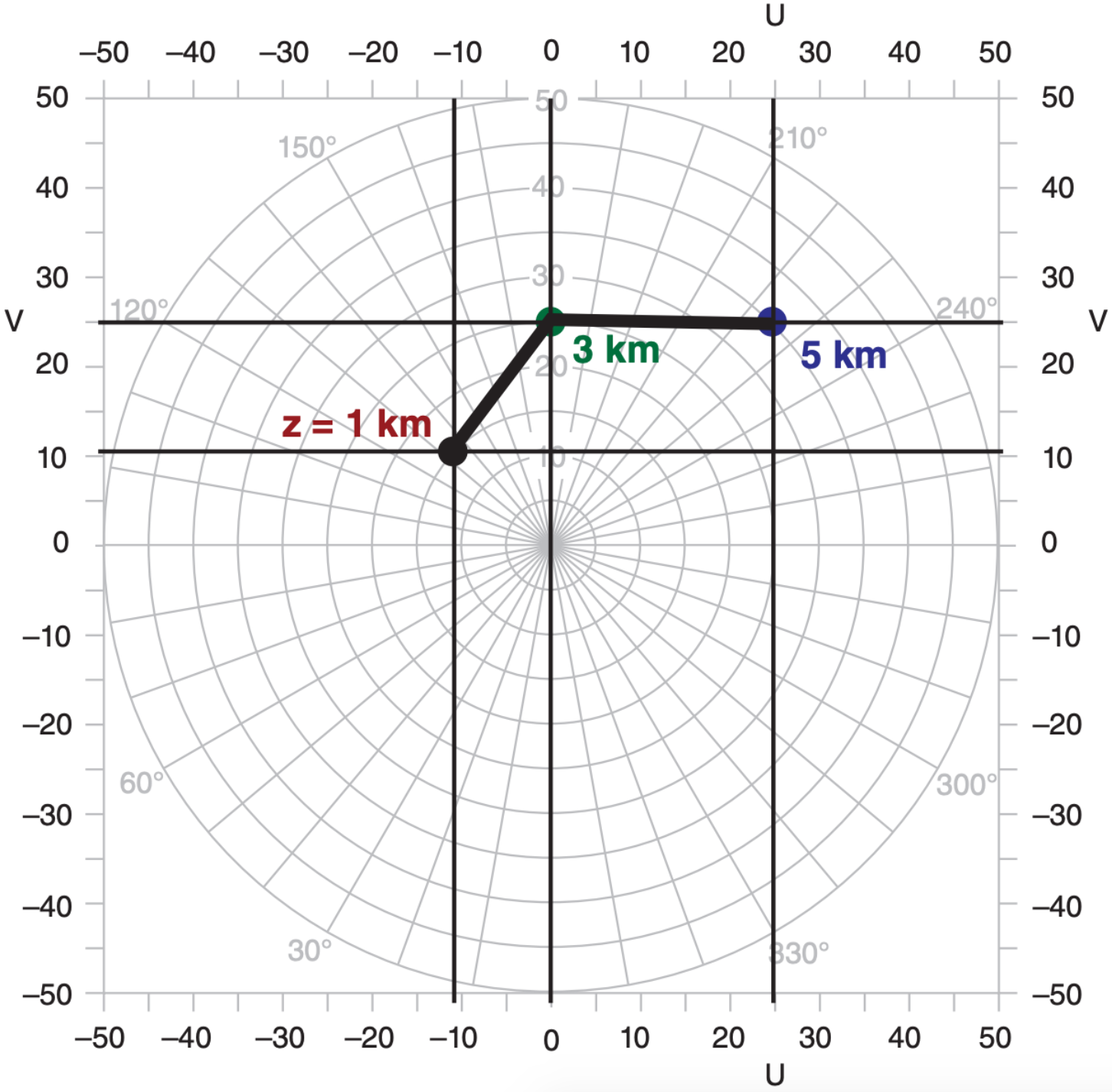
Often you need to use the U and V wind components to determine thunderstorm characteristics. You could calculate these using eqs. (1.3) and (1.4) from Chapter 1. Alternately, you can pick them off from the coordinates of the hodograph. As demonstrated in Fig. 14.52, for any hodograph point, use a straight-edge to draw a horizontal line from that point to the ordinate to determine V, and draw a vertical line from the point to the abscissa to get U.
Sample Application
For the hodograph of Fig. 14.52, find the (U, V) Cartesian coordinate for each of the 3 data points.
Find the Answer
Given: Hodograph Fig. 14.52 with data points at z = 1, 3, and 5 km.
Find: (U, V) = ? (m s–1) for each point.
Hint: The hodograph has the U velocities labeled at both top and bottom to make it easier to draw perfectly vertical lines through any data point. For the same reason, V velocities are labeled at both left and right.
Method: Draw horizontal and vertical lines through each data point, and then pick off the U and V values by eye. Results:
For z = 1 km: U = –11 m s–1, V = 11 m s–1.
For z = 3 km: U = 0 m s–1, V = 25 m s–1.
For z = 5 km: U = 25 m s–1, V = 25 m s–1.
Check: Signs and magnitudes are consistent with directions and speeds.
Exposition: Be careful to use the proper units. The legend in the Fig. 14.52 caption tells us the units for wind speed.
CAUTION: Different agencies use different units (m s–1, knots, miles·h–1, km·h–1) and sometimes forget to state the units in the hodograph legend or caption.
14.5.2. Using Hodographs
Wind and wind-shear between the surface and 6 km altitude affects the dynamics, evolution, and motion of many N. American thunderstorms. You can use a hodograph to determine key wind-related quantities, including the:
- local shear across a single layer of air
- mean wind-shear vector
- total shear magnitude
- mean wind vector (normal storm motion)
- right & left moving supercell motions
- storm-relative winds.
14.5.2.1. Shear Across a Single Layer
Local shear between winds at adjacent wind-reporting altitudes is easy to find using a hodograph. Comparing Figs. 14.46 with 14.50, you can see that the hodograph line segment between any two adjacent altitudes is equal to the local shear across that one layer. For example, the solid grey arrow in Fig. 14.53 (one of the line segments of the hodograph) shows the local shear across the 4 to 5 km layer.
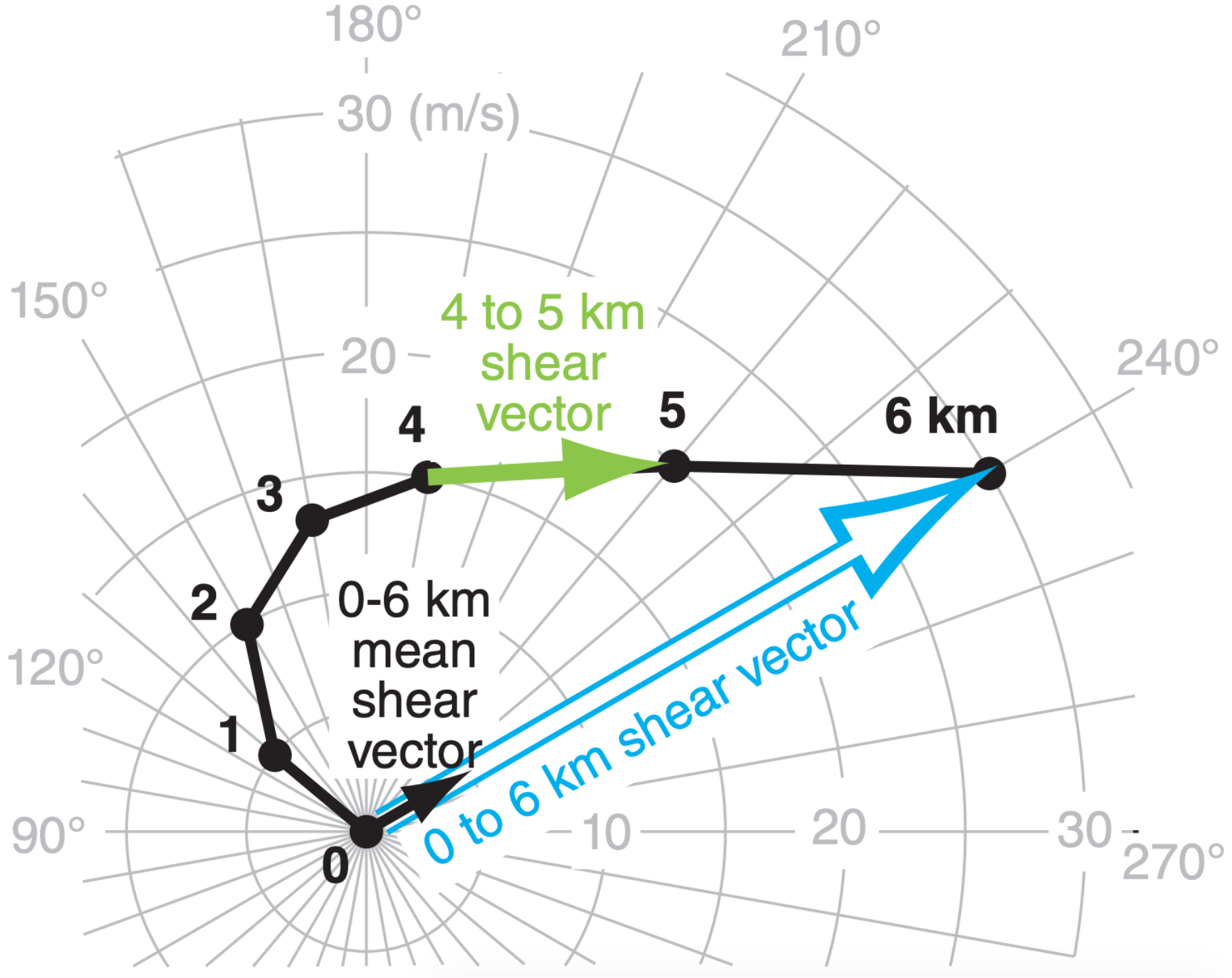
14.5.2.2. Mean Wind Shear Vector
The mean wind-shear vector across multiple layers of air of equal thickness is the vector sum of the local shear vectors (i.e., all the hodograph line segments for those layers), divided by the number of layers spanned. Namely, it is a vector drawn from the bottom-altitude wind point to the top-altitude wind point (white arrow with grey border in Fig. 14.53), then divided by the number of layers to give the mean (solid black arrow). For Fig. 14.53, the mean 0 - 6 km shear vector is 5 m s–1 from the west southwest. This graphical method is easy to use.
To find the same mean wind-shear vector mathematically, use the U and V components for the wind at z = 0, and also for the wind at z = 6 km. The mean shear (subscript ms) vector coordinates (∆U, ∆V)ms for the 0 to 6 km layer is given by:
\(\ \begin{align} \Delta U_{m s}=\left(U_{6 k m}-U_{0 k m}\right) / 6\tag{14.13}\end{align}\)
\(\ \begin{align} \Delta V_{m s}=\left(V_{6 k m}-V_{0 k m}\right) / 6\tag{14.14}\end{align}\)
You can use these coordinates to plot the mean shear vector on a hodograph. You can use eqs. (14.15) and (14.16) to determine the magnitude and direction, respectively, of the mean shear vector. Namely:
\(\ \begin{align} \Delta M_{m s}=\left(\Delta U_{m s}^{2}+\Delta V_{m s}^{2}\right)^{1 / 2}\tag{14.15}\end{align}\)
\(\ \begin{align} \alpha_{m s}=90^{\circ}-\frac{360^{\circ}}{\mathrm{C}} \cdot \arctan \left(\Delta V_{m s} / \Delta U_{m s}\right)+\alpha_{o}\tag{14.16}\end{align}\)
where C = 360° or 2π radians (depending on the units returned by your calculator or spreadsheet), and αo = 180° if ∆Ums > 0, but is zero otherwise. As before, these wind-difference values are surrogates for the true shear.
Sample Application
Using data from the previous Sample Application, mathematically get the mean shear vector coordinates (∆U, ∆V)ms, magnitude (∆M ms), and direction (α ms).
Find the Answer
Given: Wind vectors at 6 and 0 km altitude:
M = 30 m s–1 and α = 240° at z = 6 km, M = 0 m s–1 and α = 0° at z = 0 km.
Let: subscript “ms” = “mean shear”
Find: (∆U, ∆V)ms = ? (m s–1), ∆Mms = ? (m s–1), αms = ? (°)
First, find (U, V) components from eqs. (1.3) & (1.4):
U6km = –M·sin(α) = –(30m s–1)·sin(240°) = 26 m s–1.
V6km = –M·cos(α) = –(30m s–1)·cos(240°) = 15 m s–1.
and
U0km = –M·sin(α) = –(0m s–1)·sin(0°) = 0 m s–1.
V0km = –M·cos(α) = –(0m s–1)·cos(0°) = 0 m s–1.
Next, use eqs. (14.13 & 14.14):
∆Ums = (26 – 0 m s–1)/6 = 4.3 m s–1.
∆Vms = (15 – 0 m s–1)/6 = 2.5 m s–1.
Then, use (∆U, ∆V) in eq. (14.15):
∆Mms = [(∆Ums)2 + (∆Vms)2] 1/2
= [(4.3 m s–1)2 + (2.5 m s–1)2] 1/2
= [24.74 (m s–1)2] 1/2 = 5 m s–1
Finally, use (∆U, ∆V) in eq. (14.16):
αms = 90° – arctan(∆Vms/∆Ums) + 180°
= 90° – arctan(2.5/4.3) + 180°
= 90° – 30° + 180° = 240°
Check: Units OK. Agrees with Fig. 14.53 black arrow.
Exposition: The only reason the mean shear direction equaled the wind direction at 6 km was because the surface wind was zero. Normally they differ.
14.5.2.3. Total Shear Magnitude
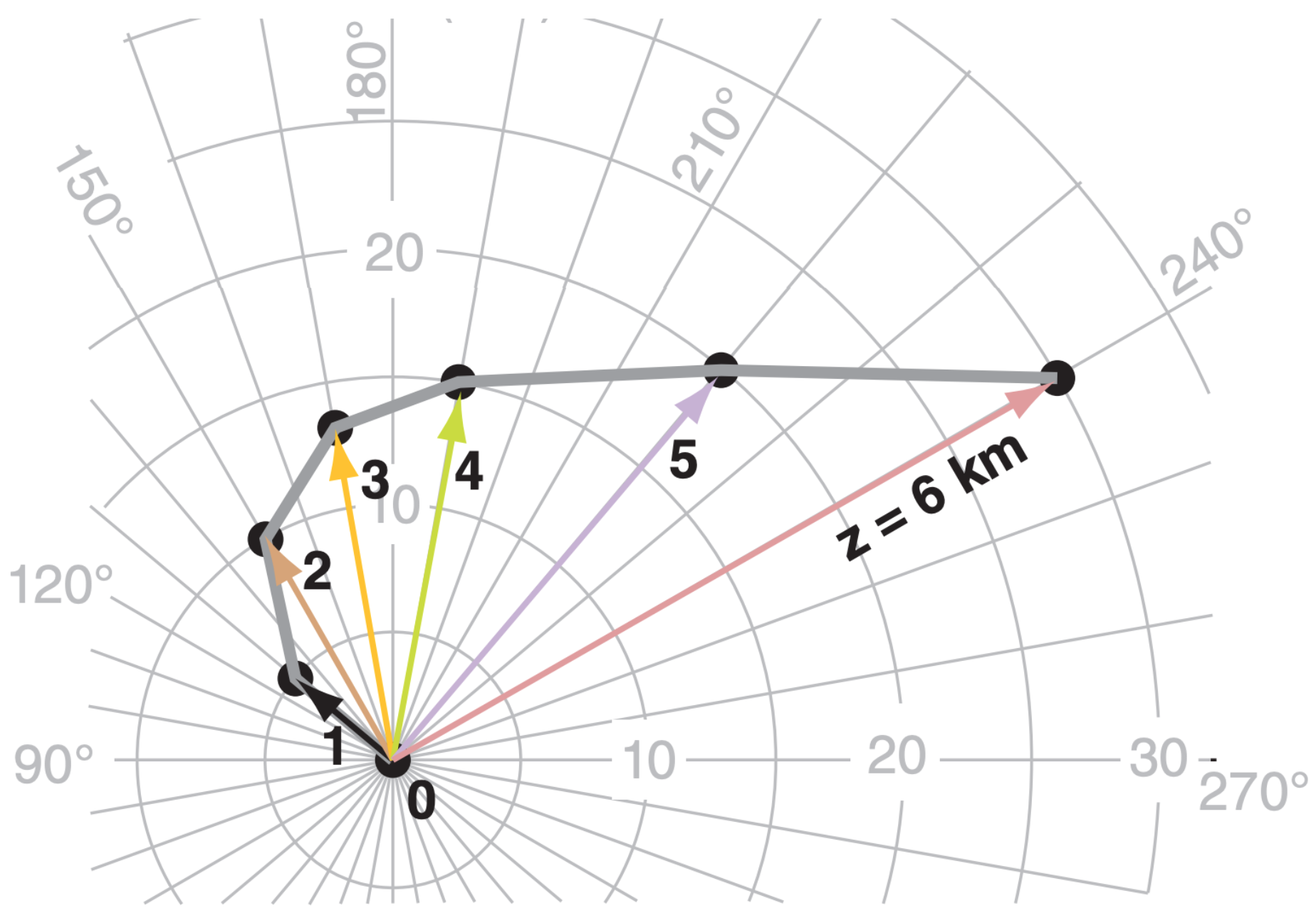
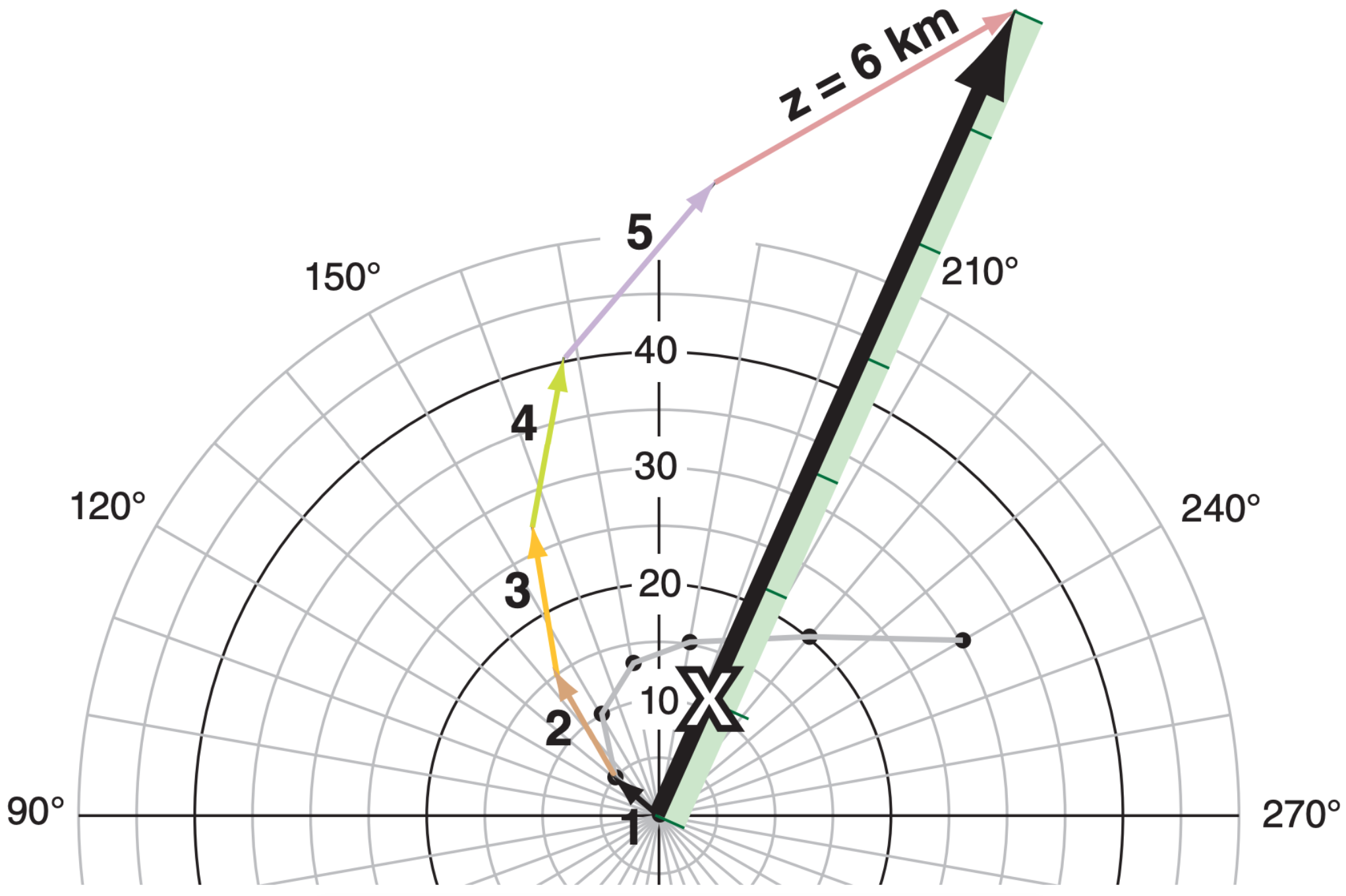
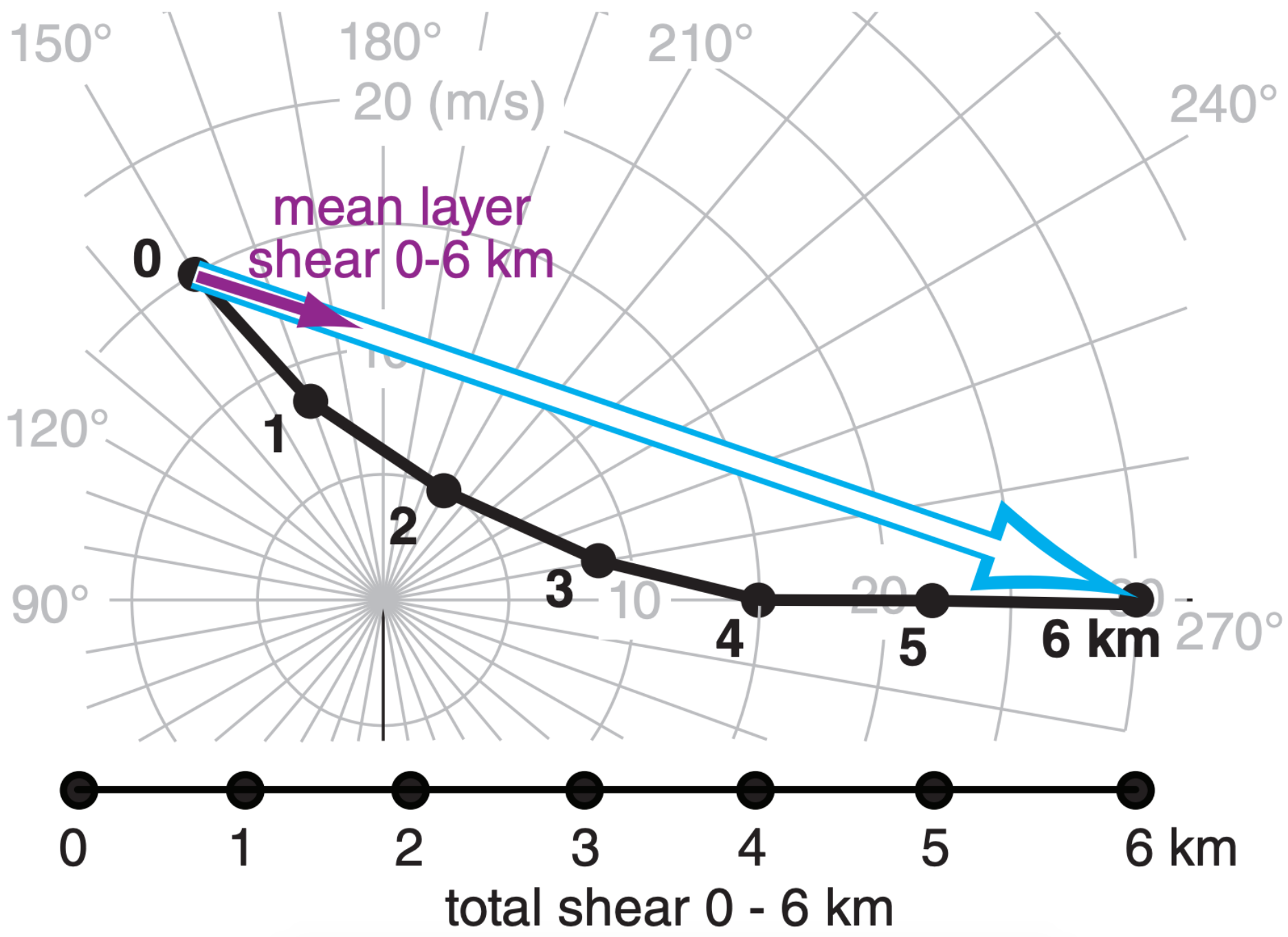
The total shear magnitude (TSM) across many layers, such as between 0 to 6 km, is the algebraic sum of the lengths of the individual line segments in the hodograph. For a simple graphic method that gives a good estimate of TSM, conceptually lay flat the grey line from Fig. 14.54 without stretching or shrinking any of the line segments. The length of the resulting straight line (black line in Fig. 14.54) indicates the total shear magnitude. For this example, the total shear magnitude is roughly 45 m s–1, comparing the length of the black line to the wind-speed circles in the graph. Again, wind difference is used as a surrogate for shear.
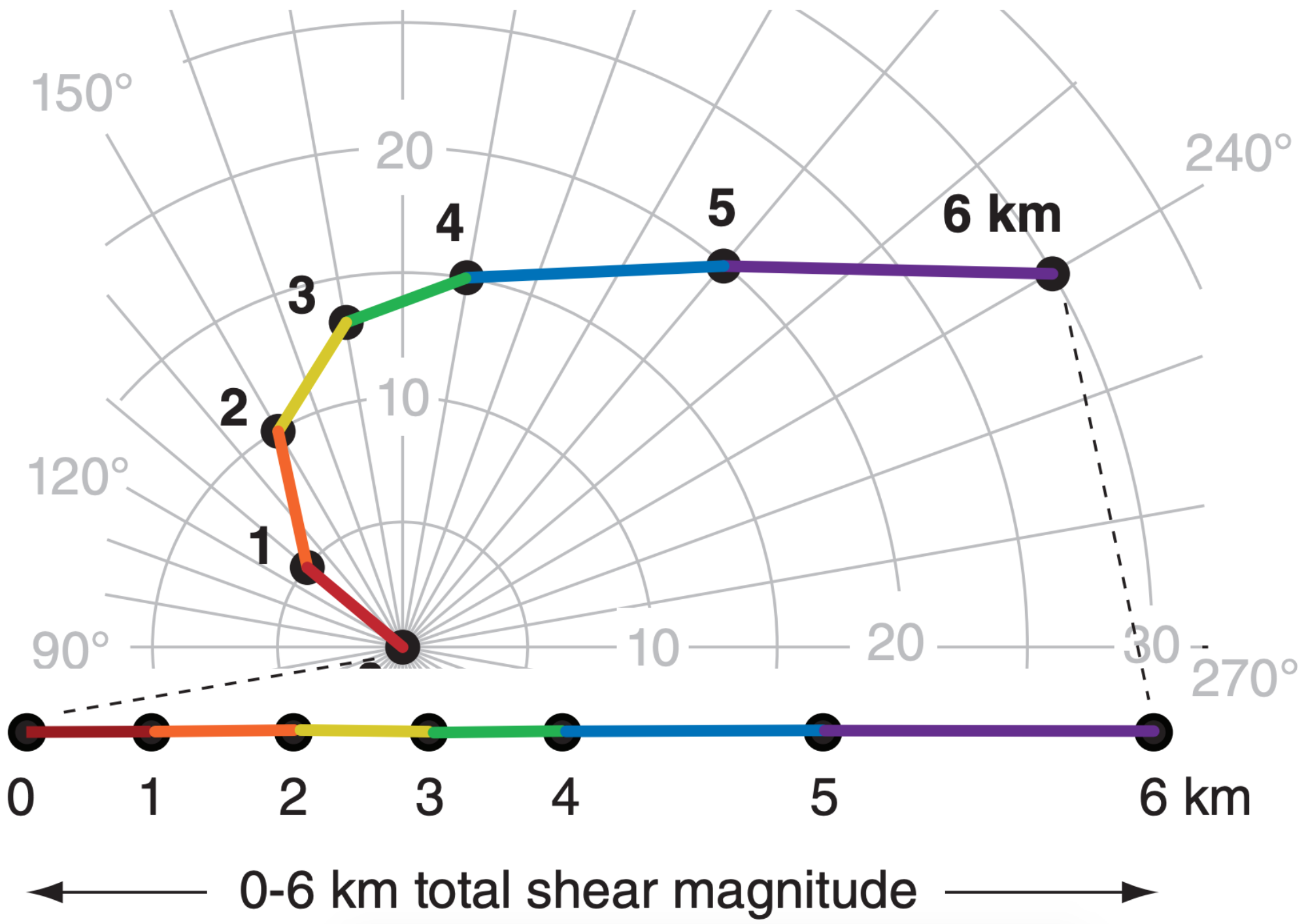
To find the total shear magnitude mathematically, sum over all the individual layer shear magnitudes (assuming layers of equal thickness):
\(\ \begin{align} T S M=\sum_{i=1}^{n}\left[\left(U_{i}-U_{i-1}\right)^{2}+\left(V_{i}-V_{i-1}\right)^{2}\right]^{1 / 2}\tag{14.17}\end{align}\)
where n is the total number of layers, and subscripts i and i–1 indicate the top and bottom, respectively, of the ith layer. For the Fig. 14.54 example, n = 6, and each layer is 1 km thick.
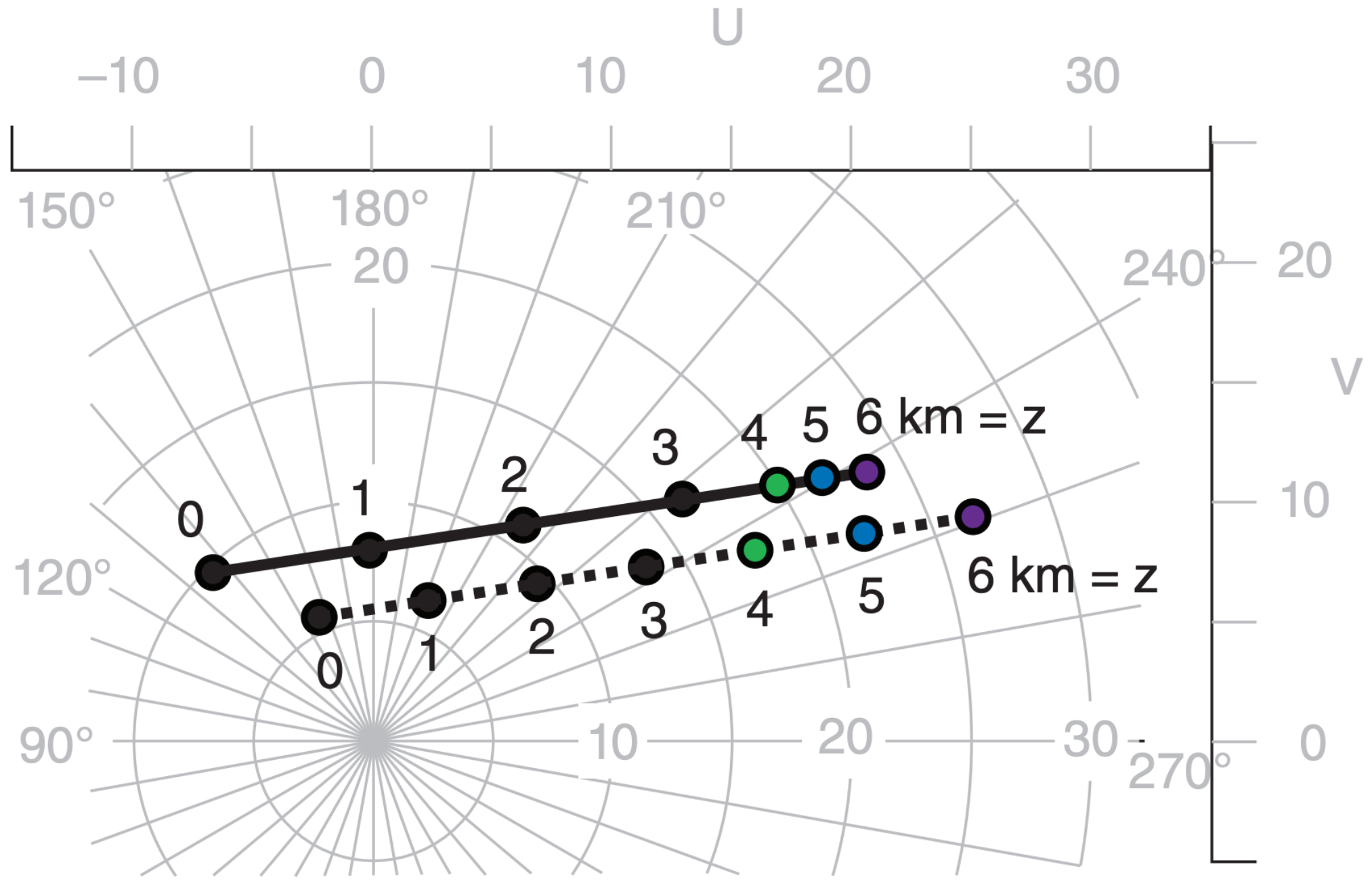
Although two different environments might have the same total wind shear (TSM), the distribution of that shear with height determines the types of storms possible. For example, Fig. 14.55 shows two hodographs with exactly the same total shear magnitude, but the solid line has most of that shear in the bottom 3 km (as seen by the spacing between height (z) points along the hodograph), favoring squall lines and bow echoes. The dashed line has evenly distributed shear through the bottom 6 km, favoring supercells. Fig. 14.56 also shows that greater TSM values support supercells.
14.5.2.4. Mean Environmental Wind (Normal Storm Motion)
Airmass thunderstorms often translate in the direction and speed of the mean environmental wind vector in the bottom 6 km of the atmosphere. These are sometimes called steering-level winds or normal winds. To find the 0 to 6 km mean wind (i.e., NOT the shear) first picture the wind vectors associated with each point on the hodograph (Fig. 14.57). Next, vector sum all these winds by moving them tail to head, as shown in Fig. 14.58).
Sample Application
How many fence posts are needed to support a straight fence with 6 sections?

Find the Answer 7 (see figure)
Exposition: Only 6 posts are needed for a closed loop.
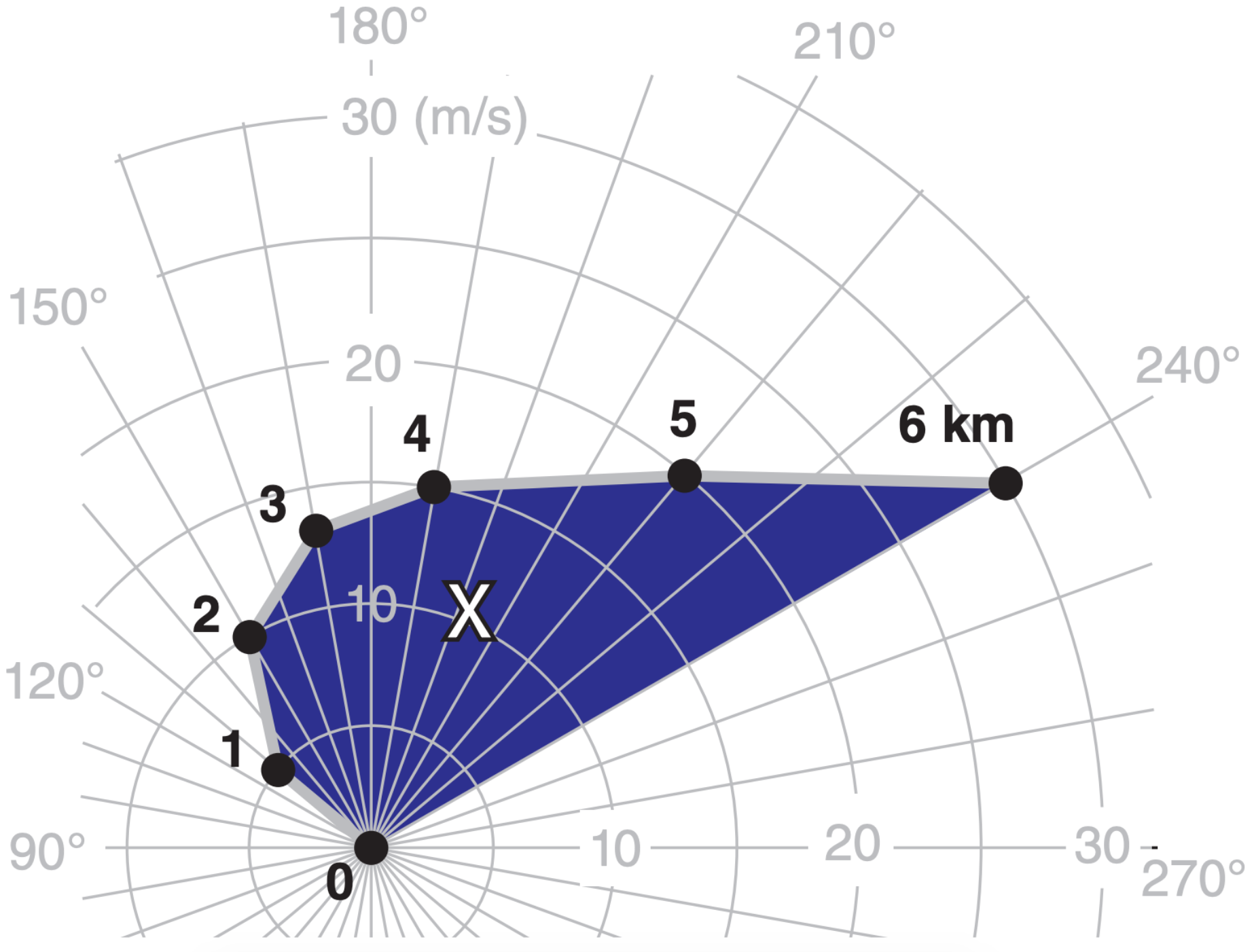
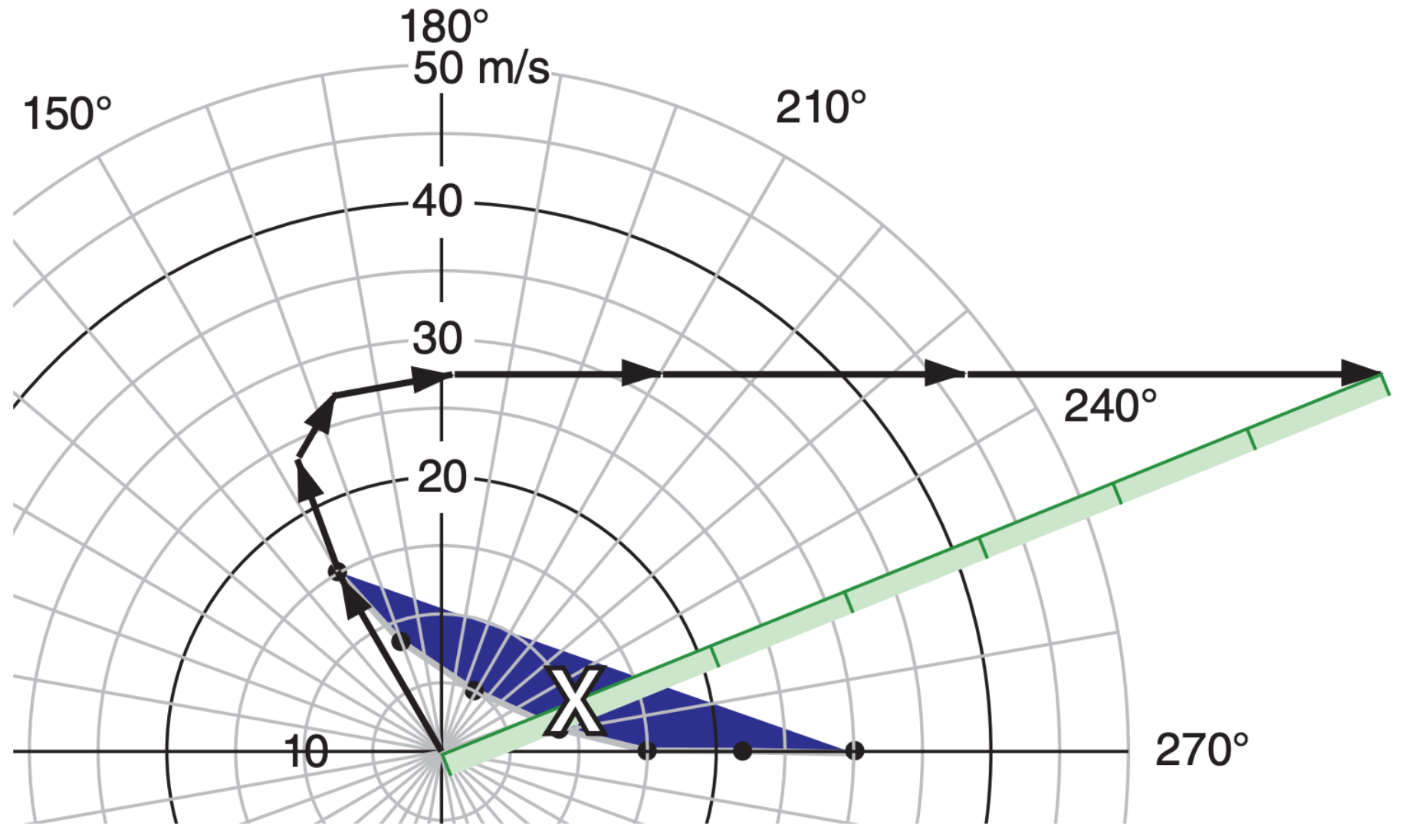
Finally, divide the vector sum by the number of wind points to find the mean wind vector, as indicated with the “X”. [CAUTION: As you can see in Fig. 14.58, the 6 layers of air are bounded by 7 windvector points, not forgetting the zero wind at the ground. Thus, the vector sum in Fig. 14.58 must be divided by 7, not by 6.] For this example, the mean wind (i.e., the location of the “X”) is from about 203° at 11 m s–1. An arrow from the origin to the “X” gives the forecast normal motion for thunderstorms.
An easier way to approximate this mean wind vector without doing a vector addition is to estimate (by eye) the center of mass of the area enclosed by the original hodograph (shaded black in Fig. 14.59; NOT the area enclosed by the re-positioned vectors of Fig. 14.58). Namely, if you were to: (1) trace that area onto a piece of cardboard, (2) cut it out, & (3) balance it on your finger tip, then the balance point (shown by the “X” in Fig. 14.59) marks the center of mass. “X” indicates the normal motion of thunderstorms, and is easy to estimate graphically using this center-of-mass estimate.
To mathematically calculate the mean wind (indicated with overbars) in the bottom 6 km of the atmosphere, first convert the vectors from speed and direction to their Cartesian components. Then sum the U velocities at all equally-spaced heights, and separately sum all the V velocities, and divide those sums by the number of velocity heights to get the components \((\bar{U}, \bar{V})\) of the mean wind.
\(\ \begin{align} \bar{U}=\frac{1}{N} \sum_{j=0}^{N} u_{j}\tag{14.18}\end{align}\)
\(\ \begin{align} \bar{V}=\frac{1}{N} \sum_{j=0}^{N} V_{j}\tag{14.19}\end{align}\)
where N is the number of wind levels (not the number of shear layers), and j is the altitude index. For winds every 1 km from z = 0 to z = 6 km, then N = 7. [CAUTION: Don’t confuse the mean wind given above with the mean shear of eqs. (14.13 & 14.14).] Then, use eqs. (1.1) and (1.2) [similar to eqs. (14.15 & 14.16), but for mean wind instead of mean shear] to convert from Cartesian coordinates to mean speed and direction \((\bar{M}, \bar{\alpha})\).
In summary the normal motion of thunderstorms is \((\bar{U}, \bar{V})\), or equivalently \((\bar{M}, \bar{\alpha})\). This corresponds to the center-of-mass “X” estimate of Fig. 14.59.
Charles Doswell’s web page recommends stormchase guidelines, which I paraphrase here:
The #1 Threat: Being on the Highways
- Avoid chasing alone.
- Be very alert to standing water on the roads.
- Avoid chasing in cities if at all possible.
- Don’t speed.
- Pull fully off the road when you park.
- Use your turn signals.
- Slow down in poor visibility (rain; blowing dust).
- Plan where to get fuel; don’t let your tank get low.
- Avoid unpaved roads (very slippery when wet).
- Make your vehicle visible to other vehicles.
(continues in the next chapter).
Sample Application
Mathematically calculate the mean wind components, magnitude, and direction for the hodograph of Fig. 14.57 (i.e., using data table from the Sample Application about 5 pages ago).
Find the Answer
Given: The data table from 5 pages ago, copied below.
Find: \((\bar{U}, \bar{V})\) = ? (m s–1), \((\bar{M}, \bar{\alpha})\) = ? (m s–1, °)
Use a spreadsheet to do these tedious calculations: First, use eqs. (1.3) and (1.4) to find U and V components of the winds. Next, average the U’s, and then the V’s, using eqs. (14.18 & 14.19). Finally, use eqs. (1.1) and (1.2) to covert to speed and direction.
All velocities below are (m s–1), direction is in degrees, and height z in km.
| z | direction | M | U | V |
|---|---|---|---|---|
| 6 | 240 | 30 | 26.0 | 15.0 |
| 5 | 220 | 20 | 12.9 | 15.3 |
| 4 | 190 | 15 | 2.6 | 14.8 |
| 3 | 170 | 13 | -2.3 | 12.8 |
| 2 | 150 | 10 | -5.0 | 8.7 |
| 1 | 130 | 5 | -3.8 | 3.2 |
| 0 | 0 | 0 | 0.0 | 0.0 |
| Mean wind components (ms–1)= | 4.3 | 10.0 | ||
| Mean wind magnitude (m s–1) = | 10.9 | |||
| Mean wind direction (°) = | 203.5 | |||
Check: Units OK. Magnitudes and signs reasonable.
Exposition: The graphical estimate of mean wind speed 11 m s–1 and direction 203° by eye from Figs. 14.58 and 14.59 were very close to the calculated values here. Namely, expect normal thunderstorms to move from the south southwest at about 10.9 m s–1 in this environment. Thus, the center-of-mass estimate by eye saves a lot of time and gives a reasonable answer.
14.5.2.5. Supercell Storm Motion
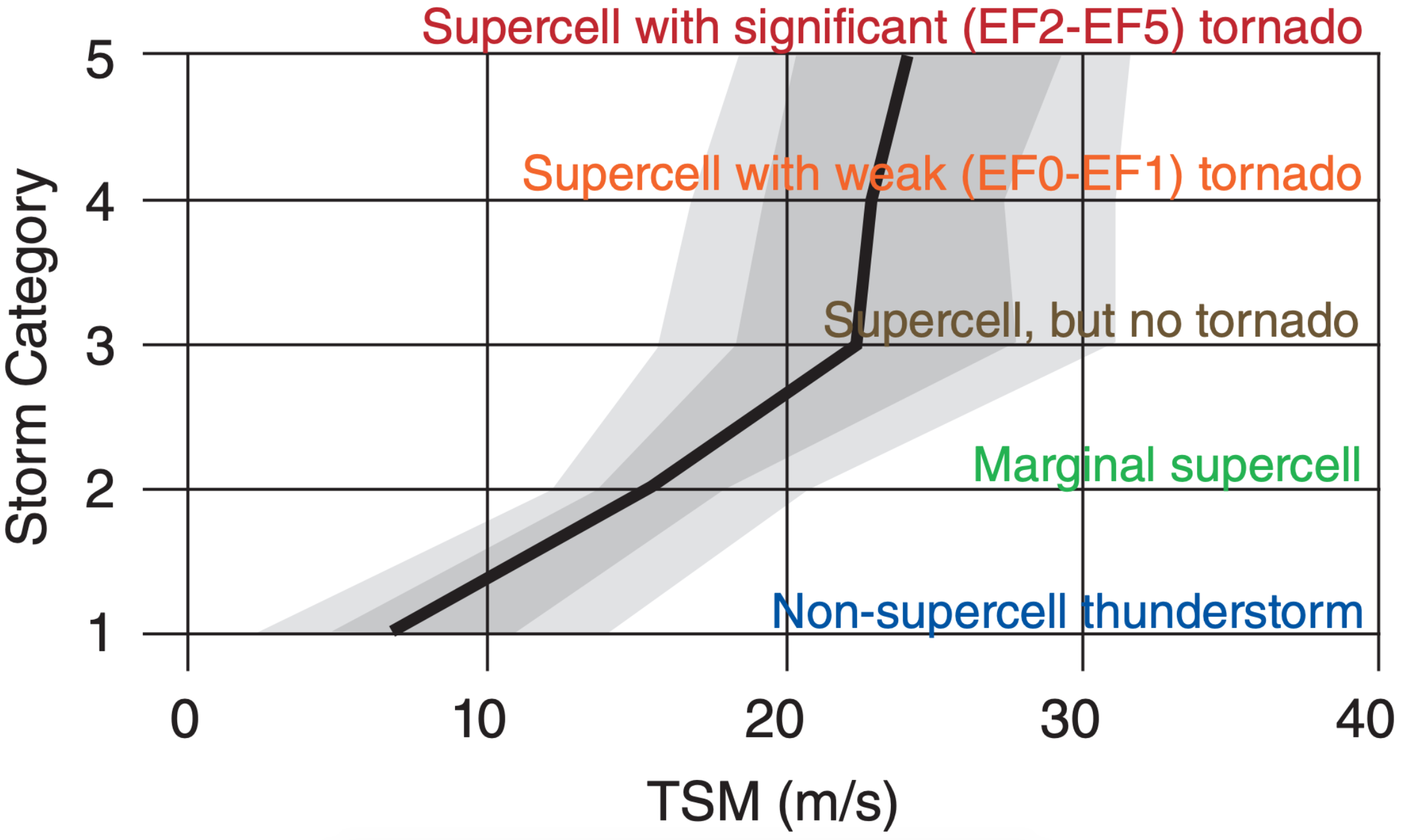
Supercell thunderstorms have rotating updrafts, and to get that rotation they need sufficient environmental wind shear. The environmental total shear magnitude typically must be TSM ≥ 25 m s–1 and be distributed relatively evenly across the lower troposphere (from the surface to 6 km) to support supercells. Total shear magnitudes less than 15 m s–1 often are too small for supercells. In between those two shears, thunderstorms may or may not evolve into supercells.
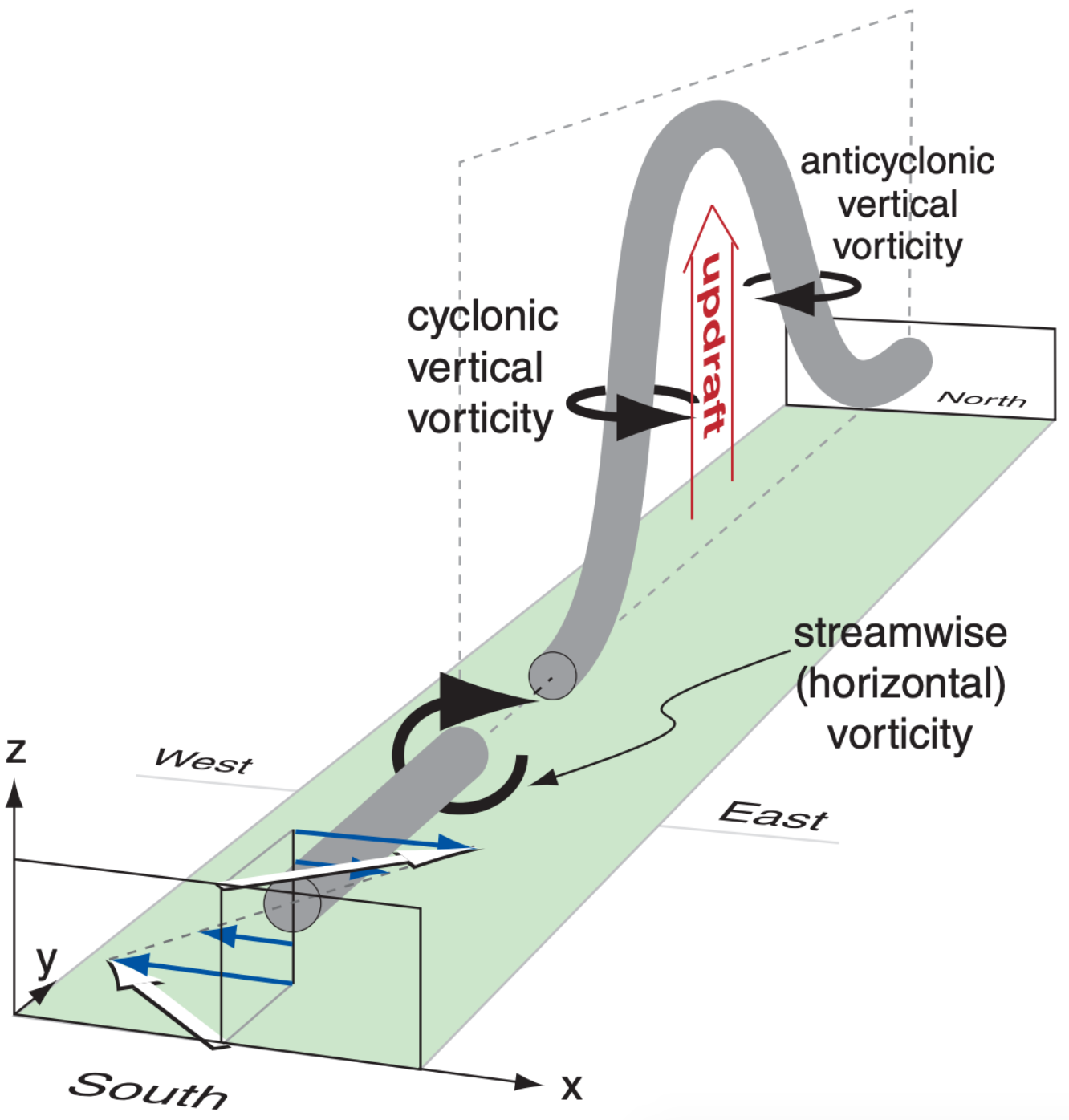
One reason that strong shear promotes supercells is that horizontal vorticity associated with vertical shear of the horizontal wind in the environment can be tilted into vertical vorticity by the strong convective updraft of a thunderstorm (Fig. 14.60). This causes counter-rotating vortices (mesocyclones) on the left and right sides of the updraft. These two mesocyclones are deep (fill the troposphere) and have diameters roughly equal to the tropospheric depth. One is cyclonic; the other is anticyclonic.
Sometimes supercell thunderstorms split into two separate storms: right-moving and left-moving supercells. Namely, the cyclonic and anticyclonic mesocyclones support their own supercells, and move right and left of the “normal” motion as would have been expected from the mean steeringlevel wind.
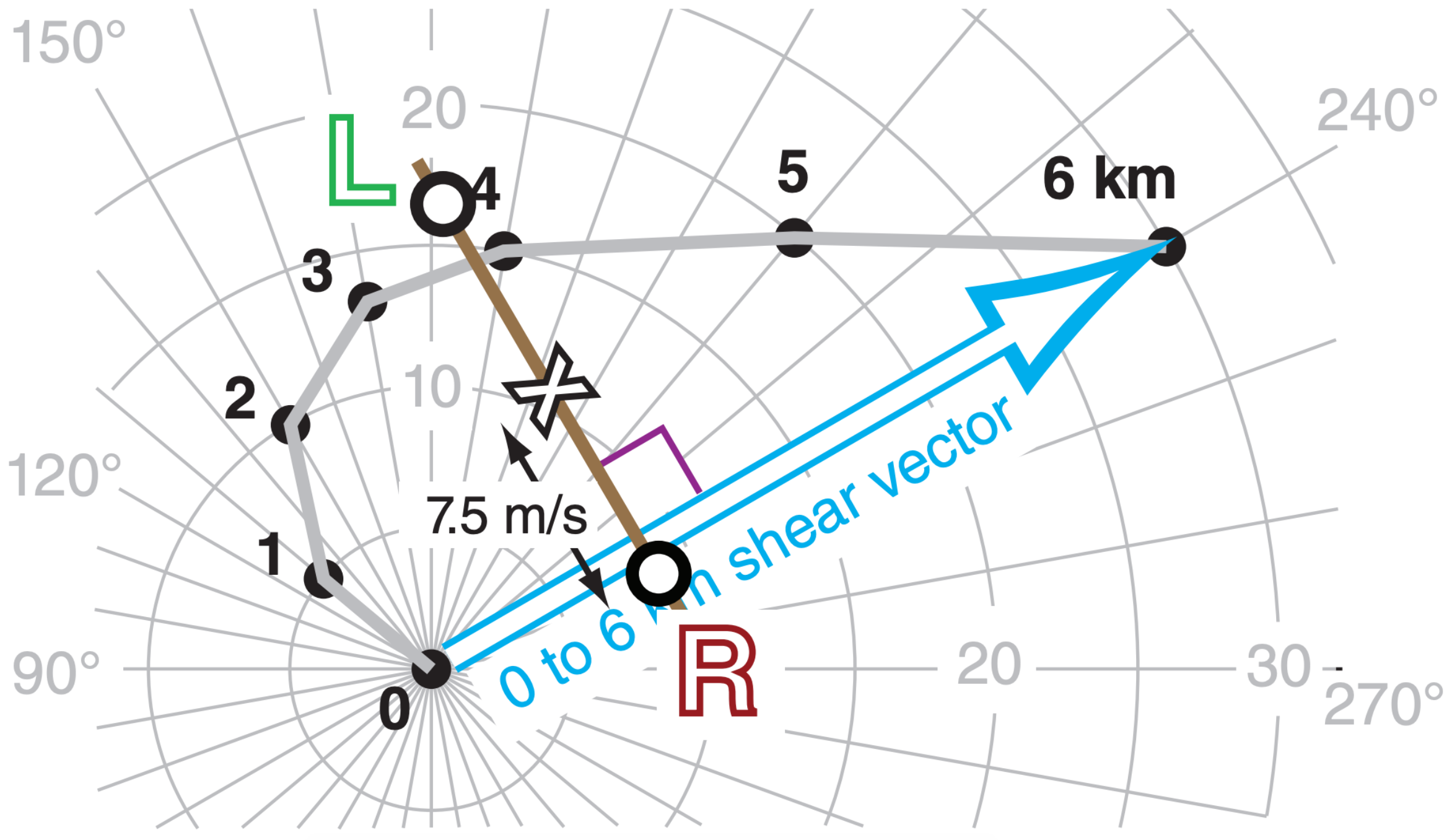
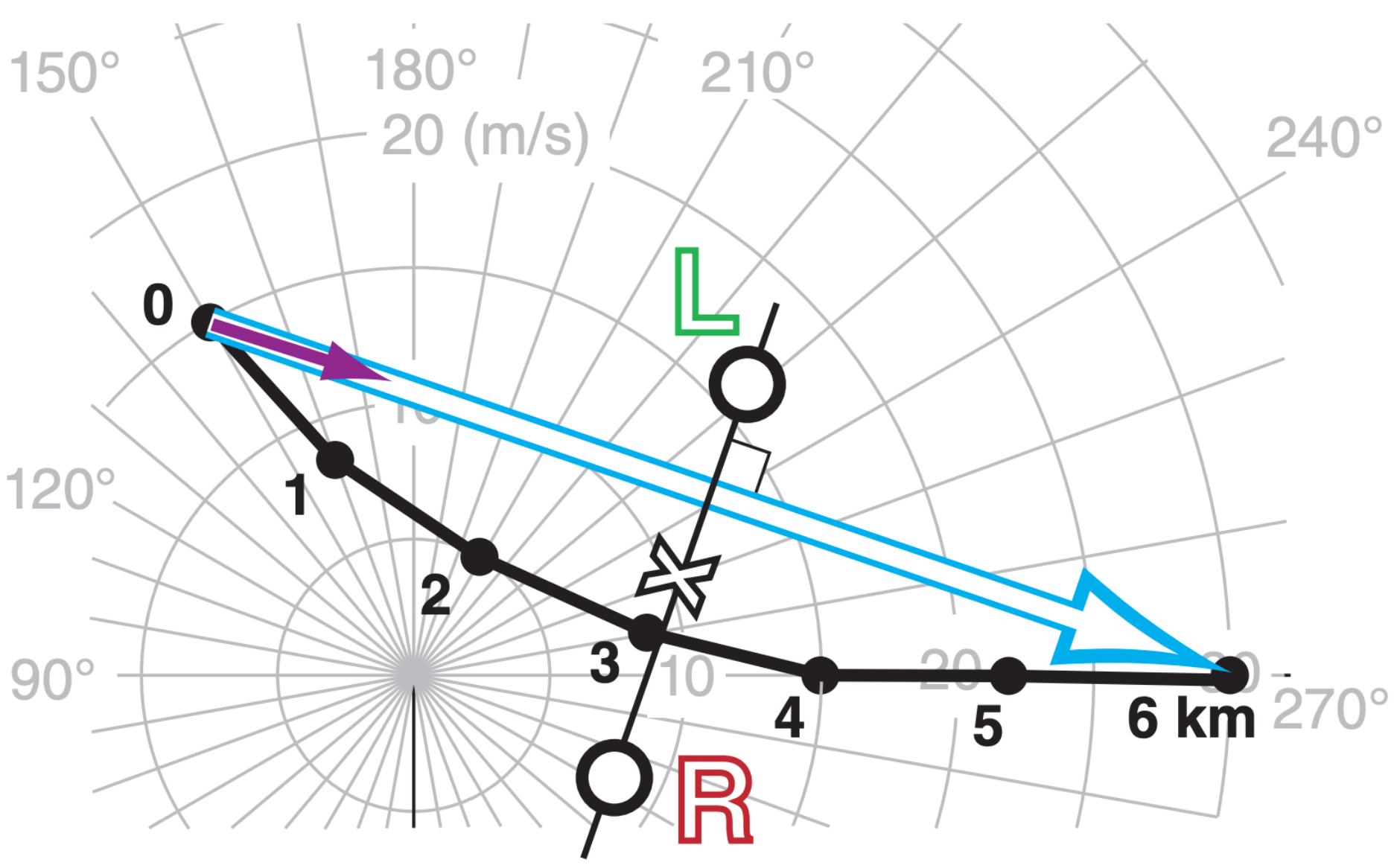
Bunker’s Internal Dynamics (ID) method to forecast the movement of these right and left-moving supercells is:
- Find the 0 to 6 km mean wind (see “X” in Fig. 14.61), using methods already described. This would give normal motion for thunder- storms. But supercells are not normal.
- Find the 0.25 to 5.75 km layer shear vector (approximated by the 0 to 6 km layer shear, shown with the white arrow in Fig. 14.61).
- Through the mean wind point (X), draw a line (black line in Fig. 14.61) perpendicular to the 0 to 6 km shear vector.
- On the line, mark 2 points (white in black circles): one 7.5 m s–1 to the right and the other 7.5 m s–1 to the left of the “X”.
- The point that is right (clockwise) from the mean wind “X” gives the average motion forecast for the right (R) moving storm.
- The point that is left (counter-clockwise) from the mean wind “X” gives the average forecast motion of the left (L) moving storm.
Not all storms follow this simple rule, but most supercell motions are within a 5 m s–1 radius of error around the R and L points indicated here. For the example in Fig. 14.61, motion of the right-moving supercell is 8 m s–1 from 246°, and the left mover is 17 m s–1 from 182°.
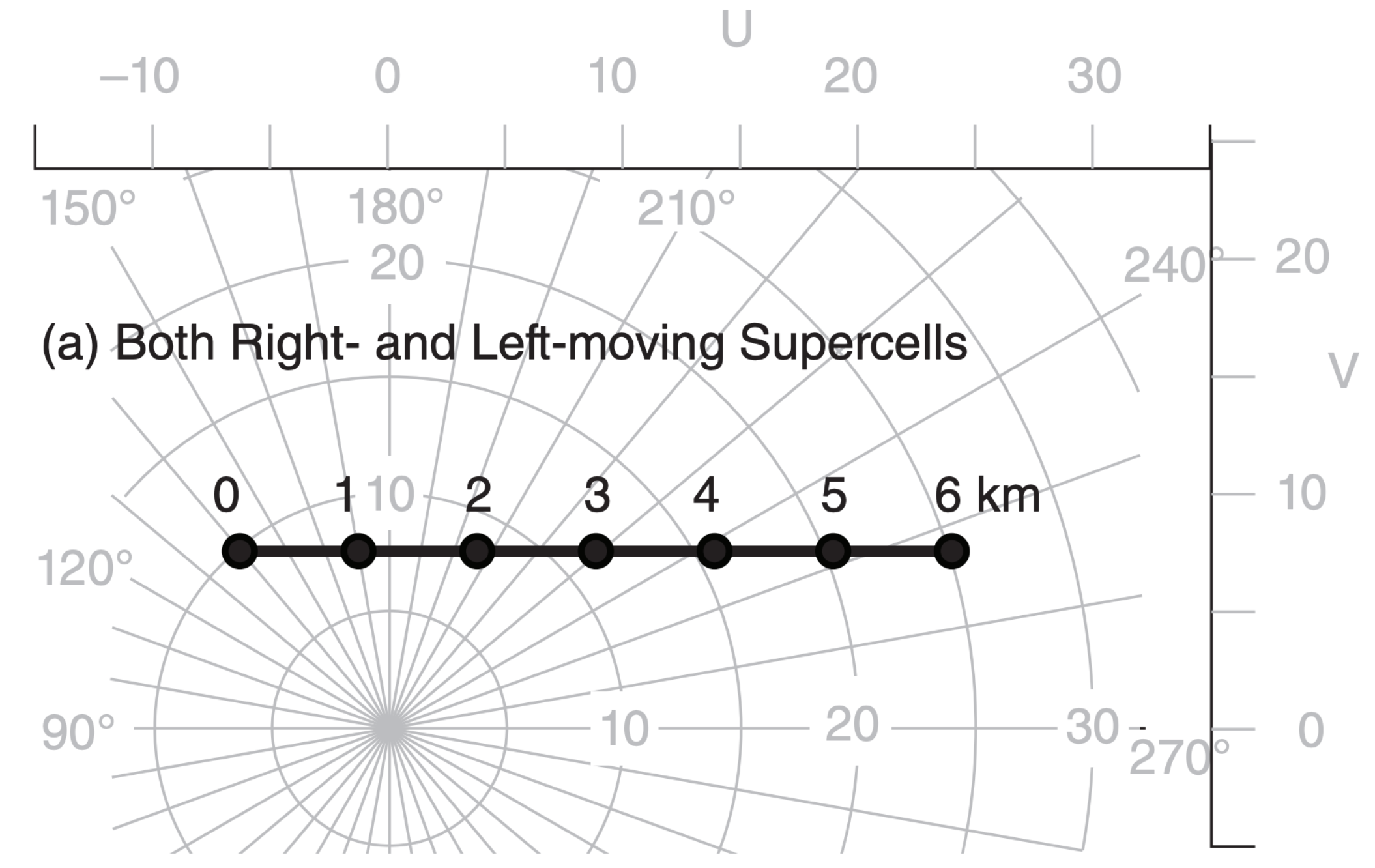
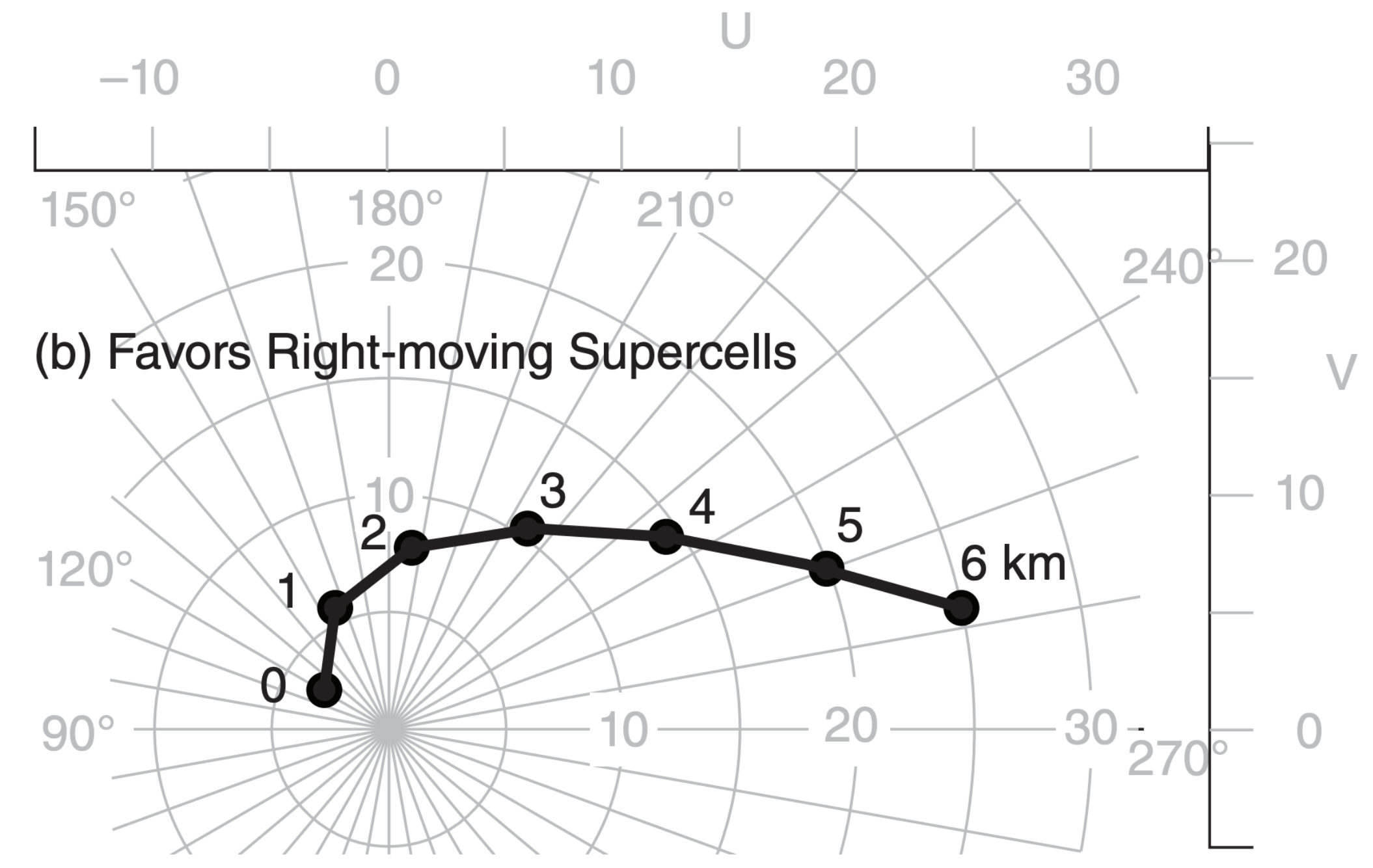
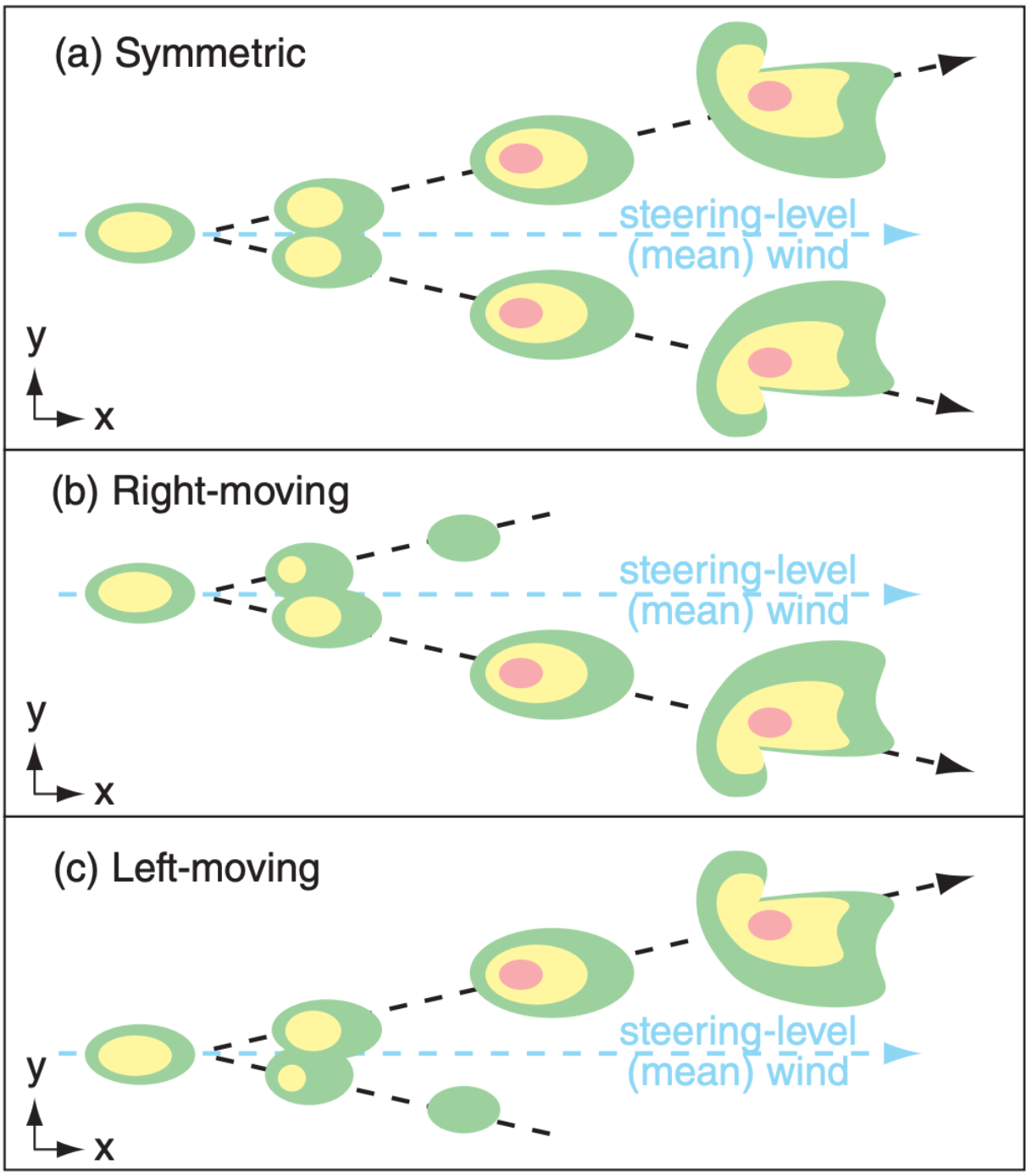
Only for straight hodographs (Fig. 14.62a) are the right- and left-moving supercells expected to exist simultaneously with roughly equal strength (Fig. 14.63a). Hodographs that curve clockwise (Fig. 14.62b) with increasing height favor right-moving supercell thunderstorms (Fig. 14.63b). For this situation, the left-moving storm often dissipates, leaving only a right-moving supercell.
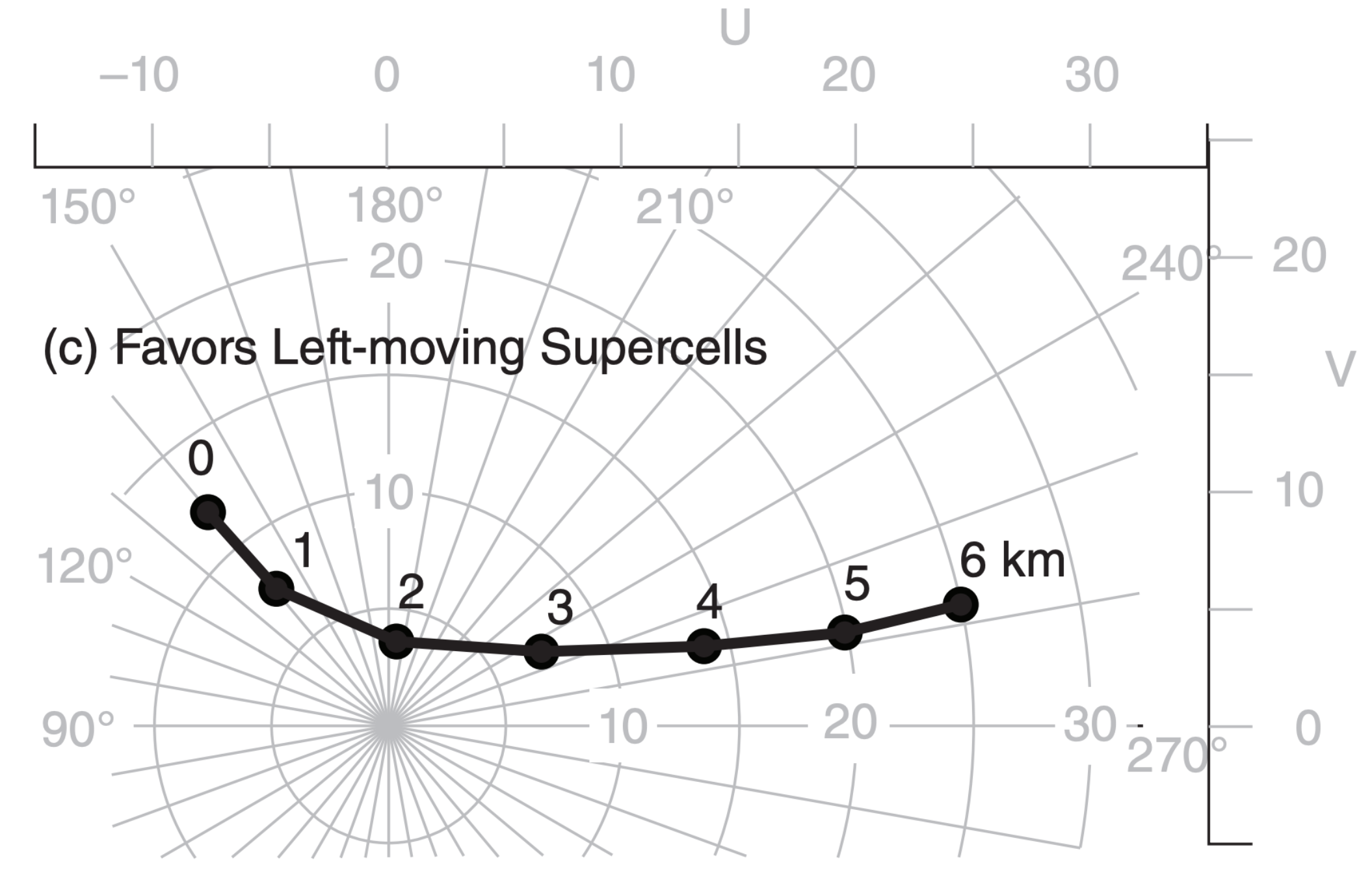
Hodographs that curve counterclockwise (Fig. 14.62c) favor left-moving supercells (Fig. 14.63c), with the right-moving storm often dissipating. In the plains/prairies of North America, most hodographs curve clockwise (as in Figs. 14.61 and 14.62b), resulting in right-moving supercell thunderstorms that are about ten times more abundant than leftmoving storms. The opposite is true in the S. Hemisphere for midlatitude thunderstorms.
In summary, supercell thunderstorms often do not move in the same direction as the mean environmental (steering-level) wind, and do not move at the mean wind speed. The methods that were discussed here are useful to forecast movement of storms that haven’t formed yet. However, once storms form, you can more accurately estimate their motion by tracking them on radar or satellite. Regardless of the amounts of shear, CAPE, and moisture in the pre-storm environment, thunderstorms won’t form unless there is a trigger mechanism. Triggers are discussed in the next main section (after the Bulk Richardson Number subsection).
Sample Application
Given the wind profile in the table below:
| z (km) | dir. (°) | speed (ms-1) | z (km) | dir. (°) | speed (m s-1) |
|---|---|---|---|---|---|
| 0 | 150 | 15 | 4 | 270 | 15 |
| 1 | 160 | 8 | 5 | 270 | 22 |
| 2 | 210 | 5 | 6 | 270 | 30 |
| 3 | 260 | 8 |
- Plot the hodograph;
- Graphically find the mean wind-shear vector for the 0 to 6 km layer;
- Graphically find the total shear magnitude.
- Does this hodograph favor bow echoes or supercells? Why?
- Graphically find the 0 to 6 km mean wind.
- Graphically find the motions for the right and left-moving supercell thunderstorms.
- Which is favored: right or left-moving supercell thunderstorms? Why?
Find the Answer
- Plot the hodograph (see curved black line below):
- The 0 to 6 km shear vector is shown with the white arrow. Dividing by 6 gives a mean layer shear (red arrow) of about 7 m s–1 from the west northwest.
- The total shear magnitude, shown at the bottom of the hodograph above, is about 42 m s–1.
- Supercells are favored, because shear is evenly distributed throughout the bottom 6 km of atmosphere. Also, the total shear magnitude is greater than 25 m s–1, as required for supercells.
- The answer is a vector, drawn from the origin to the “X” (see hodograph in next column). Namely, the mean wind is from about 250° at 10 m s–1. Vector method roughly agrees with center-of-mass method, done by eye.
Thus, for normal thunderstorms (and for the first supercell, before it splits into right and left-moving supercells) forecast them to move from the west southwest at about 10 m s–1. For both this example and Fig. 14.59, the center-of-mass method gives a better estimate if we look at the hodograph area between z = 0.5 and 5.5 km.
- Bunker’s Internal Dynamics method is shown graphically on the hodograph below. The left supercell (L) is forecast to move at 16 m s–1 from 229°, and the right supercell (R) at 8 m s–1 from 298°.
- Left-moving supercells are favored, because the hodograph curves counterclockwise with increasing height. This storm moves faster than the mean wind (i.e., faster than the “normal” thunderstorm steeringlevel wind).
Exposition: This hodograph shows veering winds (winds turning clockwise with increasing altitude), implying warm-air advection according to the thermal-wind relationship. Even though the wind vectors veer clockwise, the hodograph curvature is counterclockwise (implying dominant left-moving supercell storms), so be careful not to confuse these two characteristics.
Winds alone are not sufficient to forecast whether thunderstorms will occur. Other key ingredients are instability and abundant moisture, as previously discussed. Also needed is sufficient lifting by a trigger mechanism to overcome the capping inversion.
14.5.2.6. Bulk Richardson Number
Thunderstorm type depends on the amounts of both instability and wind shear in the pre-storm environmental sounding. The bulk Richardson number (BRN) is the ratio of nonlocal instability (i.e., the CAPE) in the mid to upper part of the troposphere to shear (∆M) in the lower half of the troposphere:
\(\ \begin{align} B R N=\frac{C A P E}{0.5 \cdot(\Delta M)^{2}}\tag{14.20}\end{align}\)
Table 14-2 summarizes the utility of the BRN. The BRN is dimensionless, because the units of CAPE (J·kg–1) are the same as (m2·s–2) (see Appendix A).
In the denominator, the shear magnitude (∆M) is given by:
\(\ \begin{align} \Delta M=\left[(\Delta U)^{2}+(\Delta V)^{2}\right]^{1 / 2}\tag{14.21}\end{align}\)
where
\(\ \begin{align} \Delta U=\bar{U}-U_{S L}\tag{14.22a}\end{align}\)
\(\ \begin{align} \Delta V=\bar{V}-V_{S L}\tag{14.22b}\end{align}\)
Eqs. (14.22) are the shear components between the mean wind \((\bar{U}, \bar{V})\) in the 0 to 6 km layer, and the surface-layer winds (USL, VSL) estimated as an average over the bottom 0.5 km of the atmosphere. Fig. 14.64 shows how to estimate this shear magnitude from a hodograph.

The denominator of the BRN, known as the bulk-Richardson-number shear (BRN Shear), is
\(\ \begin{align} BRN Shear =0.5 \cdot(\Delta M)^{2}\tag{14.23}\end{align}\)
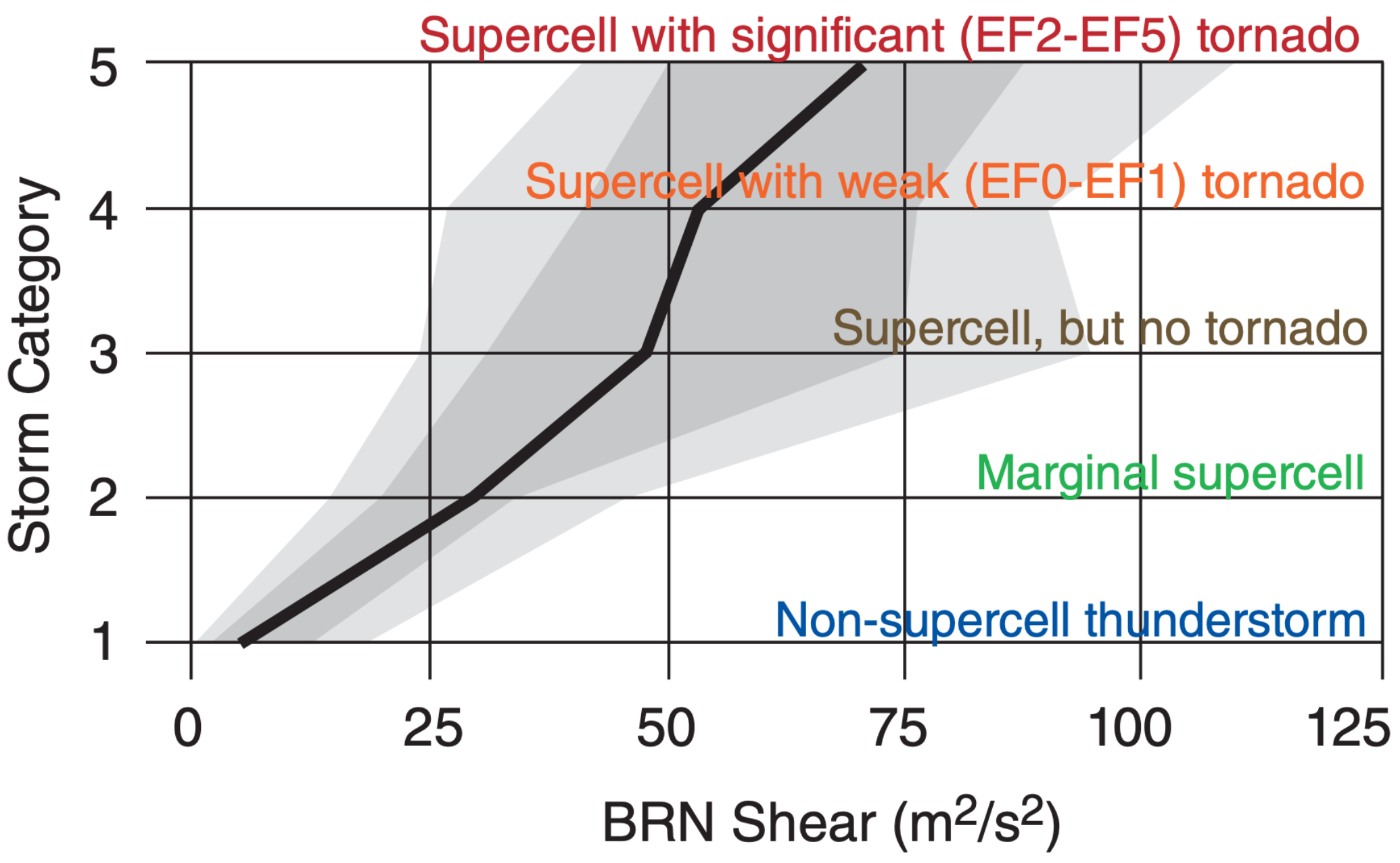
which has units of (m2·s–2). BRN shear can help indicate which supercells might be tornadic (see Table 14-3). Although BRN shear is statistically sharper than ML-CAPE, MU-CAPE and ML-LCL in its ability to discriminate between thunderstorms and tornadoes of different severity, there is still significant overlap in thunderstorm/tornado categories as was found by verification against several hundred thunderstorms in central N. America (Fig. 14.65).
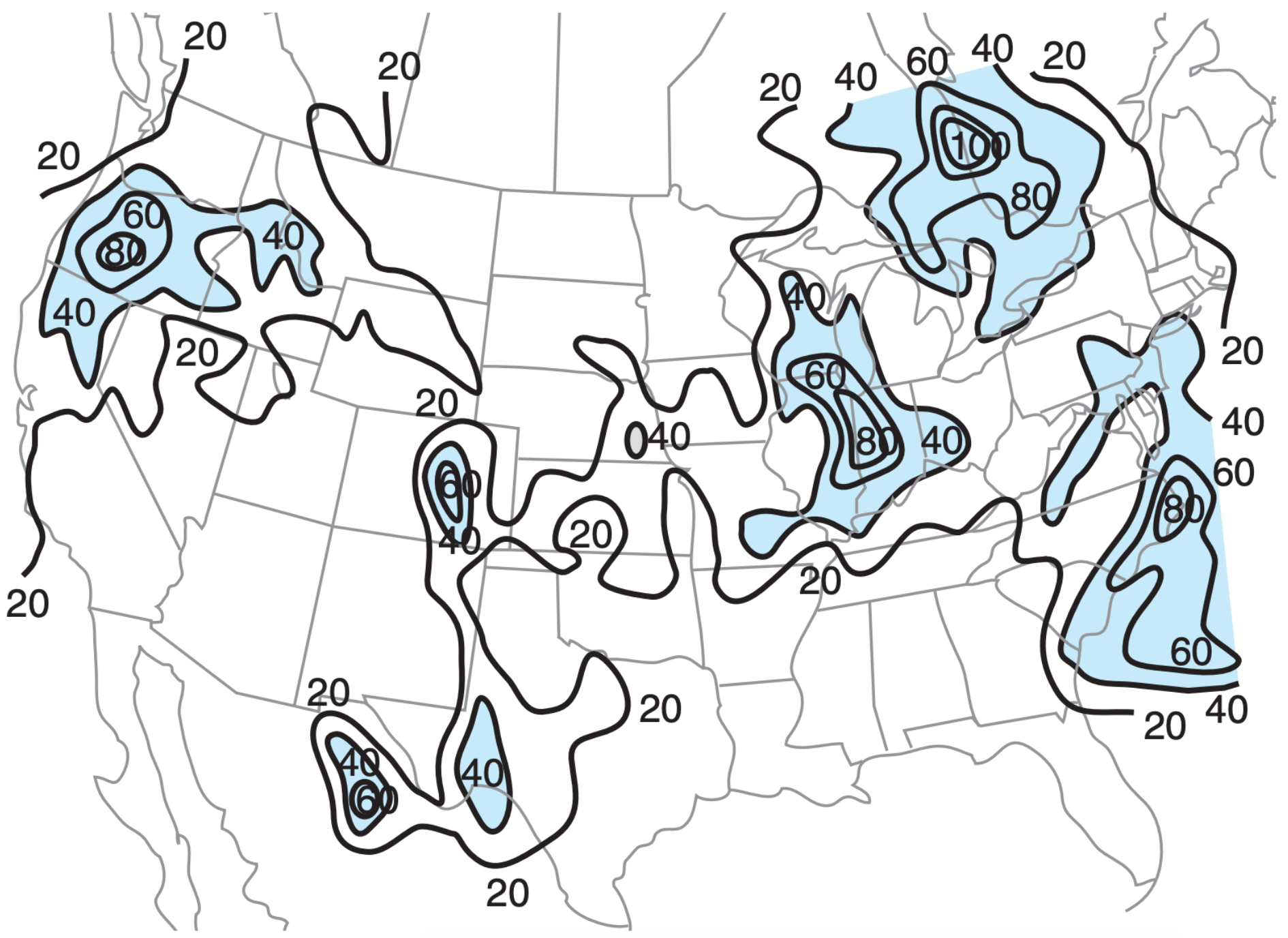
A case study weather map of BRN shear is analyzed in Fig. 14.66. As before, you should NOT use BRN shear alone as an indicator of tornado likelihood, because additional conditions must also be satisfied in order to allow tornadic thunderstorms to form. Some of these other conditions are instability, and a trigger mechanism.
| Table 14-2. Thunderstorm type determination using the bulk Richardson number (BRN) | |
| BRN | Thunderstorm Type |
|---|---|
| < 10 | (unlikely to have severe thunderstorms) |
| 10 - 45 | Supercells |
| 45 - 50 | Supercells and/or multicells |
| > 50 | Multicells |
| Table 14-3. If supercell thunderstorms form, then the bulk-Richardson-number shear (BRN Shear) suggests whether they might have tornadoes. | |
| BRN Shear (m2·s–2) | Tornadic Supercells |
|---|---|
| 25 - 35 | less likely, but possible |
| 35 - 45 | likely |
| 45 - 100 | more likely |
Sample Application
For the previous Sample Application, assume the associated CAPE = 3000 J·kg–1. (a) Find the BRN shear. (b) Find the BRN. (c) Are tornadic supercells likely?
Find the Answer
Given: CAPE = 3000 J·kg–1 hodograph = previous Sample Application
Find: BRN shear = ? m2·s–2 BRN = ? (dimensionless) Yes/no: thunderstorms? supercells? tornadoes?
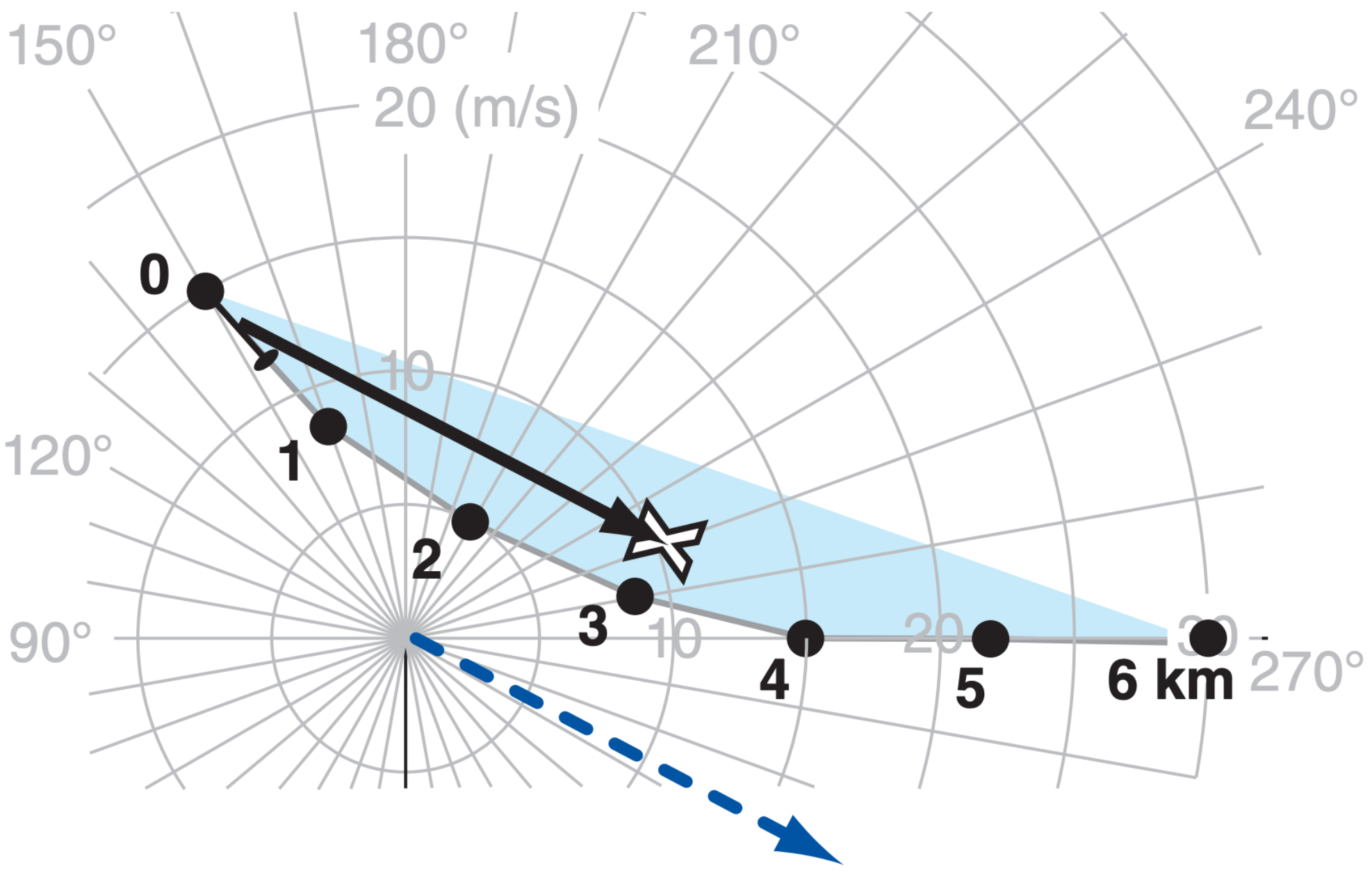
- On the hodograph from the previous Sample Application, put the tail of a vector (solid black arrow) a quarter of the way from the z = 0 point to the z = 1 point on the hodograph). Put the arrowhead on the “X” mean wind. Then measure the vector’s length (see dashed arrow), which is about ∆M = 18 m s–1.
Then use eq. (14.23): BRN Shear = 0.5·(18 m s–1)2 = 162 m2 s–2
- Use eq. (14.20): BRN = (3000 m2 s–2) / (162 m2 s–2) = 18.5
- Assume CAPE ≈ MLCAPE. Then from Table 14-1: Severe thunderstorms likely, possible tornado.
Next, use Table 14-2: Thunderstorm type = supercell. Finally, use Table 14-3: Tornadoes are likely.
14.5.2.7. The Effective Layer
Most of the shear calculations shown so far have assumed that all thunderstorms are alike. Namely, all storms are roughly 12 km tall, and they all ingest the bottom 6 km of environmental air into the storm. That is why we have been plotting on the hodograph the 0 to 6 km winds. However, some storms are taller and some are shorter.
Also, for thunderstorms at night and early morning, a cold layer of nocturnal boundary-layer air might resist vertical motion because of its static stability, and thus not be ingested into the thunderstorm. These storms have elevated inflows with their inflow base zinflow_base at some height above ground. But for most afternoon and evening thunderstorms, zinflow_base = 0.
An effective thunderstorm depth is defined as ∆zstorm = zEL – zinflow_base . This definition works for tall and short storms (with high and low equilibrium levels, zEL, respectively), and for storms with zero and nonzero inflow-base altitude. An effective-layer bulk shear is then defined across the bottom half of the effective thunderstorm depth. This effective bulk shear would be used in place of the BRN shear previously discussed, to anticipate thunderstorm characteristics. Supercells are more likely when effective bulk wind difference is 12 to 20 m·s–1 or greater. The effective bulk shear is the same as the 0 to 6 km shear for average storms.


