10.7: Vertical Forces and Motion
- Page ID
- 10198
Forces acting in the vertical can cause or change vertical velocities, according to Newton’s Second Law. In an Eulerian framework, the vertical component of the equations of motion is:
\(\frac{\Delta W}{\Delta t}=-U \frac{\Delta W}{\Delta x}-V \frac{\Delta W}{\Delta y}-W \frac{\Delta W}{\Delta z}-\frac{1}{\rho} \frac{\Delta P}{\Delta z}-|g|-\frac{F_{z T D}}{m}\)

where the vertical acceleration given in the left side of the equation is determined by the sum of all forces/mass acting in the vertical, as given on the right. For Cartesian directions (x, y, z) the velocity components are (U, V, W). Also in this equation are air density (ρ), pressure (P), vertical turbulent-drag force (Fz TD), mass (m), and time (t). Magnitude of gravitational acceleration is |g| = 9.8 m·s–2. Coriolis force is negligible in the vertical (see the INFO box on Coriolis Force in 3-D, earlier in this chapter), and is not included in the equation above.
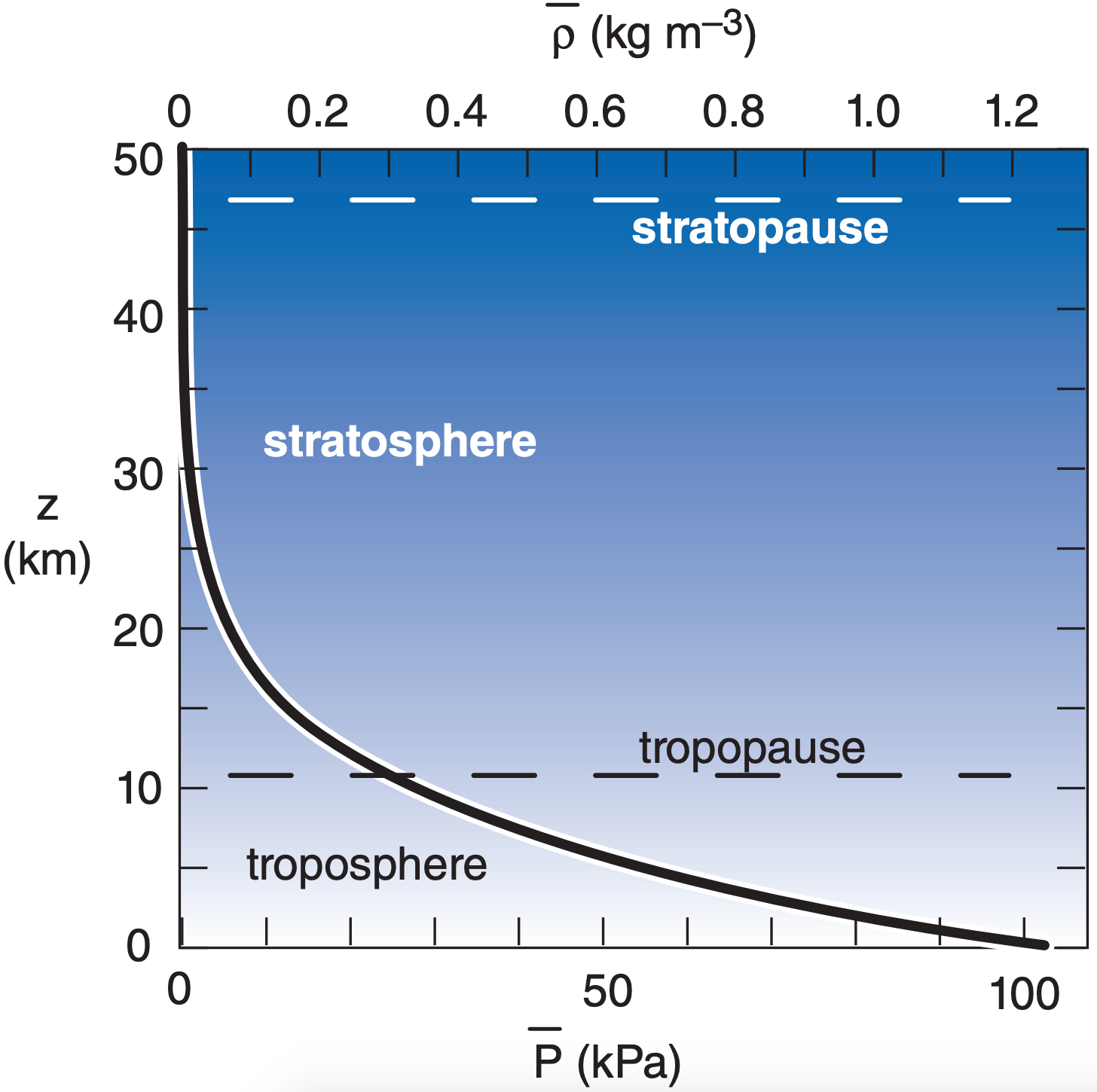
Recall from Chapter 1 that our atmosphere has an extremely large pressure gradient in the vertical, which is almost completely balanced by gravity (Fig. 10.25). Also, there is a large density gradient in the vertical. We can define these large terms as a mean background state or a reference state of the atmosphere. Use the overbar over variables to indicate their average background state. Define this background state such that it is exactly in hydrostatic balance (see Chapter 1):
\(\ \begin{align} \frac{\Delta \bar{P}}{\Delta z}=-\bar{\rho} \cdot|g|\tag{10.55}\end{align}\)
However, small deviations in density and pressure from the background state can drive important non-hydrostatic vertical motions, such as in thermals and thunderstorms. To discern these effects, we must first remove the background state from the full vertical equation of motion. From eq. (10.54), the gravity and pressure-gradient terms are:
\(\ \begin{align} \frac{1}{\rho}\left[-\frac{\Delta P}{\Delta z}-\rho|g|\right]\tag{10.56}\end{align}\)
But total density ρ can be divided into background \((\bar{\rho})\) and deviation \(\left(\rho^{\prime}\right)\) components: \(\rho=\bar{\rho}+\rho^{\prime}\). Do the same for pressure: \(P=\bar{P}+P^{\prime}\). Thus, eq. (10.56) can be expanded as:
\(\frac{1}{\left(\bar{\rho}+\rho^{\prime}\right)}\left[-\frac{\Delta \bar{P}}{\Delta z}-\frac{\Delta P^{\prime}}{\Delta z}-\bar{\rho}|g|-\rho^{\prime}|g|\right]\)
The first and third terms in square brackets in eq. (10.57) cancel out, due to hydrostatic balance (eq. 10.55) of the background state.
In the atmosphere, density perturbations \(\left(\rho^{\prime}\right)\) are usually much smaller than mean density. Thus density perturbations can be neglected everywhere except in the gravity term, where \(\rho^{\prime}|g| /\left(\bar{\rho}+\rho^{\prime}\right) \approx\left(\rho^{\prime} / \bar{\rho}\right) \cdot | g|\). This is called the Boussinesq approximation.
Recall from the chapters 1 and 5 that you can use virtual temperature \(\left(T_{v}\right)\) with the ideal gas law in place of air density (but changing the sign because low virtual temperatures imply high densities):
\(\ \begin{align} -\frac{\rho^{\prime}}{\bar{\rho}} \cdot|g|=\frac{\theta_{v}^{\prime}}{\bar{T}_{v}} \cdot|g|=\frac{\theta_{v} p-\theta_{v e}}{\bar{T}_{v e}} \cdot|g|=g^{\prime}\tag{10.58}\end{align}\)
where subscripts p & e indicate the air parcel and the environment surrounding the parcel, and where g’ is called the reduced gravity. The virtual potential temperature \(\theta_{v}\) can be in either Celsius or Kelvin, but units of Kelvin must be used for \(T_{v}\) and \(T_{v e}\).
\(\ \begin{align} \begin{aligned} \frac{\Delta W}{\Delta t}=-& U \frac{\Delta W}{\Delta x}-V \frac{\Delta W}{\Delta y}-W \frac{\Delta W}{\Delta z} -\frac{1}{\rho} \frac{\Delta P^{\prime}}{\Delta z}+\frac{\theta_{v p}-\theta_{v e}}{\bar{T}_{v e}} \cdot|g|-\frac{F_{z T D}}{m} \end{aligned}\tag{10.59}\end{align}\)
Terms from this equation will be used in the Regional Winds chapter and in the Thunderstorm chapters to explain strong vertical velocities.
When an air parcel rises or sinks it experiences resistance (turbulent drag, Fz TD) per unit mass m as it tries to move through the surrounding air. This is a completely different effect than air drag against the Earth’s surface, and is not described by the same drag equations. The nature of Fz TD is considered in the chapter on Air Pollution Dispersion, as it affects the rise of smoke-stack plumes. Fz TD = 0 if the air parcel and environment move at the same speed.
Sample Application
Suppose your neighborhood has a background environmental temperature of 20°C, but at your particular location the temperature is 26°C with a 4 m s–1 west wind and no vertical velocity. Just 3 km west is an 5 m s–1 updraft. Find the vertical acceleration.
Find the Answer
Given: Te = 273+20 = 293 K, ∆θ = 6°C, U= 4 m s–1
∆W/∆x= (5 m s–1 – 0) / (–3,000m – 0)
Find: ∆W/∆t = ? m·s–2
Assume: Because W = 0, there is zero drag. Because the air is dry: Tv = T. Given no V info, assume zero.
Apply eq. (10.59): \(\frac{\Delta W}{\Delta t}=-U \frac{\Delta W}{\Delta x}+\frac{\theta_{p}-\theta_{e}}{\bar{T}_{e}} \cdot|g|\)
∆W/∆t = –(4 m s–1)·(–5m·s–1/3,000m)+(6/293)·(9.8m·s–2) = 0.0067 + 0.20 = 0.21 m·s–2
Check: Physics & units are reasonable.
Exposition: Buoyancy dominated over advection for this example. Although drag was zero initially because of zero initial vertical velocity, we must include the drag term once the updraft forms.


