12.6: Oscillatory-Flow and Combined-Flow Bed Configurations
- Page ID
- 4230
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Introduction
As described in Chapter 6, water-surface waves propagating in water much shallower than the wavelength cause a back-and-forth motion of the water at the bottom. If the maximum speed of the water (which is attained in the middle of the oscillation) exceeds the threshold for sediment movement, oscillatory-flow bed forms develop. This is common in the shallow ocean. Swell from distant storms causes bottom oscillatory motion even though the weather is fine and calm locally. More importantly, bottom-water motions under large storm waves cause bed forms also. In that situation there is likely to be a non-negligible unidirectional current as well, resulting in a combined flow.
A Tank Experiment on Oscillatory-Flow Bed Configurations
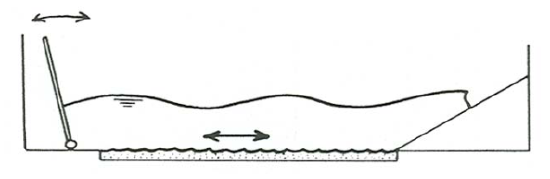
There are three ways to make oscillatory-flow bed configurations in the laboratory. One is to build a big long tank and make waves in it by putting a wave generator at one end and a wave absorber at the other end (Figure \(\PageIndex{1}\)). The generator does not need to be anything more than a flap hinged at the bottom and rocked back and forth in the direction of the tank axis at the desired period. This arrangement makes nice bed forms, but the trouble is that you are limited to short oscillation periods.
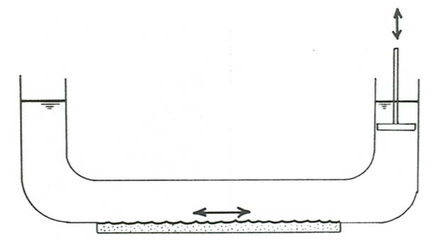
Another good way to make oscillatory-flow bed configurations is to build a horizontal closed duct that connects smoothly with reservoir tanks at both ends, fill the whole apparatus with water, and then put a piston in contact with the water surface in one of the reservoir tanks and oscillate it up and down at the desired period (Figure \(\PageIndex{2}\)). This allows you to work with much longer-period oscillations, but there is the practical problem that the apparatus has its own natural oscillation period, and if you try to make oscillations at a much different period you have to fight against what the duct wants to do, and that means large forces.
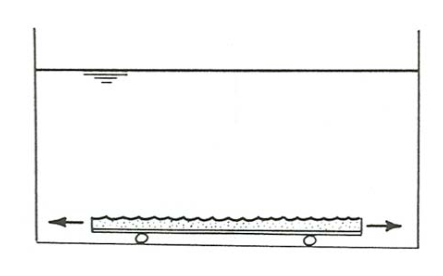
The third way should seem elegant and ingenious to you: place a sand-covered horizontal tray at the bottom of a large tank of water, and oscillate the tray back and forth underneath the water (Figure \(\PageIndex{3}\)). The problem is that the details of particle and fluid accelerations are subtly different from the other two devices, and it turns out that the bed configurations produced in this kind of apparatus do not correspond well with those produced in the other two kinds of apparatus.
Imagine making an exploratory series of runs in an oscillatory-flow duct of the kind shown in Figure \(\PageIndex{2}\) to obtain a general idea of the nature of oscillatory-flow bed configurations. Work at just one oscillation period, in the range from three to five seconds. Start at a low maximum oscillation velocity and increase it in steps. Figure \(\PageIndex{4}\) shows the sequence of bed configurations you would observe.
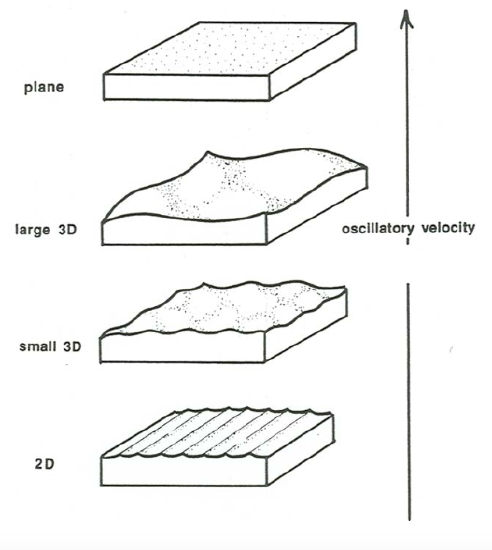
Once the movement threshold is reached, a pattern of extremely regular and straight-crested ripples develops on a previously planar bed. The ripples are symmetrical in cross section, with sharp crests and broad troughs. In striking contrast to unidirectional-flow bed configurations, the plan pattern is strikingly regular: ripple size varies little from ripple to ripple, and the ripples are straight and regular. At fairly low velocities the ripples are relatively small, withspacings of no more than several centimeters, but with increasing velocity the become larger and larger.
In a certain range of moderate velocities, the ripples become noticeably less regular and more three-dimensional, although they are still oriented dominantly transverse to the oscillatory flow. These three-dimensional ripples continue to grow in size with increasing velocity, until eventually they become flattened and are finally washed out to a planar bed. Therefore, just as in unidirectional flows, rugged bed configurations pass over into a stable plane-bed mode of transport with increasing velocity.
Oscillatory-flow bed configurations at longer oscillation periods are much less well studied, especially at high oscillatory velocities. Some comments on bed configurations produced under those conditions, which are very important in natural environments, are given in a later section.
Dimensional Analysis
Assume again, as we did earlier with unidirectional flow bed configurations, that the sediment is described well enough by its density \(\rho_{s}\) and average size \(D\). The oscillatory flow is specified by any two of the following three variables: oscillation period \(T\), orbital diameter \(d_{\text{o}}\) (the distance traveled by water particles during one-half of an oscillation), and maximum orbital velocity \(U_{m}\); I’ll use \(T\) and \(U_{m}\) here. As with unidirectional-flow bed configurations, we also need to include \(\rho\), \(\mu\), and \(\gamma^{\prime}\). The number of independent variables is seven, so we should expect a set of four equivalent dimensionless variables.
One dimensionless variable can again be the density ratio \(\rho_{s}/\rho\), and the other three have to include \(U_{m}\), \(T\), and \(D\) as well as \(\rho\), \(\mu\), and \(\gamma^{\prime}\). Adopting the same strategy as for unidirectional flow, we can form a dimensionless maximum oscillation velocity, a dimensionless oscillation period, and a dimensionless sediment size:
\(\left(\frac{\rho^{2}}{\mu \gamma^{\prime}}\right)^{1/3}U_{m}, \left(\frac {\gamma ^{\prime 2}}{\rho \mu} \right)^{1/3}T, \left(\frac{\gamma^{\prime} \rho}{\mu^{2}} \right)^{1/3}D\)
Then we can plot another three-dimensional graph to show the stability fields of oscillatory-flow bed phases, just as for unidirectional-flow bed phases (Figure \(\PageIndex{5}\)). Relationships are best revealed by looking at a series of velocity–period sections through the graph for various values of sediment size (Figure \(\PageIndex{5}\)). Figure \(\PageIndex{6}\) shows three such sections, one for very fine sands, \(0.1–0.2\) \(\mathrm{mm}\) (Figure \(\PageIndex{6}\)A), one for medium sands, \(0.3–0.4\) \(\mathrm{mm}\) (Figure \(\PageIndex{6}\)B), and one for coarse sands (\(0.5–0.6\) \(\mathrm{mm}\) (Figure \(\PageIndex{6}\)C). As with the graphs for unidirectional flows presented earlier, the axes are labeled with the \(\10^{\circ}\mathrm{C}\) values of velocity and period corresponding to the actual dimensionless variables. The data shown in Figure \(\PageIndex{6}\) are from laboratory experiments on oscillatory-flow bed configurations, made in both wave tanks and oscillatory-flow ducts, by several different investigators.
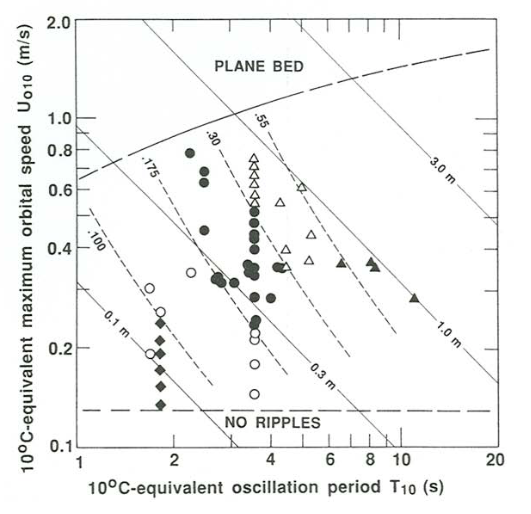
In each section in Figure \(\PageIndex{6}\), there is no movement at low velocities and a plane-bed mode of transport at high velocities. The intervening stability region for oscillation ripples narrows with decreasing oscillation period. As with ripples in unidirectional flows, there really are two different kinds of lower boundary of the stability field for oscillation ripples: one represents the threshold for sediment movement on a preexisting planar bed, and the other represents the minimum oscillation velocity needed to maintain the equilibrium of a preexisting ripple configuration. Existing data are not extensive enough to define the exact nature of these boundaries.
The most prominent feature of each of the sections in Figure \(\PageIndex{6}\) is the regular increase in ripple spacing from lower left to upper right, with increasing velocity and period. The contours of ripple spacing are close to being parallel to the lines of equal orbital diameter except near the transition to plane bed.
An important feature of the section for fine sands is a transition from extremely regular straight-crested ripples (which I will call two-dimensional ripples) at relatively low oscillation velocities to rather irregular ripples (which I will call three-dimensional ripples) with short and sinuous crest lines at relatively high oscillation velocities. The most three-dimensional bed configurations show only a weak tendency for flow-transverse orientation, and it is difficult or impossible to measure an average ripple spacing. In medium sands (Figure \(\PageIndex{6}\)B) the transition from two-dimensional ripples to three-dimensional ripples takes place at velocities closer to the transition to plane bed, and the tendency for three-dimensionality is not as marked as in fine sands.
Superimposed smaller ripples are prominent in the troughs and on the flanks of the larger ripples formed at long oscillation periods and high oscillation velocities in fine sands. These small superimposed ripples have spacings of about \(7\) \(\mathrm{cm}\), and they seem to dynamically related to ripples in unidirectional flows. The one-way flow during each half of the oscillation lasts long enough and transports enough sediment so that a pattern of current ripples becomes established in local areas on the bed. The flow in the other direction reverses the asymmetry of these small ripples but does not destroy them.
Experimental data are least abundant for long periods and high velocities, but preliminary data show the existence of three-dimensional rounded bed forms with spacings of well over a meter in fine sands under these conditions. In contrast to the smaller two-dimensional ripples, these large ripples are not static but show a tendency to change their shape and shift their position with time, even after the bed configuration has stopped changing on the average.
In coarse sands (Figure \(\PageIndex{6}\)C), no experiments have been made at the longest periods and highest velocities, but evidence from observations in modern shallow marine environments, and also from the ancient sedimentary record, suggests that ripples in coarse sands are two-dimensional over the entire range of periods and velocities characteristic of natural flow environments.
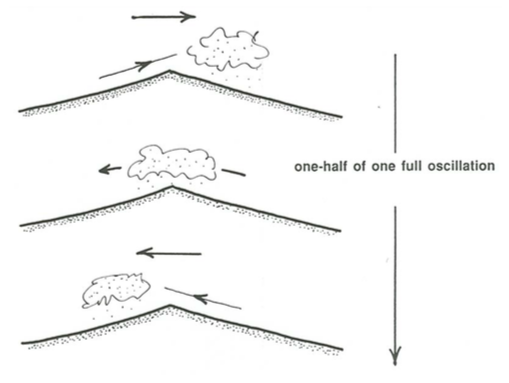
The flow over oscillation ripples is characteristic (Figure \(\PageIndex{7}\)). During half of the oscillation cycle, the flow separates over the sharp crest of the ripple, putting abundant suspended sediment in suspension in the separation vortex over the downflow side. As the flow reverses, the vortex is abruptly carried over the crest of the ripple and deposits its suspended sediment. Flow separation is then rapidly reestablished on the other side of the ripple, and a new vortex develops. For this reason, these ripples have been called vortex ripples.
Purely oscillatory flows that involve a discrete or continuous range of oscillatory components with different directions, periods, and velocities must be common in the shallow ocean. For example, when a storm passes a given area, strong winds tend to blow from different directions at different times. Some time is needed for the sea state to adjust itself to the changing wind directions, and during those times the sea state is complicated, with superimposed waves running in different directions. The nature of bed configurations under even simple combinations of two different wave trains is little known. Much more observational work needs to be done on this topic.
Combined-Flow Bed Configurations
So far we have considered only the two “end-member cases” of flows that make bed configurations. Even aside from the importance of time-varying unidirectional and oscillatory flows, and of purely oscillatory flows with more than just one oscillatory component, there is an entire range of combined flows that generate distinctive bed configurations. Observations in the natural environment are scarce, and systematic laboratory work (Arnott and Southard, 1990; Yokokawa, 1995; Dumas et al., 2005) has so far explored only a small part of the wide range of relevant conditions. This section is therefore necessarily shorter than the previous sections. Up to now, systematic observations have been made only for combined flows in which a single oscillatory component is superimposed on a current flowing with the same orientation as the oscillation. There is therefore still a major gap in our knowledge of combined-flow bed configurations.
Figure \(\PageIndex{8}\) is an inadequate attempt to provide a conceptual framework for thinking about combined-flow bed configurations. Ideally we would like to be able to plot observational data on combined-flow bed configurations on a graph with axes representing the four important independent variables: oscillatory velocity, unidirectional velocity, oscillation period, and sediment size. Unfortunately it is impossible for human beings to visualize four-dimensional graphs. A substitute approach (Figure \(\PageIndex{8}\)) is to imagine one or the other of two equivalent kinds of graphs:
- a continuous series of three-dimensional graphs with the two velocity components and sediment size along the axes, one such graph for each value of oscillation period; or
- a continuous series of three-dimensional graphs with the two velocity components and oscillation period along the axes, one such graph for each value of sediment size.
Systematic laboratory experiments on combined-flow configurations have been carried out by Arnott and Southard and, more recently, covering wider range of flow and sediment conditions, by Dumas et al. (2005). The experiments by Dumas et al. (2005) were done in large oscillatory-flow ducts with oscillation periods ranging from about \(8\) \(\mathrm{s}\) to \(11\) \(\mathrm{s}\) (scaled to \(10^{\circ}\mathrm{C}\) water temperature), with well-sorted sediments ranging in size from \(0.10\) to \(0.23\) \(\mathrm{mm}\) (scaled to \(10^{\circ}\mathrm{C}\) water temperature). Figure \(\PageIndex{9}\) shows three phase diagrams, for three combinations of oscillation period and sediment size, showing data points and phase boundaries. The boundaries within the field for ripples are gradual rather than abrupt. Bear in mind, when looking at these diagrams, that they are still an extremely “thin” representation of the graphic framework shown in Figure \(\PageIndex{8}\).

Here are some of the features of Figure \(\PageIndex{9}\). At combinations of low oscillatory velocities and low unidirectional velocities, there is no sediment movement. At combinations of high oscillatory velocities and high unidirectional velocities, a planar bed with strong sediment movement is the stable bed configuration. Note that when even a small unidirectional component is present, the oscillatory velocity for the transition from ripples to plane bed is substantially lower than in purely oscillatory flow.
In the lower part of the region of ripple stability, the ripples are relatively small. Only a small unidirectional component is needed to make the small ripples fairly asymmetrical. Except when the unidirectional component is very weak, small combined-flow ripples are not greatly different in geometry from ripples in purely unidirectional flow.
In the upper part of the region of ripple stability, the ripples are relatively large. Only a small unidirectional flow component is needed to make the large three-dimensional oscillatory-flow bed forms produced at these oscillation periods and sediment sizes noticeably asymmetrical. For relatively large oscillatory velocities, especially in the finer sand size, the bed forms acquire a three-dimensional hummocky appearance; this region is shown by the shading in Figures \(\PageIndex{9}\)A, B, and C; it is a feature that seems to become superimposed on the symmetrical to symmetrical large combined-flow ripples under those values of the velocity components.
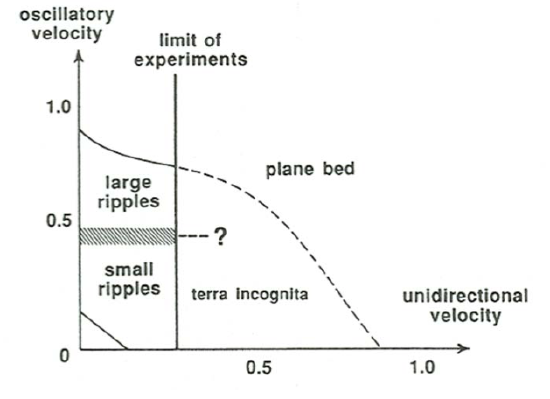
At unidirectional velocities greater than are shown in this graph, the field for large combined-flow ripples must pinch out, because small ripples are known to be the only stable bed configuration in purely unidirectional flows in these fine sand sizes. Figure \(\PageIndex{10}\) shows a speculative extrapolation of Figure \(\PageIndex{9}\) to higher unidirectional velocities. The effect of an increasingly strong oscillatory velocity component on unidirectional-flow dunes in medium and coarse sands is an intriguing problem for which no experimental data are yet available.
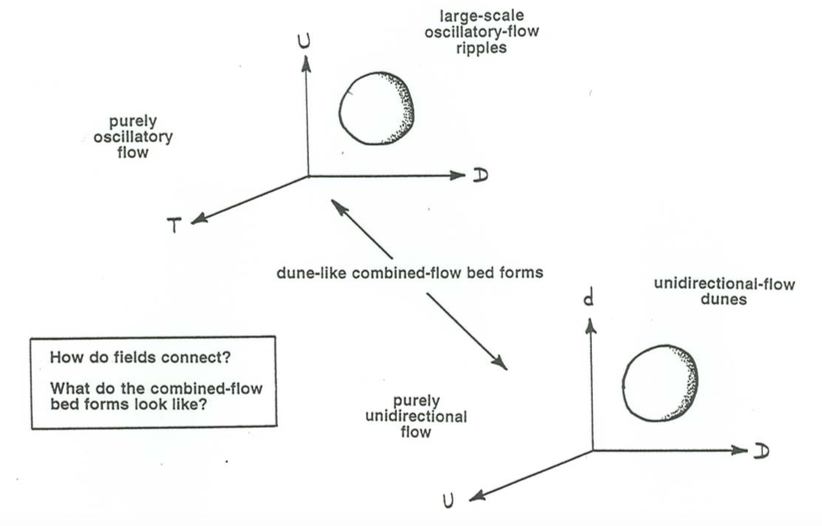
When the oscillation period is large, medium to high oscillation velocities produce large symmetrical ripples. Even a slight unidirectional component is known (e.g., Arnott and Southard, 1990; Dumas et al., 2005) to make these large ripples noticeably asymmetrical, to the point where they are not greatly different in geometry and internal stratification from unidirectional-flow dunes. That leads to an important question: what do the large-scale bed forms in the intermediate range of flow conditions and sediment sizes look like? There has been almost no systematic study of such bed forms, and yet deductively it seems that they should be important, and that a lot of the cross stratification we see in the ancient sedimentary record must have been produced under such conditions. Figure \(\PageIndex{11}\).


