3.6: Heat Budget at Earth's Surface
- Page ID
- 9545
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)So far, you examined the heat budget for a volume of air, where the volume was fixed (Eulerian) or moving (Lagrangian). Net imbalances of heat flux caused warming or cooling of air in the volume.
But what happens at the Earth’s surface, which is infinitesimally thin and thus has zero volume? No heat can be stored in this layer. Hence, the sum of all incoming and outgoing heat fluxes must exactly balance. The net flux at the surface must be zero.
3.6.1. Surface Heat-flux Balance
Recall that fluxes are defined to be positive for heat moving upward, regardless of whether these fluxes are in the soil or the atmosphere.
Relevant fluxes at the surface include:
F* = net radiation between sfc. & atmos. (Chapter 2)
FH = effective surface turbulent heat flux (the sensible Heat flux)
FE = effective surface latent heat flux caused by Evaporation or condensation (dew formation)
FG = molecular heat conduction to/from deeper below the surface (e.g., Ground, oceans).
The surface balance for dynamic heat-fluxes (in units of W m–2) is:
\(\ \begin{align} 0=\mathbb{F}^{*}+\mathbb{F}_{H}+\mathbb{F}_{E}-\mathbb{F}_{G}\tag{3.52}\end{align}\)
If you divide by ρair·Cp to get the balance in kinematic form (in units of K m s–1), the result is:
\(\ \begin{align}0=F^{*}+F_{H}+F_{E}-F_{G}\tag{3.53}\end{align}\)
The first 3 terms on the right are fluxes between the surface and the air above. The last term is between the surface and the Earth below (hence the – sign).
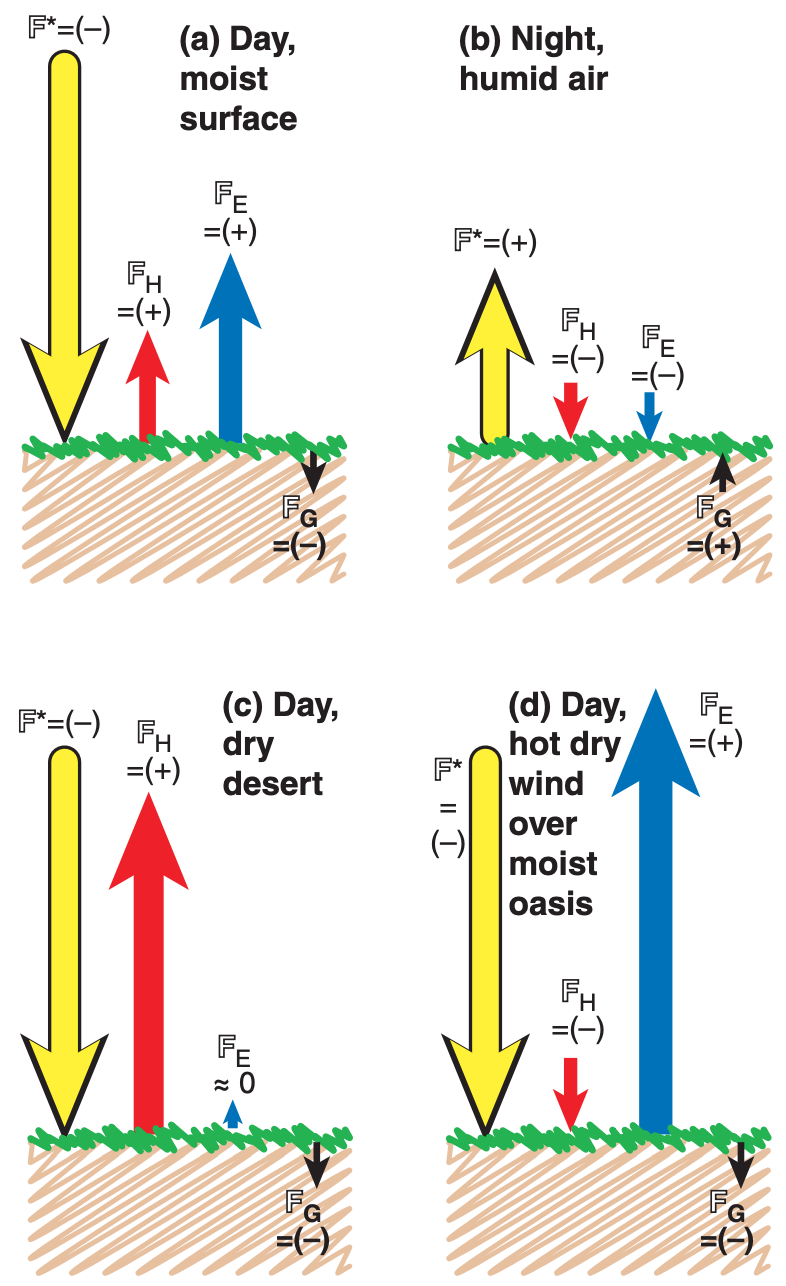
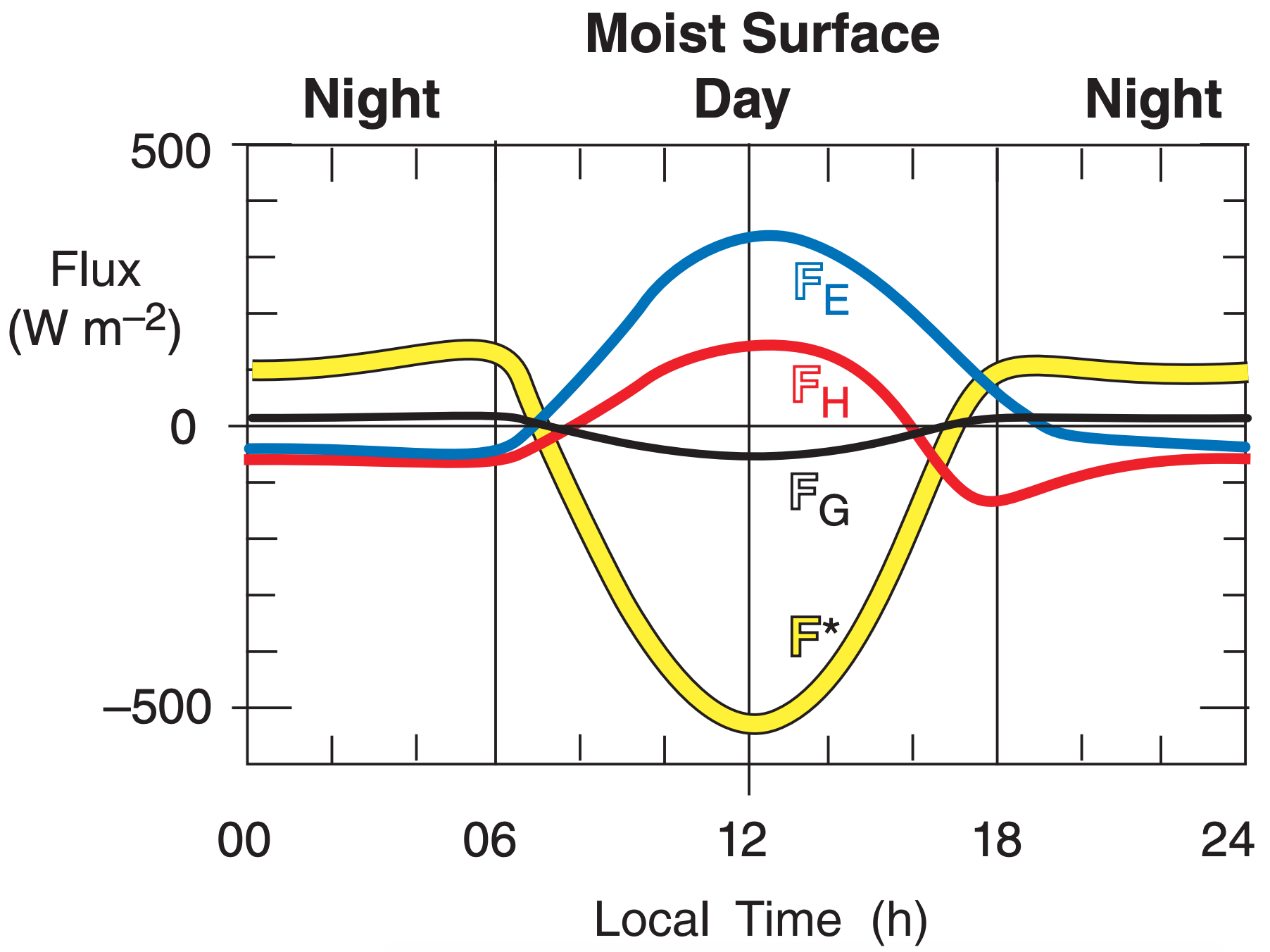
Examples of these fluxes and their signs are sketched in Figure 3.9 for different surfaces and for day vs. night. For an irrigated lawn or crop, the typical diurnal cycle (daily evolution) of surface fluxes is sketched in Figure 3.10. Essentially, net radiation F* is an external forcing that drives the other fluxes.
A crude, first-order approximation for dynamic heat flux down into the soil is
\(\ \begin{align}\mathbb{F}_{G} \approx X \cdot \mathbb{F}^{*}\tag{3.54}\end{align}\)
with a corresponding kinematic heat flux of:
\(\ \begin{align}F_{G} \approx X \cdot F^{*}\tag{3.55}\end{align}\)
with factor X = (0.1, 0.5) for (daytime, nighttime).
There are different options for estimating the other terms in eqs. (3.52 or 3.53). For effective surface sensible heat flux you can use the bulk-transfer relationships already discussed (eqs. 3.34 to 3.37). For latent heat flux at the surface, similar bulk-transfer equations will be given in the Water-Vapor chapter. Another option for estimating latent and sensible heat fluxes at the surface is to utilize the Bowen-ratio, described next.
3.6.2. The Bowen Ratio
Define a Bowen ratio, B, as surface sensible-heat flux divided by surface latent-heat flux:
\(\ \begin{align}B=\frac{\mathbb{F}_{H}}{\mathbb{F}_{E}}=\frac{F_{H}}{F_{E}}\tag{3.56}\end{align}\)
Typical values are: 10 for arid locations, 5 for semiarid locations, 0.5 over drier savanna, 0.2 over moist farmland, and 0.1 over oceans and lakes.
In the atmospheric surface layer (the bottom 10 to 25 m of the troposphere), surface effective sensible heat flux depends on ∆θ/∆z — the potential-temperature gradient. Namely, FH = –KH·∆θ/∆z , where KH is an eddy diffusivity for heat (see the Atmos. Boundary Layer chapter), z is height above ground, and the negative sign says that the heat flux flows down the local gradient (from hot toward cold air).
Sample Application
If the net radiation is –800 W·m–2 at the surface over a desert, then find sensible, latent, and ground fluxes.
Find the Answer
Given: F* = –800 W·m–2 B = 10 for arid regions
Find: FH, FE and FG = ? W·m–2
Because negative F* implies daytime, use X = 0.1 in eq. (3.54): FG = 0.1· F* = 0.1· (–800 W·m–2 ) = –80 W·m–2
Eqs. (3.52 & 3.56) can be manipulated to give:
FE = (FG – F*) / (1 + B)
FH = B·(FG – F*) / (1 + B)
Thus,
FE= (–80 + 800 W·m–2) / (1 + 10) = 65.5 W·m–2
FH= 10·(–80 + 800 W·m–2) / (1 + 10) = 654.5 W·m–2
Check: Physics and units are reasonable. Also, we should confirm that the result gives a balanced energy budget. So apply eq. (3.52):
0 = F* + FH + FE – FG ???
0 = –800 + 654.5 + 65.5 + 80 W·m–2 True.
Exposition: Although typical values for the Bowen ratio were given on the previous page, the actual value for any given type of surface depends on so many factors that it is virtually useless when trying to use the Bowen ratio method to predict surface fluxes. However, the field-measurement approach shown in the figure below and in eqs. (3.58 - 3.63) does not require an a-priori Bowen ratio estimate. Hence, this field approach is quite accurate for measuring surface fluxes, except near sunrise and sunset.
An analogous expression for effective surface moisture flux is Fwater = –KE·∆r/∆z , where mixing ratio r is defined in the next chapter as mass of water vapor contained in each kg of dry air. If you approximate the eddy diffusivity for moisture, KE, as equaling that for heat and if the vertical gradients are measured across the same air layer ∆z, then you can write the Bowen ratio as:
\(\ \begin{align}B=\gamma \cdot \frac{\Delta \theta}{\Delta r}\tag{3.57}\end{align}\)
for a psychrometric constant defined as γ = Cp/Lv = 0.4 (gwater vapor/kgair)·K–1.
Eq. (3.57) is appealing to use in field work because the difficult-to-measure fluxes have been replaced by easy-to-measure mean-temperature and humidity differences. Namely, if you erect a short tower in the surface layer and deploy thermometers at two different heights and mount hygrometers (for measuring humidity) at the same two heights (Figure 3.11), then you can compute B. Don’t forget to convert the temperature difference to potential-temperature difference: ∆θ = T2 – T1 + (0.0098 K m–1)·(z2 – z1).
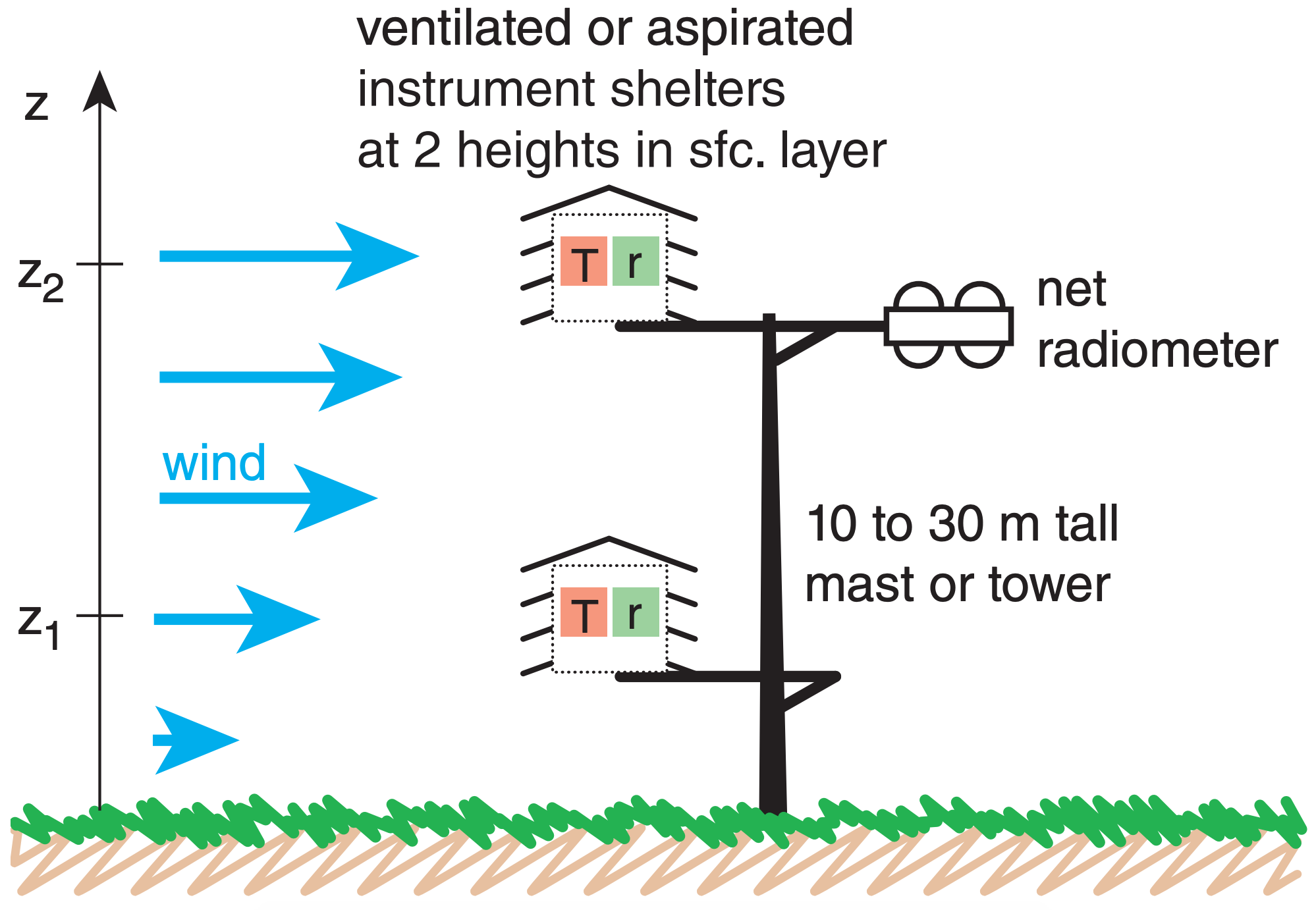
With a bit of algebra you can combine eqs. (3.57, 3.56, 3.54, and 3.52) to yield effective surface sensible heat flux in dynamic units (W m–2) as a function of net radiation:
\(\ \begin{align} \mathbb{F}_{H}=\frac{-0.9 \cdot \mathbb{F}^{*}}{\frac{\Delta r}{\gamma \cdot \Delta \theta}+1}\tag{3.58}\end{align}\)
or kinematic units (K m s–1):
\(\ \begin{align} F_{H}=\frac{-0.9 \cdot F^{*}}{\frac{\Delta r}{\gamma \cdot \Delta \theta}+1}\tag{3.59}\end{align}\)
A bit more algebra yields the latent heat flux (W m–2) caused by movement of water vapor to or from the surface:
\(\ \begin{align}\mathbb{F}_{E}=\frac{-0.9 \cdot \mathbb{F}^{*}}{\frac{\gamma \cdot \Delta \theta}{\Delta r}+1}\tag{3.60}\end{align}\)
or in kinematic units (K m s–1):
\(\ \begin{align}F_{E}=\frac{-0.9 \cdot F^{*}}{\frac{\gamma \cdot \Delta \theta}{\Delta r}+1}\tag{3.61}\end{align}\)
The next chapter shows how to convert latent heatflux values into waver-vapor fluxes.
If you have found sensible heat flux from eqs. (3.58 or 3.59), then the latent heat flux is easily found from:
\(\ \begin{align}\mathbb{F}_{E}=-0.9 \cdot \mathbb{F}^{*}-\mathbb{F}_{H}\tag{3.62}\end{align}\)
or
\(\ \begin{align}F_{E}=-0.9 \cdot F^{*}-F_{H}\tag{3.63}\end{align}\)
Sample Application
A Bowen-ratio field site observes the following:
| index | z (m) | T (°C) | r (gvapor/kgair) |
| 2 | 15 | 16 | 7 |
| 1 | 1 | 20 | 12 |
with, F* = –650 W·m–2 . Find all surface fluxes.
Find the Answer
Given: info above.
Find: surface dynamic fluxes (W·m–2) FE , FH , FG = ?
First step is to find ∆θ:
∆θ = T2 – T1 + (0.0098 K m–1)·(z2 – z1) = 16 K – 20 K + (0.0098 K m–1)·(15m – 1m) = –4 K + 0.137 K = –3.86 K
Apply eq. (3.58)
\(\mathbb{F}_{H}=\frac{-0.9 \cdot\left(-650 \mathrm{W} \cdot \mathrm{m}^{-2}\right)}{\frac{\left(-5 \mathrm{g}_{\mathrm{vap}} / \mathrm{kg}_{\mathrm{air}}\right)}{\left[0.4\left(\mathrm{g}_{\mathrm{vap}} / \mathrm{kg}_{\mathrm{air}}\right) \cdot \mathrm{K}^{-1}\right] \cdot(-3.86 \mathrm{K})}+1}\)
FH = 138 W·m–2.
Next, apply eq. (3.62):
FE = –0.9·F* – FH = –0.9·(–650 W·m–2) – 138. W·m–2 = 447 W·m–2
Finally, apply eq. (3.54): FG = 0.1·F* = –65 W m–2.
Check: Physics & units are reasonable. Also, all the flux terms sum to zero, verifying the balance.
Exposition: The resulting Bowen ratio is B = 138/447 = 0.31, which suggests the site is irrigated farmland.


