7: Geologic Time
- Page ID
- 22419
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
The Earth is 4.566 billion years old. [1]
Knowing this is not simply some esoteric piece of trivia. Instead, the discovery of Earth’s great antiquity is the crowning achievement of the geosciences.
Why does it matter? If Earth were only a mere 10,000 years old, then human civilization would represent a significantly large portion of Earth’s history. Instead, in the context of an Earth that is 4.566 billion years old, modern human civilization hardly registers at all, amounting to a trivially small sliver (~0.0003%) of Earth’s history. Understanding geological time is central to properly understanding our place in nature and history.
If you think about large numbers and try to comprehend the difference in the amounts, say the difference between 50,000 and 50,000,000, you have no frame of reference to visualize the difference. This is the difficulty in trying to understand the vastness of geological time—which is measured by millions and billions of years. Essayist John McPhee coined the phrase “deep time” in his 1981 book, Basin and Range. Deep time is truly staggering, a view captured eloquently in a recent essay in the New York Times by Peter Brannen.
Have a look at the image below (or download this PDF file), which illustrates 5,000 individual zeros.
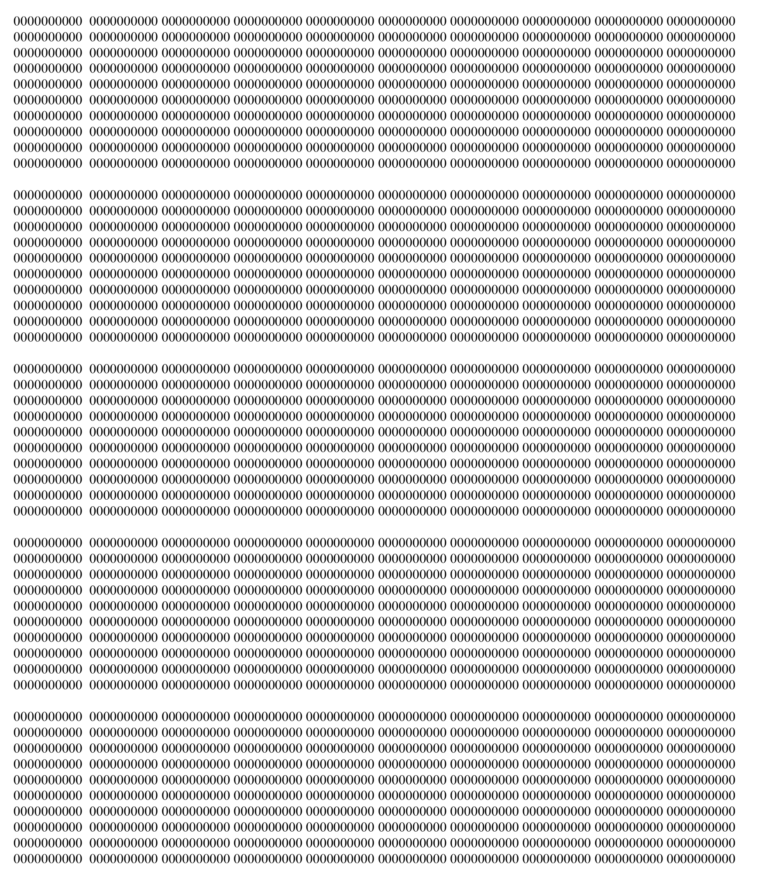
How many copies of this image (or sheets of paper) would you need to print to reach a total of a million zeros?
\[1,000,000 / 5,000 = 200 \text{ sheets of paper}\nonumber\]
Now, how many copies would you need to print to reach one-billion zeros?
\[1,000,000,000 / 5,000 = 200,000 \text{ sheets of paper}\nonumber\]
The Earth is 4, 566, 000, 000 years old. How many copies would you need to print to cover the age of the Earth with each year represented by 1 zero?
\[4,566,000,000 / 5000 = 913,200 \text{ sheets of paper}\nonumber\]
Copy paper is bundled by 500 sheets. This is called a ream of paper. How many reams of paper would you need to replicate the age of the Earth?
\[913,200 / 500 = 1,826 \text{ reams of paper}\nonumber\]
Typically there are 6 reams of paper per box, that makes 304 boxes of copy paper just to print out the number of zeros in the age of the Earth.
That is a long time...