8.3.1: Introduction

On high energy coasts, long-term (years to decades) shoreline changes are predominantly due to human-induced longshore effects. Cross-shore movement of sediment typically occurs on short timescales (days) and has little direct influence on the longer term changes in beach position, unless material is permanently lost from or introduced to the system. Possible sediment sinks are offshore canyons from where any deposited sediment cannot return, the hinterland (through aeolian transport) or sand mining from the beaches (for instance for construction purposes). Examples of sediment sources are dredge disposal or sediment input from rivers. Often however, longshore effects are the most important cause of shoreline changes on the longer timescales. Coastal change due to longshore processes will be the focus in the following.

Note

Please note that the coastline is aligned with the \(x\)-axis in the remainder of this chapter. This is different from the other parts of the lecture notes.

Figure 8.6: Longshore transport continuity.

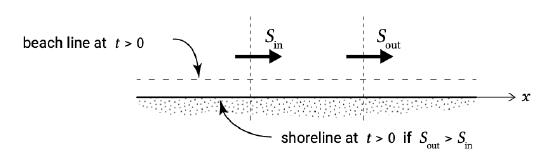
The long-term coastline changes are governed by net, yearly-averaged longshore sediment transport rates averaged over several wave conditions with varying magnitudes and directions. In computations this is taken into account by a schematised wave climate with a limited number of conditions (as discussed in Sect. 8.2.5). The very presence of sediment transport does not lead to either erosion or deposition. Indeed, if one considers a portion of beach as sketched in plan in Fig. 8.6, the coastline will remain stable as long as \(S_{\text{in}}\) in is equal to \(S_{\text{out}}\). On the other hand if \(S_{\text{out}}\) is greater than \(S_{\text{in}}\) (\(S\) increases as we move along the beach, \(\tfrac{dS}{dx} > 0\), or sediment transport divergence) material must be eroded in the area under consideration in order to maintain a sediment mass balance. The coastal profile including the shoreline will recede. The shoreline will move seawards (accretion) if the longshore sediment transport decreases in the transport direction (\(\tfrac{dS}{dx} < 0\) or sediment transport convergence). A general form of the sediment mass balance was given in Eq. 1.5.2.1.

There are different ways to solve coastal changes from a sediment balance (Eq. 1.1):

• In complex morphological computer models (such as Delft3D), the sediment balance is computed for each cell in a fine grid that covers the area of interest. At every time step a field of sediment transport rates is computed, based on the relevant hydrodynamic parameters. Subsequently, the bed levels are updated for every grid based on sediment continuity. Such detailed morphological models are generally used in complex areas or in complex applications in which cross-shore and longshore transports are important. They are relatively expensive to run and require skilled people to interpret the computational results. At Delft University of Technology, students will be taught to use these models in Coastal Dynamics II (CIE4309);
• If wave-induced longshore transport is the dominant mechanism we can simplify the problem. The coarsest schematization is made by the single line or one-line theory, in which the behaviour of the coast is mapped onto a single line, the coastline. In this approach it is assumed that the shape of the coastal profile itself does not change over the considered period of time or along the coast. The coast can thus be schematised as one single line, which moves sea-ward (accretion) or landward (erosion) depending on the sediment balance. This is what we (implicitly) assumed in Fig. 8.6. The single line theory is discussed in Sect. 8.3.2 and analytical solutions in Sect. 8.3.3. It gives a valuable insight into the principles of coastline dynamics;
• Multiple (two and more) line theories (Sect. 8.3.4) are based on the same principle, but now the coastal (cross-shore) profile is schematized into a number of sections (depth zones), which can each be represented by one line in the along-shore direction. This is a method to account for the fact that different depth zones respond differently to changing sediment budgets.