9.7: Measuring Earthquakes
- Page ID
- 8570
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Seismographs
People feel approximately 1 million earthquakes a year, usually when they are close to the source and the earthquake registers at least moment magnitude 2.5. Major earthquakes of moment magnitude 7.0 and higher are extremely rare. The U. S. Geological Survey (USGS) Earthquakes Hazards Program real-time map shows the location and magnitude of recent earthquakes around the world.
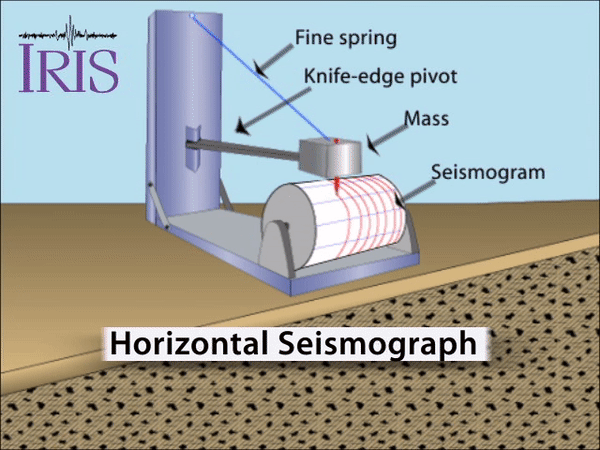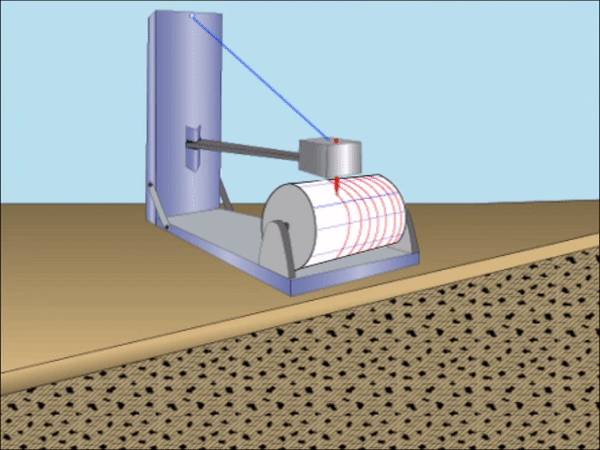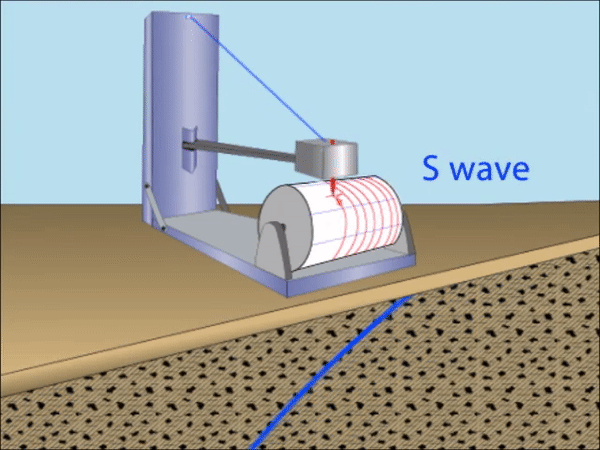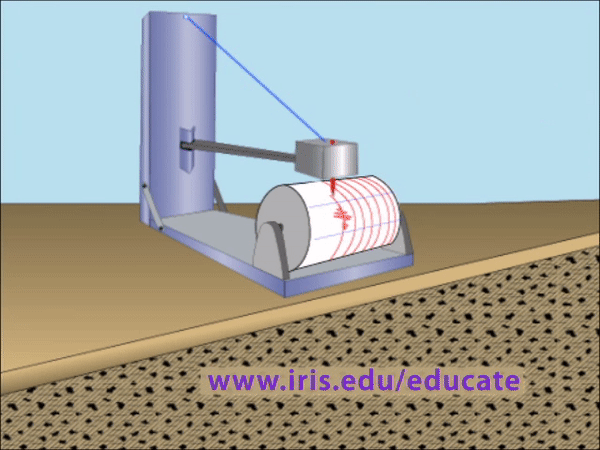
To accurately study seismic waves, geologists use seismographs that can measure even the slightest ground vibrations. Early 20th-century seismograms use a weighted pen (pendulum) suspended by a long spring above a recording device fixed solidly to the ground. The recording device is a rotating drum mounted with a continuous strip of paper. During an earthquake, the suspended pen stays motionless and records ground movement on the paper strip. The resulting graph a seismogram. Digital versions use magnets, wire coils, electrical sensors, and digital signals instead of mechanical pens, springs, drums, and paper. A seismograph array is multiple seismographs configured to measure vibrations in three directions: north-south (x-axis), east-west (y-axis), and up-down (z-axis).
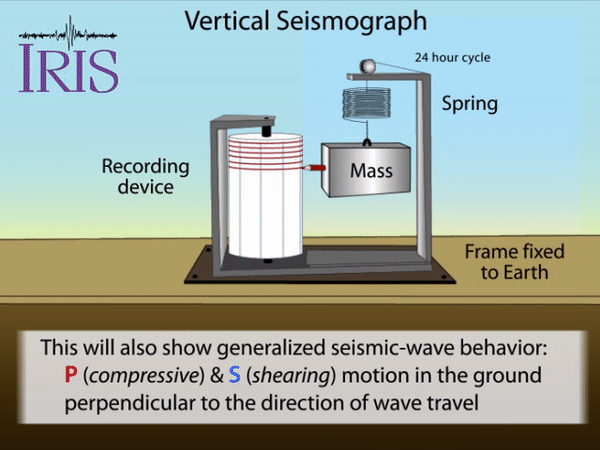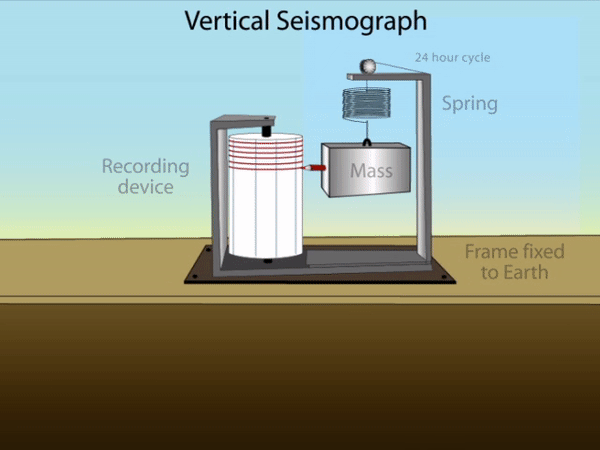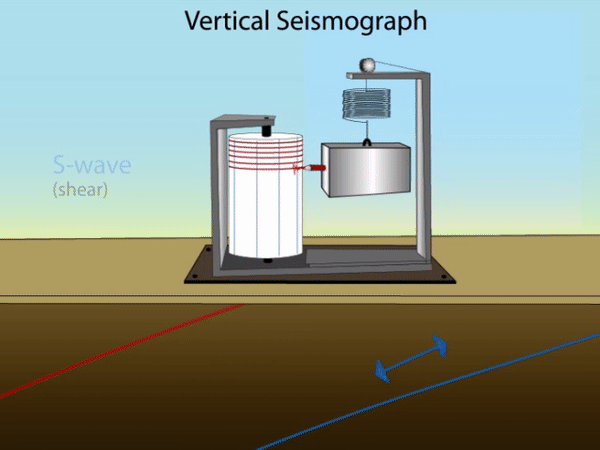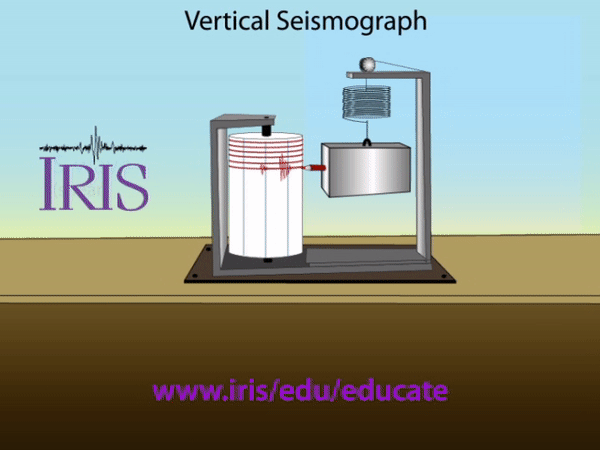
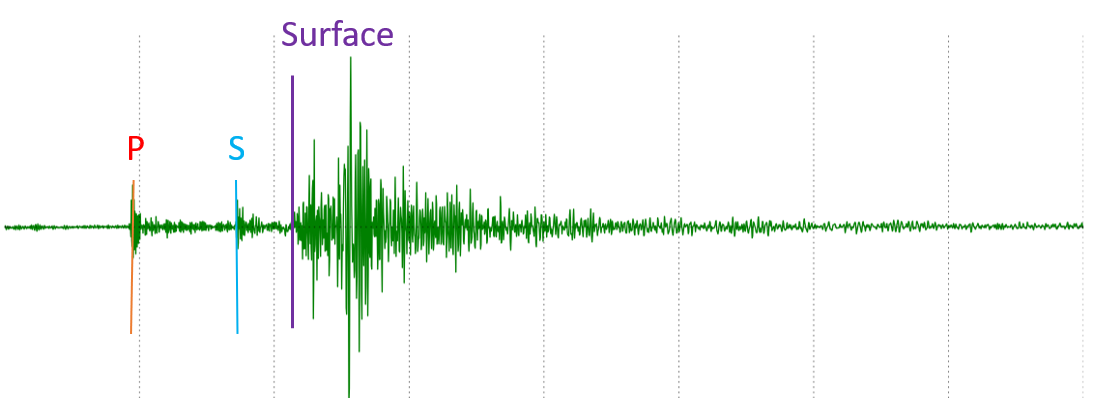
To pinpoint the location of an earthquake epicenter, seismologists use the differences in arrival times of the P, S, and surface waves. After an earthquake, P waves will appear first on a seismogram, followed by S waves, and finally surface waves, which have the largest amplitude. It is important to note that surface waves lose energy quickly, so they are not measurable at great distances from the epicenter. These time differences determine the distance but not the direction of the epicenter. By using wave arrival times recorded on seismographs at multiple stations, seismologists can apply triangulation to pinpoint the location of the epicenter of an earthquake. At least three seismograph stations are needed for triangulation. The distance from each station to the epicenter is plotted as the radius of a circle. The epicenter is demarked where the circles intersect. This method also works in 3D, using multi-axis seismographs and sphere radii to calculate the underground depth of the focus.
This video shows the method of triangulation to locate the epicenter of an earthquake.
Seismograph Network
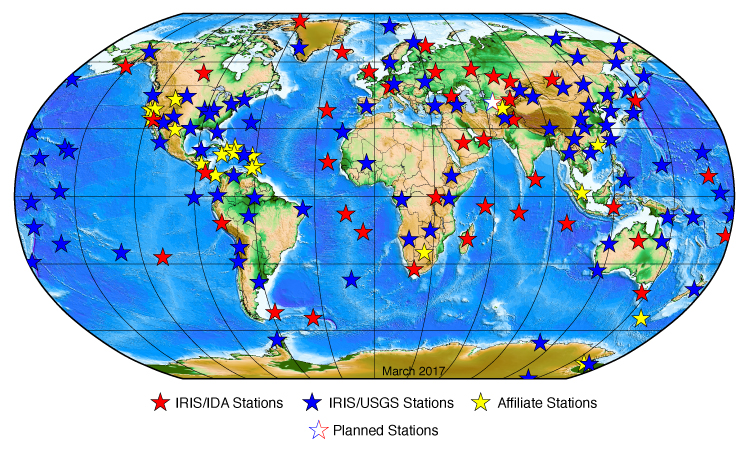
The International Registry of Seismograph Stations lists more than 20,000 seismographs on the planet. By comparing data from multiple seismographs, scientists can map the properties of the inside of the Earth, detect detonations of large explosive devices, and predict tsunamis. The Global Seismic Network, a worldwide set of linked seismographs that electronically distribute real-time data, includes more than 150 stations that meet specific design and precision standards. The USArray is a network of hundreds of permanent and transportable seismographs in the United States that are used to map the subsurface activity of earthquakes (see video).
Along with monitoring for earthquakes and related hazards, the Global Seismograph Network helps detect nuclear weapons testing, which is monitored by the Comprehensive Nuclear Test Ban Treaty Organization. Most recently, seismographs have been used to determine nuclear weapons testing by North Korea.
Nepal Earthquake M7.9 Ground Motion Visualization
Seismic Tomography
Very much like a CT (Computed Tomography) scan uses X-rays at different angles to image the inside of a body, seismic tomography uses seismic rays from thousands of earthquakes that occur each year, passing at all angles through masses of rock, to generate images of internal Earth structures.
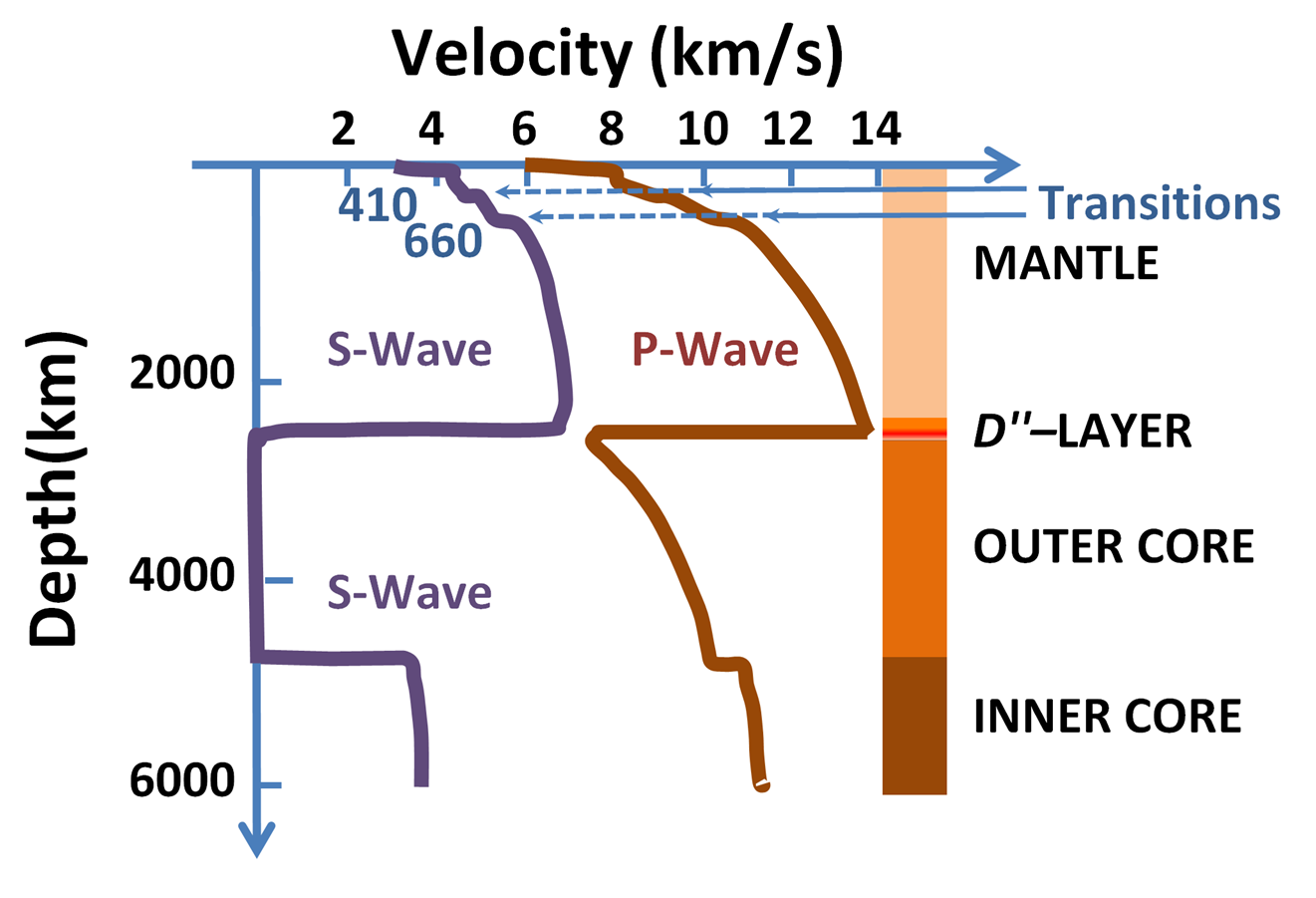 CC BY-SA 3.0 or GFDL], via Wikimedia Commons" width="516px" height="353px" src="/@api/deki/files/7861/Speeds_of_seismic_waves.png">
CC BY-SA 3.0 or GFDL], via Wikimedia Commons" width="516px" height="353px" src="/@api/deki/files/7861/Speeds_of_seismic_waves.png">Using the assumption that the earth consists of homogenous layers, geologists developed a model of expected properties of earth materials at every depth within the earth called the PREM (Preliminary Reference Earth Model). These properties include seismic wave transmission velocity, which is dependent on rock density and elasticity. In the mantle, temperature differences affect rock density. Cooler rocks have a higher density and therefore transmit seismic waves faster. Warmer rocks have a lower density and transmit earthquake waves slower. When the arrival times of seismic rays at individual seismic stations are compared to arrival times predicted by PREM, differences are called seismic anomalies and can be measured for bodies of rock within the earth from seismic rays passing through them at stations of the seismic network. Because seismic rays travel at all angles from lots of earthquakes and arrive at lots of stations of the seismic network, like CT scans of the body, variations in the properties of the rock bodies allow 3D images to be constructed of the rock bodies through which the rays passed. Seismologists are thus able to construct 3D images of the interior of the Earth.
For example, seismologists have mapped the Farallon Plate, a tectonic plate that subducted beneath North America during the last several million years, and the Yellowstone magma chamber, which is a product of the Yellowstone hot spot under the North American continent. Peculiarities of the Farallon Plate subduction are thought to be responsible for many features of western North America including the Rocky Mountains (See chapter 8).
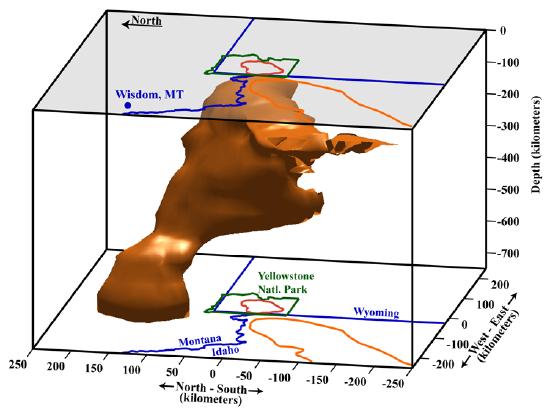
![By NASA [Public domain], via Wikimedia Commons Tomographic image of the Farallon plate in the mantle below North America.](https://geo.libretexts.org/@api/deki/files/7865/Farallon_Plate-e1490562202658.jpg?revision=1&size=bestfit&width=366&height=337)
Earthquake Magnitude and Intensity
Richter Scale
Magnitude is the measure of the energy released by an earthquake. The Richter scale (ML), the first and most well-known magnitude scale, was developed by Charles F. Richter (1900-1985) at the California Institute of Technology. This was the magnitude scale used historically by early seismologists. Used by early seismologists, Richter magnitude (ML) is determined from the maximum amplitude of the pen tracing on the seismogram recording. Adjustments for epicenter distance from the seismograph are made using the arrival-time differences of S and P waves [7].
The Richter Scale is logarithmic, based on powers of 10. This means an increase of one Richter unit represents a 10-fold increase in seismic-wave amplitude or in other words, a magnitude 6 earthquake shakes the ground 10 times more than a magnitude 5. However, the actual energy released for each magnitude unit is 32 times greater, which means a magnitude 6 earthquake releases 32 times more energy than a magnitude 5.
The Richter Scale was developed for earthquakes in Southern California, using local seismographs. It has limited applications for larger distances and very large earthquakes. Therefore, most agencies no longer use the Richter Scale. The moment magnitude (MW), which is measured using seismic arrays and generates values comparable to the Richter Scale, is more accurate for measuring earthquakes across the Earth, including large earthquakes, although they require more time to calculate. News media often report Richter magnitudes right after an earthquake occurs even though scientific calculations now use moment magnitudes.
Moment Magnitude Scale
The Moment Magnitude scale depicts the absolute size of earthquakes, comparing information from multiple locations and using a measurement of actual energy released calculated from the cross-sectional area of rupture, amount of slippage, and the rigidity of the rocks. Because each earthquake occurs in a unique geologic setting and the rupture area is often hard to measure, estimates of moment magnitude can take days or even months to calculate.
Like the Richter Scale, the moment magnitude scale is logarithmic. The magnitude values of the two scales are approximately equal, except for very large earthquakes. Both scales are used for reporting earthquake magnitude. The Richter Scale provides a quick magnitude estimate immediately following the quake and thus, is usually reported in news accounts. Moment magnitude calculations take much longer but are more accurate and thus, more useful for scientific analysis.
| Intensity | Shaking | Description/Damage |
|---|---|---|
| I | Not felt | Not felt except by a very few under especially favorable conditions. |
| II | Weak | Felt only by a few persons at rest, especially on upper floors of buildings. |
| III | Weak | Felt quite noticeably by persons indoors, especially on upper floors of buildings. Many people do not recognize it as an earthquake. Standing motor cars may rock slightly. Vibrations similar to the passing of a truck. Duration estimated. |
| IV | Light | Felt indoors by many, outdoors by few during the day. At night, some awakened. Dishes, windows, doors disturbed; walls make cracking sound. Sensation like heavy truck striking building. Standing motor cars rocked noticeably. |
| V | Moderate | Felt by nearly everyone; many awakened. Some dishes, windows broken. Unstable objects overturned. Pendulum clocks may stop. |
| VI | Strong | Felt by all, many frightened. Some heavy furniture moved; a few instances of fallen plaster. Damage slight. |
| VII | Very strong | Damage negligible in buildings of good design and construction; slight to moderate in well-built ordinary structures; considerable damage in poorly built or badly designed structures; some chimneys broken. |
| VIII | Severe | Damage slight in specially designed structures; considerable damage in ordinary substantial buildings with partial collapse. Damage great in poorly built structures. Fall of chimneys, factory stacks, columns, monuments, walls. Heavy furniture overturned. |
| IX | Violent | Damage considerable in specially designed structures; well-designed frame structures thrown out of plumb. Damage great in substantial buildings, with partial collapse. Buildings shifted off foundations. |
| X | Extreme | Some well-built wooden structures destroyed; most masonry and frame structures destroyed with foundations. Rails bent. |
Table. Abridged Mercalli Scale from USGS General Interest Publication 1989-288-913.
ShakeMaps
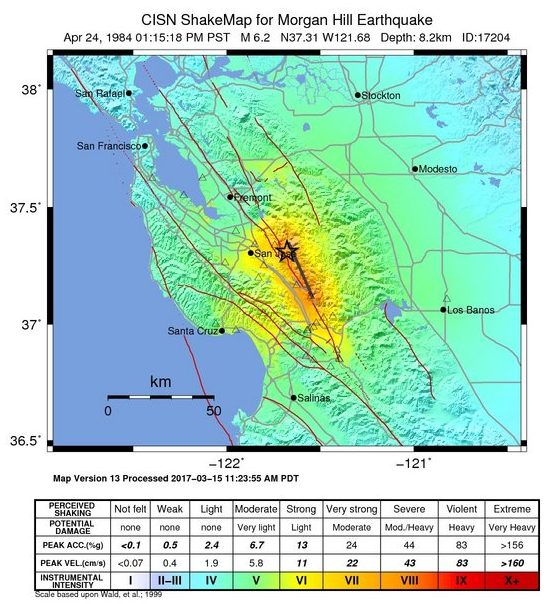
Shake maps, written ShakeMaps by the USGS, use high-quality, computer-interpolated data from seismograph networks to show areas of intense shaking. Shake maps are useful in the crucial minutes after an earthquake, as they show emergency personnel where the greatest damage likely occurred and help them locate possibly damaged gas lines and other utility facilities.


