3.2: Flow Past a Sphere at Low Reynolds Numbers
- Page ID
- 4163
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)We will make a start on the flow patterns and fluid forces associated with flow of a viscous fluid past a sphere by restricting consideration to low Reynolds numbers \(\rho U D / \mu\) (where, as before, \(U\) is the uniform approach velocity and \(D\) is the diameter of the sphere).
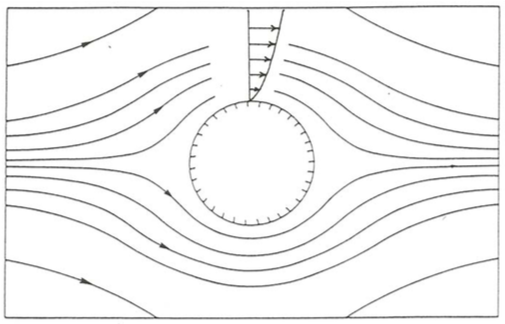
At very low Reynolds numbers, \(\mathrm{Re} \ll 1\), the flow lines relative to the sphere are about as shown in Figure \(\PageIndex{1}\). The first thing to note is that for these very small Reynolds numbers the flow pattern is symmetrical front to back. The flow lines are straight and uniform in the free stream far in front of the sphere, but they are deflected as they pass around the sphere. For a large distance away from the sphere the flow lines become somewhat more widely spaced, indicating that the fluid velocity is less than the free-stream velocity. Does that do damage to your intuition? One might have guessed that the flow lines would be more crowded together around the midsection of the sphere, reflecting a greater velocity instead—and as will be shown later in this chapter, that is indeed the case at much higher Reynolds numbers. (See a later section for more on what I have casually called flow lines here.) For very low Reynolds numbers, however, the effect of “crowding”, which acts to increase the velocity, is more than offset by the effect of viscous retardation, which acts to decrease the velocity.
The velocity of the fluid is zero everywhere at the sphere surface (remember the no-slip condition) and increases only slowly away from the sphere, even in the vicinity of the midsection: at low Reynolds numbers, the retarding effect of the sphere is felt for great distances out into the fluid. You will see later in this chapter that the zone of retardation shrinks greatly as the Reynolds number increases, and the “crowding” effect causes the velocity around the midsection of the sphere to be greater than the free-stream velocity except very near the surface of the sphere; more on that later.
Using the Navier-Stokes Equation to Describe Velocity and Pressure of a Creeping Flow
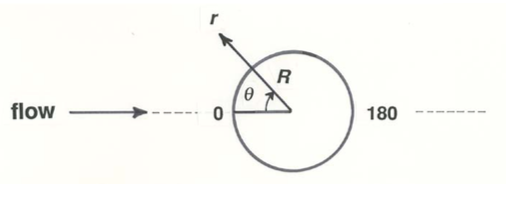
If you would like to see for yourself how the velocity varies in the vicinity of the sphere, Equations \ref{3.1} give the theoretical distribution of velocity \(v\), as a function of distance \(r\) from the center of the sphere and the angle \(\theta\) measured around the sphere from \(0^{\circ}\) at the front point to \(180^{\circ}\) at the rear point (Figure \(\PageIndex{2}\)):
\[\begin{array}{l}{u_{r}=-U \cos \theta\left(1-\frac{3 R}{2 r}+\frac{R^{3}}{2 r^{3}}\right)} \\ {u \theta=U \sin \theta\left(1-\frac{3 R}{4 r}-\frac{R^{3}}{4 r^{3}}\right)}\end{array} \label{3.1}\]
This result was obtained by Stokes (1851) by specializing the Navier–Stokes equations for an approaching flow that is so slow that accelerations of the fluid as it passes around the sphere can be ignored, resulting in an equation that can be solved analytically. I said in Chapter 2 that fluid density \(\rho\) is needed as a variable to describe the drag force on a sphere because accelerations are produced in the fluid as the sphere moves through it. If these accelerations are small enough, however, it is reasonable to expect that their effect on the flow and forces can be neglected. Flows of this kind are picturesquely called creeping flows. The reason, to which I alluded in the previous section, is that in the Navier–Stokes equations the term for rate of change of momentum becomes small faster than the two remaining terms, for viscous forces and pressure forces, as the Reynolds number decreases.
You can see from Equations \ref{3.1} that as \(r \rightarrow \infty\) the velocity approaches its free-stream magnitude and direction. The \(1/r\) dependence in the second terms in the parentheses on the right-hand sides of Equations \ref{3.1} reflects the appreciable distance away from the sphere the effects of viscous retardation are felt. A simple computation using Equations \ref{3.1} shows that, at a distance equal to the sphere diameter from the surface of the sphere at the midsection in the direction normal to the free-stream flow, the velocity is still only \(50 \%\) of the free-stream value.
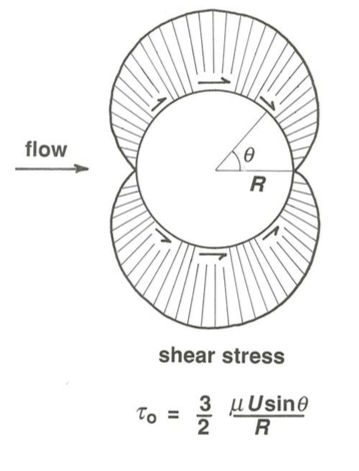
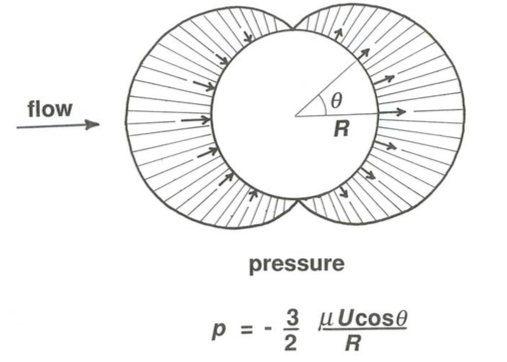
At every point on the surface of the sphere there is a definite value of fluid pressure (normal force per unit area) and of viscous shear stress (tangential force per unit area). These values also come from Stokes’ solution for creeping flow around a sphere. For the shear stress, you could use Equations \ref{3.1} to find the velocity gradient at the sphere surface and then use Equation 1.3.7 to find the shear stress. For the pressure, Stokes found a separate equation,
\[p-p_{0}=\frac{3}{2} \frac{\mu U R}{r^{2}} \cos \theta \label{3.2} \]
where \(p_{0}\) is the free-stream pressure. Figures \(\PageIndex{3}\) and \(\PageIndex{4}\) give an idea of the distribution of these forces. It is easy to understand why the viscous shear stress should be greatest around the midsection and least on the front and back surface of the sphere, because that is where the velocity near the surface of the sphere is greatest. The distribution of pressure, high in the front and low in the back, also makes intuitive sense. It is interesting, though, that there is a large front-to-back difference in pressure despite the nearly perfect front-to-back symmetry of the flow.
Using the Navier-Stokes Equation to Describe Drag Force (Stoke's Law)
You can imagine adding up both pressures and viscous shear stresses over the entire surface, remembering that both magnitude and direction must be taken into account, to obtain a resultant pressure force and a resultant viscous force on the sphere. Because of the symmetry of the flow, both of these resultant forces are directed straight downstream. You can then add them together to obtain a grand resultant, the total drag force \(F_{D}\). Using the solutions for velocity and pressure given above (Equations \ref{3.1} and \ref{3.2}), Stokes obtained the result
\[F_{D}=6 \pi \mu U R \label{3.3} \]
for the total drag force on the sphere. Density does not appear in Stokes’ law because it enters the equation of motion only the mass-time-acceleration term, which was neglected. For Reynolds numbers less than about one, the result expressed by Equation \ref{3.3} (called Stokes’ law) is in nearly perfect agreement with experiment. It turns out that in the Stokes range, for \(Re \ll 1\), exactly one-third of \(F_{D}\) is due to the pressure force and two-thirds is due to the viscous force.
Now pretend that you do not know anything about Stokes’ law for the drag on a sphere at very low Reynolds numbers. If you reason, as discussed above, that \(\rho\) can safely be omitted from the list of variables that influence the drag force, then you are left with four variables: \(F_{D}\), \(U\), \(D\), and \(\mu\). The functional relationship among these four variables is necessarily
\[f\left(F_{D}, U, D, \mu\right)= \text{const} \label{3.4} \]
You can form only one dimensionless variable out of the four variables \(F_{D}\), \(U\), \(D\), and \(\mu\), namely \(F_{D} / \mu U D\). So, in dimensionless form, the functional relationship in Equation \ref{3.4} becomes
\[\frac{F_{D}}{\mu U D}= \text{const} \label{3.5} \]
You can think of Equation \ref{3.5} as a special case of Equation 2.3.1. If you massage Stokes’ law (Equation \ref{3.3}) just a bit, by dividing both sides of the equation by \(\mu U R\) to make the equation dimensionless, and using the diameter \(D\) instead of the radius \(R\), you obtain
\[\frac{F_{D}}{\mu U D}=3 \pi \label{3.6} \]
Compare this with Equation \ref{3.5} above. You see that dimensional analysis alone, without recourse to attempting exact solutions, provides the equation to within the proportionality constant. Stokes’ theory provides the value of the constant.
The flow pattern around the sphere and the fluid forces that act on the sphere gradually become different as the Reynolds number is increased. The progressive changes in flow pattern with increasing Reynolds number are discussed in more detail later in this chapter, after quite a bit of necessary further background in the fundamentals of fluid dynamics.


