8.1: Earth’s Heat Budget

The balance of incoming and outgoing heat on Earth is referred to as its heat budget. As with any budget, to maintain constant conditions the budget must be balanced so that the incoming heat equals the outgoing heat. The heat budget of Earth appears below (Figure \(\PageIndex{1}\)).

Figure \(\PageIndex{1}\) Earth’s heat budget. Of all of the solar radiation reaching Earth, 30% is reflected back to space and 70% is absorbed by the Earth (47%) and atmosphere (23%). The heat absorbed by the land and oceans is exchanged with the atmosphere through conduction, radiation, and latent heat (phase change). The heat absorbed by the atmosphere is eventually radiated back into space (PW).

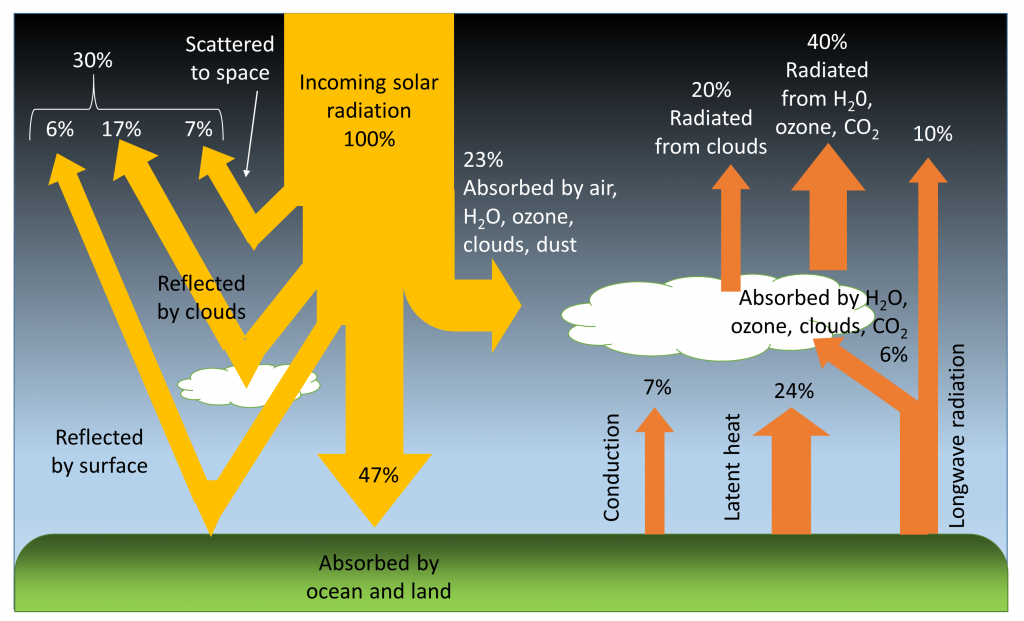
Of all of the solar energy reaching the Earth, about 30% is reflected back into space from the atmosphere, clouds, and surface of the Earth. Another 23% of the energy is absorbed by the water vapor, clouds, and dust in the atmosphere, where it is converted into heat. Just under half (47%) of the incoming solar radiation is absorbed by the land and ocean, and this energy heats up the Earth’s surface. The energy absorbed by the Earth returns to the atmosphere through three processes; conduction, radiation, and latent heat (phase change)(Figure \(\PageIndex{1}\)).

Conduction is the transfer of heat through direct contact between the surface and the atmosphere. Air is a relatively poor thermal conductor (which means it is a good insulator), so conduction represents only a small part of the energy transfer between the Earth and the atmosphere; equal to about 7% of the incoming solar energy.

All bodies with a temperature above absolute zero (-273^o C) radiate heat in the form of longwave, infrared radiation (see the electromagnetic spectrum in section 6.5). The warmed Earth is no exception, and about 16% of the original solar energy is radiated from the Earth to the atmosphere (Figure \(\PageIndex{1}\)). Some of this radiated energy will dissipate into space, but a significant amount of heat will be absorbed by the atmosphere. This is the basis for the greenhouse effect (Figure 8.12). In the greenhouse effect, shortwave solar radiation passes through the atmosphere and reaches the Earth’s surface where it gets absorbed. When the radiation is re-emitted by the Earth, it is now in the form of long wavelength, infrared radiation, which does not easily pass through the atmosphere. Instead, this infrared radiation is absorbed by the atmosphere, particularly by the greenhouse gases such as CO₂, methane, and water vapor. As a result, the atmosphere heats up. Without the greenhouse effect, the average temperature on Earth would be about -18^o C, which is too cold for liquid water, and therefore life as we know it could not exist!

Figure \(\PageIndex{2}\) An explanation of the greenhouse effect (By US EPA [Public domain], via Wikimedia Commons).

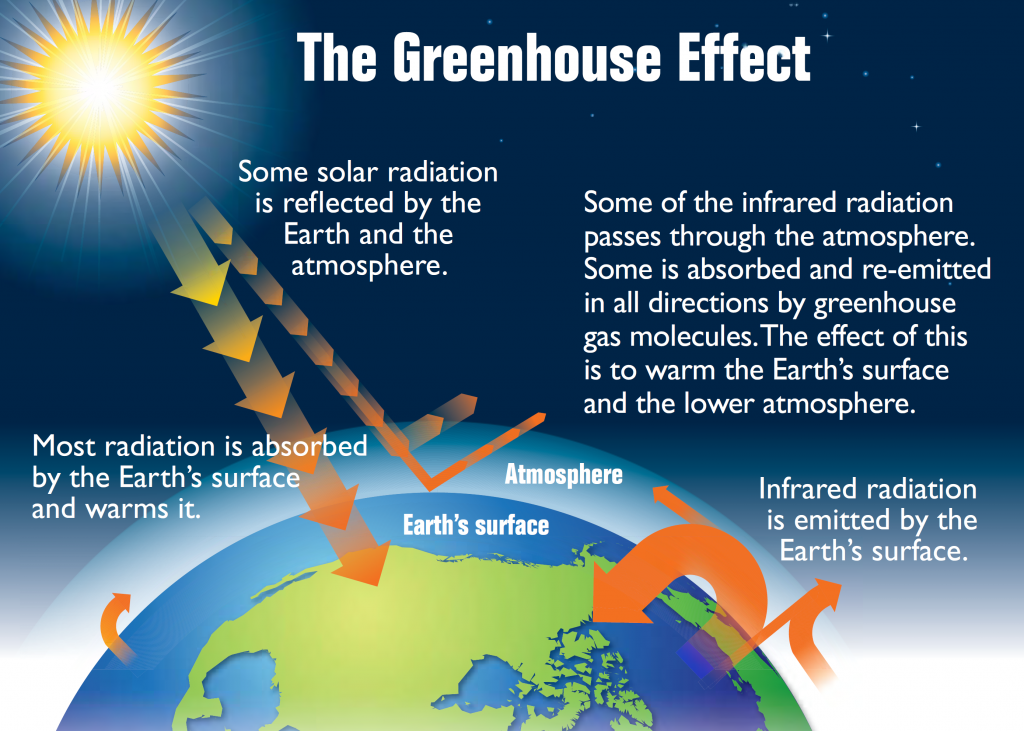
There is a great deal of concern about the greenhouse effect across the globe; not because of the presence of the effect itself, but because the effect is intensifying, causing climate change or global warming. Since the Industrial Revolution the atmospheric concentrations of the major greenhouse gases, particularly CO₂ and methane, have increased dramatically due to industrialization, the burning of fossil fuels, and deforestation. At the same time, there has been rapid warming of the global climate; CO₂ concentrations have increased more than 25% and global temperature has risen by 0.5^o C over the past century. Unless production of these greenhouse gases is curbed, this rapid warming trend may continue, with potentially dire consequences. See section 8.5 for detailed information on the causes and effects of climate change.

The largest pathway for heat exchange between the land or oceans and the atmosphere is latent heat transferred through phase changes; heat released or absorbed when water moves between solid, liquid, and vapor forms (see section 5.1). Heat must be added to liquid water to make it evaporate, and when water vapor is formed, that heat is removed from the ocean and transferred to the atmosphere along with the water vapor. When water vapor condenses into rain, that heat is then returned to the oceans. The same process happens with the formation and melting of ice. Heat is absorbed by ice when it melts, and heat is released when ice forms, and these phase changes transfer heat between the oceans and the atmosphere.

To complete the heat budget, the heat that is absorbed by the atmosphere either directly from solar radiation or as a result of conduction, radiation and latent heat, is eventually radiated back into space (Figure \(\PageIndex{1}\)).

Differential Heating of Earth’s Surface

If the Earth was a flat surface facing the sun, every part of that surface would receive the same amount of incoming solar radiation. However, because the Earth is a sphere, sunlight is not equally distributed over the Earth’s surface, so different regions of Earth will be heated to different degrees. This differential heating of Earth’s surface occurs for a number of reasons. First, because of the curvature of Earth, sunlight only falls perpendicularly to the surface at the center of the sphere (equatorial regions). At any other point on Earth, the angle between the surface and the incoming solar radiation is less than 90^o. Because of this, the same amount of incoming solar radiation will be concentrated in a smaller area at the equator, but will be spread over a much larger area at the poles (Figure \(\PageIndex{3}\)). Thus the tropics receive more intense sunlight and a greater amount of heating per unit of area than the polar regions.

Figure \(\PageIndex{3}\) Because of the curvature of the Earth, the same amount of sunlight will be spread out over a larger area at the poles compared to the equator. The equator therefore receives more intense sunlight, and a greater amount of heat per unit of area (By Thebiologyprimer (Own work) [CC0], via Wikimedia Commons).

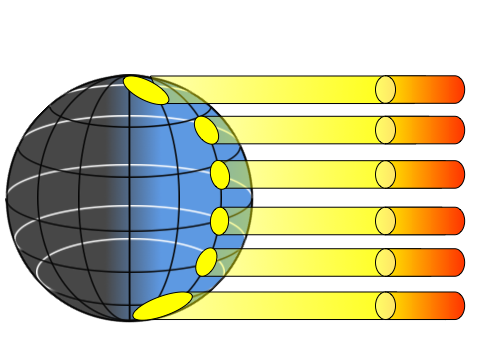
The angle at which sunlight strikes the Earth contributes to differential heating of the surface in an additional way. At the poles, because of the angle at which the solar energy strikes the surface, more of the light will glance off of the surface and the atmosphere and be reflected back into space. At the equator, the direct angle with which light reaches the surface results in more of the energy being absorbed rather than reflected. Finally, the poles reflect more solar energy than other parts of the Earth because the poles have a higher albedo. The albedo refers to reflectivity of a surface. Lighter surfaces are more reflective than darker surfaces (which absorb more energy), and therefore have a higher albedo. At the poles, the ice, snow and cloud cover create a much higher albedo, and the poles reflect more and absorb less solar energy than the lower latitudes. Through all of these mechanisms, the poles absorb much less solar radiation than equatorial regions, which is why the poles are cold and the tropics are very warm.

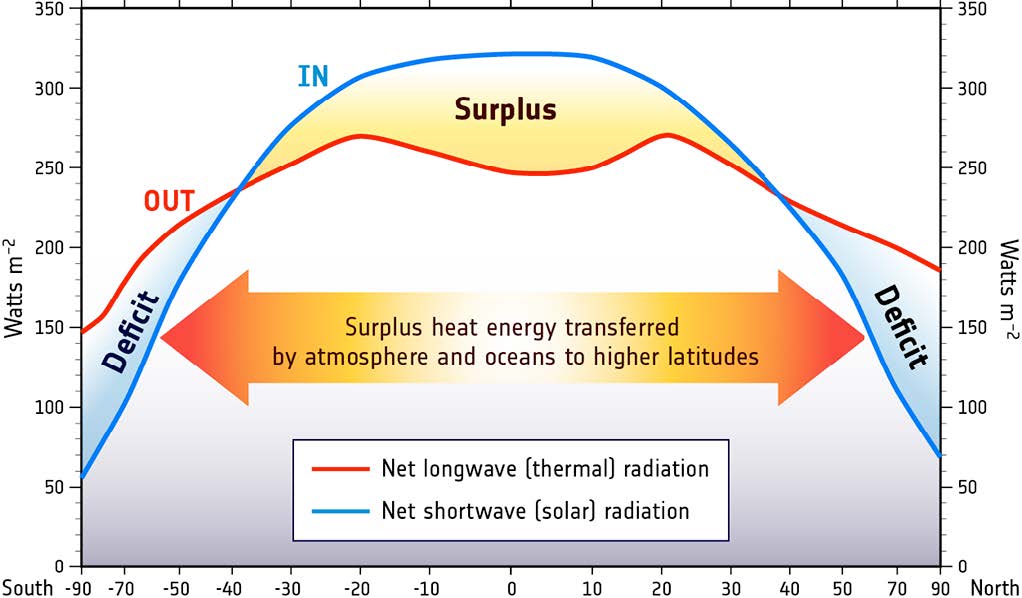
But there is an interesting twist to this global distribution of heat. The tropical regions actually receive more radiant heat than they emit, and the poles emit more heat than they receive (Figure \(\PageIndex{4}\)). We should therefore expect that the tropics will be getting continually warmer, while the poles become increasingly cold. Yet this is not the case; so what is happening? Rather than the heat remaining isolated near the equator, about 20% of the heat from the tropics is transported to the poles before it is emitted. This large scale transport of energy moderates the climates at both extremes. The mechanisms for this heat transfer are ocean and atmospheric circulation, the topic of the next section.

Figure \(\PageIndex{4}\) The balance between heat gain and heat loss as a function of latitude. Excess heat received near the equator is transferred towards the poles (National Oceanography Centre (NOC). Creative Commons 3.0 unported license).

The idea of differential heating of the Earth’s surface is fundamental to understanding a wide range of oceanographic and atmospheric processes. This differential heating leads to atmospheric convection, which creates winds, which blow over the water and create waves and surface currents, and these currents influence nutrient distribution, which promotes primary production, which then supports the rest of the ocean ecosystem. So there’s a lot riding on the simple fact that more light reaches the tropics than the poles!