7.4: Structural losses gains
- Page ID
- 16207
In the foregoing sections we have assumed that while the beach and surf zone may breathe and may display three-dimensionality or simply two-dimensionality under cross-shore wave forcing, the amount of sediment in the active zone remains constant. On engineering scales (1 to 100 years) a number of processes exist that violate these as- sumptions. A prime one is alongshore transport gradients, treated in Ch. 8. Shoreline orientations and hence alongshore transport rates will vary, which will lead to either shoreline advance or retreat, while the upper shoreface profile will remain in profile equilibrium. On these engineering scales aeolian (transport by wind) sand loss from the beach to the dunes and nearshore offshore canyons may be important as well. They can be accounted for as sinks.
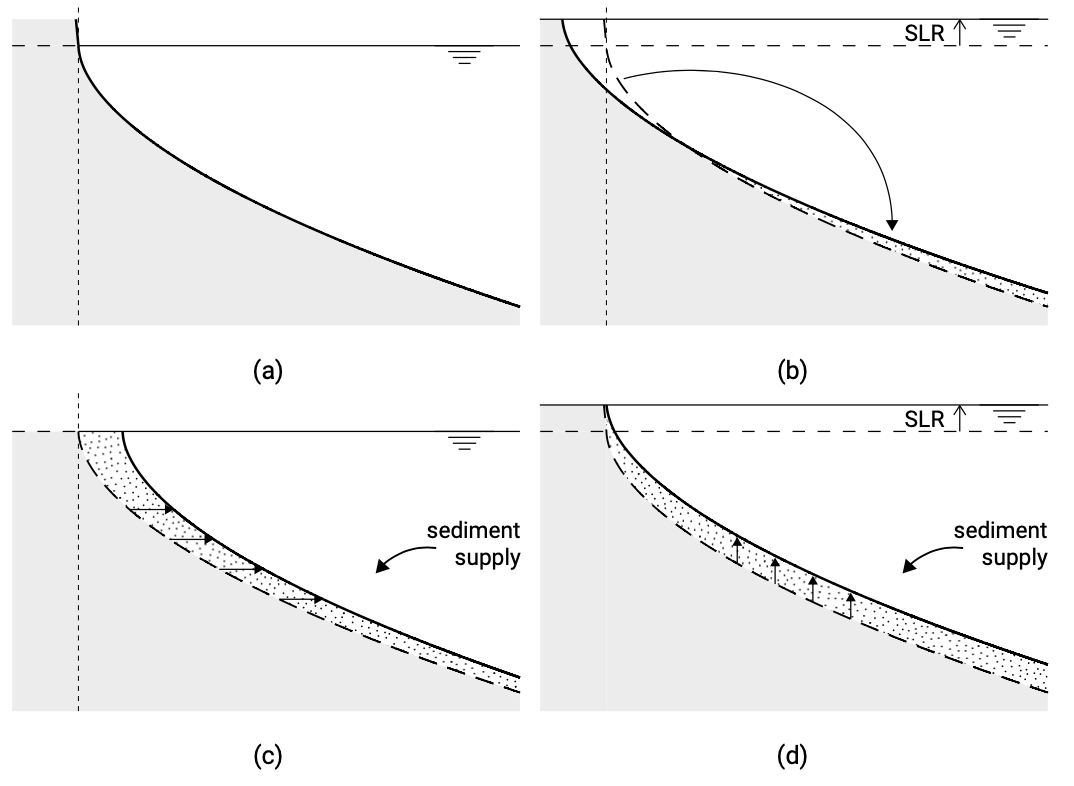
A further ‘sink’, which is virtual because no sediment is lost, is due to relative sea-level rise. This effect was first described by Bruun (1962). As explained in Sect. 2.5.2, Bruun argued that the response of the upper shoreface to an increased MSL is so fast that the equilibrium upper shoreface profile will adjust to the same profile but relative to the new MSL (Figs. 2.22 and 7.19b). Hence, even though no sediment is lost from the profile, the shoreline retreats.
The retreat distance \(R\) follows from sediment continuity considerations. The new equilibrium profile requires that the shoreline retreats to supply sediment to the lower surf zone in order to maintain the equilibrium profile. Horizontal retreat \(R\) leads to a sediment yield of \(R \times d\) where \(d\) is the height over which erosion and sedimentation takes place (viz. the closure depth plus dune crest height). Relative Sea Level Rise (RSLR) leads to a demand of sand of \(L \times\) RLSR where \(L\) is the length over which the erosion and sedimentation takes place. Balancing the two (no net loss or gain) gives:
\[R_{\text{RSLR}} = \text{RSLR} (L/d)\]
where RSLR is the rise of relative sea level above MSL, \(L\) the length over which the erosion and sedimentation takes place and \(d\) the corresponding height. In practice the ratio \(L/d\) is of the order 50 to 150 (Zhang et al., 2004).
Sediment supply may counteract the effect of shoreline retreat. Fig. 7.19c assumes a horizontal shift of the profile in the case of a sediment source. If the rate of sediment supply keeps up with the rate of sea-level rise, the accommodation space created by the sea-level rise is filled by incoming sediments (Fig. 7.19d) and the profile moves upward only; the position of the shoreline remains unchanged.
Quite often the Bruun rule is used to assess possible future effects of sea-level rise. Although it gives qualitative insight into profile response to sea-level changes, it is not a valid model approach in general due to oversimplifications (see e.g. Ranasinghe and Stive (2009)). One of the problems in the application of the Bruun rule – for instance when considering shore nourishment to counteract the effects of sea-level rise – is that time is required for equilibrium to be established. Often this point is not considered by coastal engineers. Rapid sea-level rise induces rapid coastal response. However, the timescale on which present sea-level rise influences coastal evolution in general and coastal erosion in particular, is relatively large. Present coastal evolution on smaller time and spatial scales is dominated by other causes.