10.5.2: Characteristics of Crystals Belonging to the Different Crystal Systems
- Page ID
- 18481
10.5.2.1 Cubic System
The cubic system is also called the isometric system. Crystals have high symmetry, all having four 3-fold or four 3 axes. Some have three 4-fold axes as well, and some have 2-fold axes or mirror planes. Cubes and octahedra are examples of forms belonging to the cubic system, but other forms belong to the cubic system, too. We saw some special forms (cube, octahedron, dodecahedron, and trapezohedron) and the general form, a hexoctahedron, earlier in this chapter.
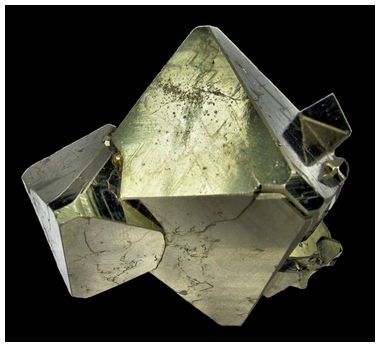
The three photos below are crystals belonging to the cubic system. Figure 10.48 shows a dodecahedral garnet. It has symmetry 4/m32/m; a dodcahedron is a special form in that point group. Figure 10.49 shows a pyrite crystal with 5-sided faces. The form is called a pyritohedron. Pyrite has symmetry 2/m3, and the pyritohedron is the general form in the 2/m3 point group. Figure 10.50 shows pyrite octahedra. Some small cube faces are present, too. Like the dodecahedral garnet, these crystals have symmetry 4/m32/m. And like the dodecahedron, octahedra and cubes are special forms in the class.
Crystals of the cubic system may have many different and complex shapes, but all tend to be equant, meaning they are approximately equidimensional. Often the crystals contain only one or two forms.
We saw other examples of minerals whose crystals belong to the cubic system in earlier chapters:
•pyrite in Figure 3.3 (Chapter 3)
•halite in Figure 3.2 (Chapter 3)
•garnet in Figure 3.6 (Chapter 3)
•fluorite in Figure 3.69 (Chapter 3), Figure 4.37 (Chapter 4)
•galena in Figure 9.34 (Chapter 9)
10.5.2.2 Hexagonal and Rhombohedral Systems
Hexagonal crystals have a single 6-fold, or 6 axis. Rhombohedral crystals contain a single 3-fold or 3 axis. Crystals with more than one 3-fold or 3 axis belong to the cubic system. No crystals have more than one 6-fold or 6 axis. Crystals in either system may also have 2-fold axes and mirror planes. Because they have one direction that is different from others, hexagonal crystals are often prisms of three or six sides terminated by pyramid faces. Other forms, including scalenohedron and rhombohedron, are also common in both the hexagonal and rhombohedral systems.
Figure 10.51 shows a crystal of hanksite, a rare sodium-potassium evaporite mineral that has symmetry 6/m. The hexagonal symmetry is reminiscent of apatite crystals. Figure 10.52 shows prismatic tourmaline crystals. They have symmetry 3m. Figure 10.53 shows one large, and several small, calcite scalenohedra. Calcite has symmetry 32m, but calcite crystals have many common forms and come in many shapes.
We saw other examples of minerals whose crystals belong to the hexagonal or rhombohedral systems in earlier chapters:
•quartz in Figure 1.8 (Chapter 1)
•beryl in Figure 1.12 (Chapter 1), Figure 4.1 (Chapter 4), Figure 6.89 (Chapter 6), Figure 9.60 (Chapter 9)
•tourmaline in Figure 4.13 (Chapter 4) and 6.22 (Chapter 6)
•calcite in Figure 3.1 (Chapter 3)
•molybdenite in Figure 3.40 (Chapter 3)
•graphite in Figure 3.78 (Chapter 3)
•ilmenite in Figure 9.55 (Chapter 9)
•chabazite in Figure 7.56 (Chapter 7)
Note that micas and other sheet silicate minerals sometimes appear to be hexagonal. For example, look at the biotite crystals in Figure 6.62, the lepidolite in Figure 6.66, and the clinochlore in Figure 6.69. In two dimensions they appear hexagonal, but if you could see the third dimension clearly it would be apparent that they are not. Most sheet silicates are monoclinic. Tourmaline, too, often appears hexagonal but a close look reveals that the crystal cross sections are 6-sided but are not true hexagons.
10.5.2.3 Tetragonal System
All tetragonal crystals have one 4-fold or one 4 axis of symmetry; crystals that have more than one 4-fold or 4 axis must belong to the cubic system. Tetragonal crystals may also have 2-fold axes and mirror planes. As with the crystals in the hexagonal system, tetragonal crystals are often combinations of prisms with other forms. Figure 10.54 shows a green apophyllite crystal from Bombay, India. It has prominent tetragonal prism and tetragonal pyramid faces. Compare this with the other photo of apophyllite earlier in this chapter (Figure 10.13). The apophyllite in Figure 10.54 is on top of stilbite. Apophyllite (which is a sheet silicate) usually occurs with zeolites such as stilbite (which is a monoclinic framework silicate). We find these minerals most commonly as secondary minerals in vugs and pockets in basalt.
Figure 10.55 show tetragonal vesuvianite crystals with both prism and pyramid faces. Both apophyllite and vesuvianite have symmetry 4/m2/m2/m. Figure 10.56 shows another tetragonal mineral, wulfenite, forming thin square orange crystals. Wulfenite crystals have symmetry 4/m.
10.5.2.4 Orthorhombic System
Crystals in the orthorhombic system have either three perpendicular 2-fold axes, two perpendicular mirror planes paralleling a 2-fold axis, or three perpendicular 2/m axes. A shoebox shape is an excellent example, but many shapes and forms are possible. The sulfur crystal shown in Figure 10.57, the topaz in Figure 10.58, and the barite in Figure 10.59 all have symmetry 2/m2/m2/m. The crystal with disphenoids in Figure 10.40, earlier in this chapter, is also orthorhombic and belongs to point group 222. Sometimes orthorhombic crystals may be rather equant but they are often quite tabular, like the barite crystals seen in the opening photo of this chapter (Figure 10.1) and here in Figure 10.59.
10.5.2.5 Monoclinic System
A single 2-fold axis, a single mirror, or a 2-fold axis perpendicular to a mirror characterize crystals in the monoclinic system. In the simplest case, monoclinic crystals may appear as shoe boxes distorted in one direction so that two faces are not rectangles. The symmetry may be hard to see because monoclinic crystals often comprise many different forms. Figure 10.60 shows gypsum, Figure 10.61 shows a large orthoclase crystal from the Jarilla Mountains, southern New Mexico, and Figure 10.62 shows a cluster of heulandite crystals (Ca-Na zeolite) from Maharashtra, India. All these monoclinic crystals have symmetry 2/m but it is hard to discern in the heulandite crystals.
We saw other examples of minerals whose crystals belong to the monoclinic system in earlier chapters:
• twinned gypsum Figure 4.39 (Chapter 4)
• twinned orthoclase in Figure 4.41 (Chapter 4)
• augite in Figure 6.78 (Chapter 6)
10.5.2.6 Triclinic System
Triclinic crystals have no symmetry greater than an inversion center. The photos below show three examples: albite from Tuscany, Italy (Figure 10.63), amblygonite from Brazil (Figure 10.64), and rhodonite from Brazil (Figure 10.65). The photo of albite also contains minor amounts of black tourmaline and quartz. These crystals all have symmetry 1, equivalent to an inversion center, and no more. It appears that the albite crystal might have a vertical mirror and so, perhaps, be monoclinic. But albite, like all plagioclase, is triclinic. In contrast with plagioclase feldspars, K-feldspar is monoclinic at high temperature (sanidine, orthoclase) and triclinic at low temperature (microcline).
We saw other examples of feldspar crystals in the triclinic system in earlier chapters:
•albite in Figure 6.37 (Chapter 6)
•anorthite in Figure 6.38 (Chapter 6)