8.4.2: Metamorphic Phase Diagrams

Last updated
Save as PDF

Page ID: 18619

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

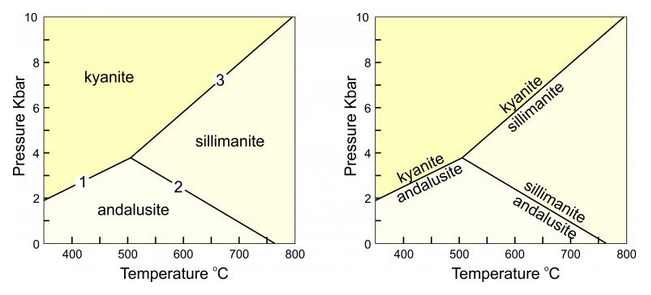
8.39.jpg — Figure 8.39: The stability of different aluminosilicate minerals

The Laws of Thermodynamics allow us to predict which minerals form under particular conditions. We use phase diagrams like the ones seen in Figure 8.39 to show the conditions at which particular minerals or mineral assemblages are stable. The diagram on the left, for example, depicts stability fields for kyanite, sillimanite, and andalusite, the Al₂SiO₅ polymorphs. The different fields are the ranges of pressure and temperature where each polymorph is stable. The reaction lines separating the fields show the conditions at which chemical reactions occur.

The diagram on the right shows the same information, but the reactions are labeled, not the stability fields. Petrologists use both kinds of diagrams. These diagrams tell us that rocks containing kyanite form at low temperature and high pressure, rocks containing andalusite form at low pressure, and those containing sillimanite form at high temperature. The diagram also allows us to make predictions: for example, if a rock containing andalusite is metamorphosed at high temperature, the andalusite will change into sillimanite.

Phase diagrams for simple chemical systems may only contain a few reactions. The aluminosilicate diagram (above in Figure 8.39) is an example. All the minerals considered have the same composition and are related by three reactions:

kyanite = andalusite (reaction 1)
Al₂SiO₅ = Al₂SiO₅

andalusite = sillimanite (reaction 2)
Al₂SiO₅ = Al₂SiO₅

kyanite = sillimanite (reaction 3)
Al₂SiO₅ = Al₂SiO₅

Reactions between kyanite, andalusite, and sillimanite are only three of many that involve minerals of Al₂O₃, SiO₂, and H₂O chemical system. Other minerals consist of these same components. The phase diagram seen below in Figure 8.40 is a more complete phase diagram for the system. It includes all stable minerals and reactions. Some of the reactions give off water vapor, labeled as H₂O. Eight minerals are involved but most have restricted stability fields.

8.40.jpg — Figure 8.40: Minerals and reactions in the system Al₂O₃ – SiO₂ – H₂O

Stable Minerals in the System: Al₂O₃ – SiO₂ – H₂O
Mineral	Formula
kaolinite (Ka) quartz (Qz) pyrophyllite (Py) diaspore (Dsp) kyanite (Ky) andalusite (And) sillimanite (Sill) corundum (Co)	Al₄(Si₄O₁₀)(OH)₈ SiO₂ Al₂Si₄O₁₀(OH)₂ AlO(OH) Al₂SiO₅ Al₂SiO₅ Al₂SiO₅ Al₂O₃

According to this phase diagram, at low temperature, kaolinite (a clay mineral) and quartz are stable together, but as temperature increases to almost 300 ̊C, kaolinite and quartz react to produce pyrophyllite and H₂O vapor. With a bit more heating, any leftover kaolinite decomposes to pyrophyllite, diaspore, and H₂O vapor. Pyrophyllite itself breaks down at higher temperatures – around 400 ̊C, so pyrophyllite is only be stable over a limited range of temperature. At temperatures over about 450 °C, the only stable minerals are corundum, quartz, and the three Al₂SiO₅ polymorphs (andalusite, kyanite, sillimanite), but corundum and quartz cannot be found together.

Phase diagrams like this permit prediction of the pressures and temperatures at which individual minerals and specific mineral assemblages will form. Conversely, these diagrams allow us to estimate the pressure and temperature of formation for some rocks containing specific minerals. For example, rocks containing kaolinite and quartz are constrained to have formed at temperatures below about 300 °C (because kaolinite is not stable at higher temperature). If andalusite accompanies the kaolinite and quartz, pressure is restricted to less than 1 kbar. The rock must have equilibrated at conditions within the small triagular field near the 300^o lable on the bottom axis.

Using Phase Diagrams

If a rock contains a specific mineral assemblage at equilibrium, we can use an appropriate phase diagram to put restrictions on the conditions at which the rock formed. For example, the diagram in this box is modified from Figure 8.40. It lists all the different 3-mineral assemblages that are stable within fields between reactions. (For simplicity, only assemblages in the high-pressure fields are labeled.) The different assemblages are consistent with the reactions shown.

8.41.png — Figure 8.41: Minerals and reactions in the system Al₂O₃-SiO₂-H₂O

But, besides 3-mineral assemblages, different pairs of minerals are stable within the different fields. And individual minerals are stable in different parts of the diagram. In all, there are 87 different mineral combinations stable in different parts of the diagram. If we labeled them all, the diagram would become very messy. This is why most phase diagrams only have reactions labeled.

Consider a rock that contains only diaspore. This diagram says it could have formed in any of the fields 1 through 5. In Field 6, diaspore is not stable and breaks down to corundum and H₂O. Suppose a rock contains diaspore with pyrophyllite. It is stable in Fields 1, 2, and 3, but not at higher temperatures because the two minerals would react to kyanite and H₂O. And, consider a rock that contains pyrophyllite, diaspore, and H₂O. It is stable in Field 3 only. At lower temperature the minerals react to produce kaolinite, and at higher temperature pyrophyllite and diaspore react to produce kyanite. What about four minerals together? Four minerals can only be stable if conditions lie on one of the reaction lines. Thus, we see that the more minerals present, the more restricted the conditions of formation. This is true for all chemical systems at equilibrium, not just minerals in rocks.