5.6: Classifying Rivers

Rivers are varied in so many ways that you should expect complexity in classification. Rivers can be classified in several ways:

• by the nature of their substrate
• by the percentage of time they flow
• by their relationship to the groundwater table
• by the kind of sediment load they carry
• by the dominant particle size of the bed sediment • by their morphology

In the following I’ll make some comments on classification of rivers in each of these ways. (But I’ll defer discussion of classification of rivers by sediment load until later, after I’ve talked about the sediment load.)

Nature of Substrate:

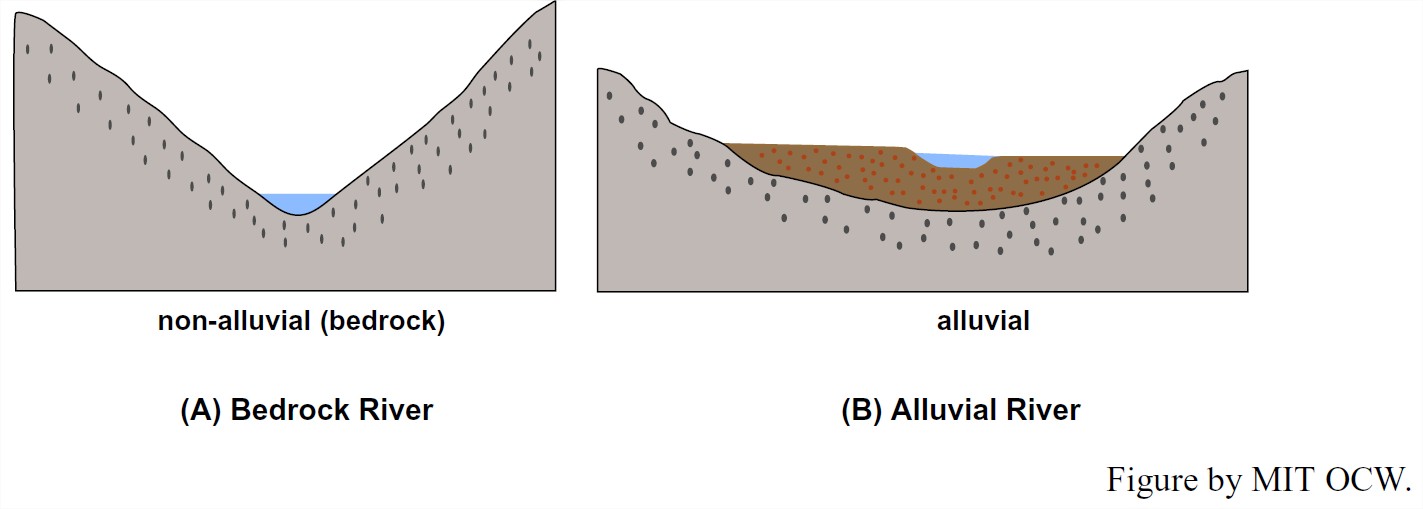
Some rivers, especially small rivers in mountainous areas, flow directly on bedrock. Such rivers are called bedrock rivers, or non-alluvial rivers (Figure 5-22A). Other rivers, especially large rivers, flow on a bed a sediment they have deposited and can continue to transport. Such rivers are called alluvial rivers (Figure 5-22B). Of course, some rivers lie in between, in that they have bedrock beds in some reaches and alluvial beds in other reaches (Figure 5-23). Alluvial rivers have held most of the problems, fascination, and importance for fluviologists, but if you’re a white-water canoeing enthusiast I suppose you’re more interested in bedrock rivers.

Figure 5-22. A) Bedrock rivers. B) Alluvial rivers.

Relationship to Groundwater Table:

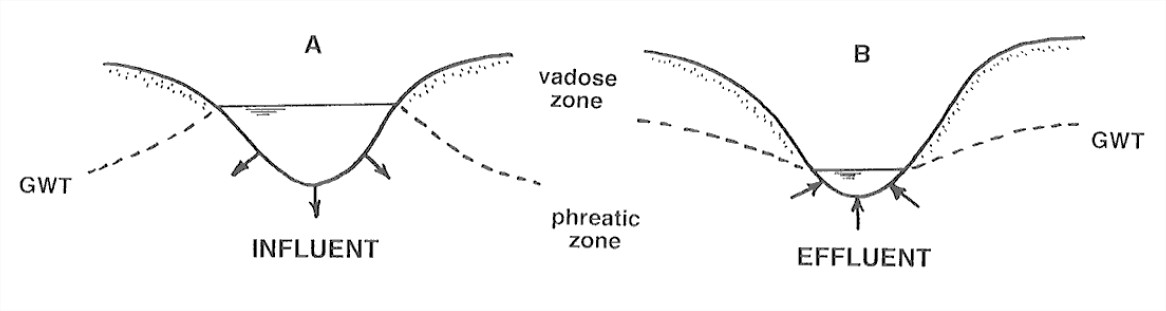
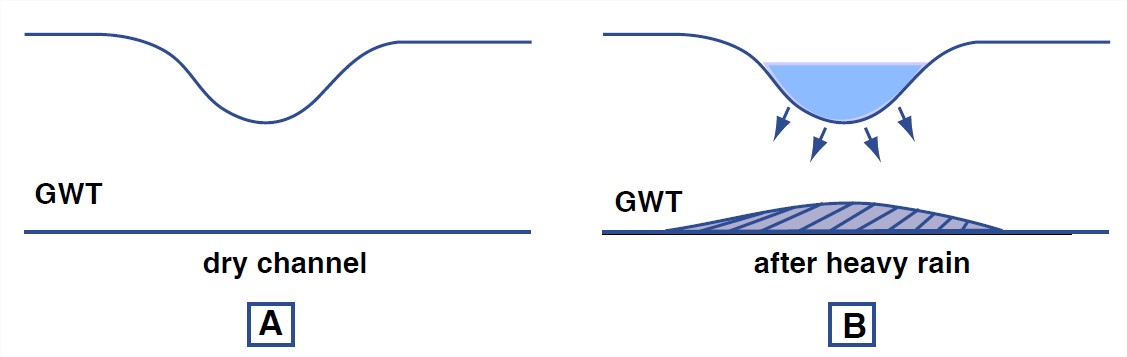
Think about the relative position of the water surface in the river and the local groundwater table. If the water surface in the river lies above the local groundwater table in the river banks, then the river loses water to its banks. Such a river is said to be an effluent river (Figure 5-24A). On the other hand, if the water surface in the river lies below the local groundwater table in the banks, then the river gains water from its banks. Such a river is said to be influent river (Figure 5-24B). (These two terms are difficult to keep straight. It helps to think in terms of alternative terminology: an effluent river is also called a gaining river, because it is gaining water from the adjacent substrate, and an influent river is called a losing river.) Keep in mind that it’s also possible for the groundwater table to lie entirely below the river bed (Figure 5-25). The river is still called an effluent river in that case.

Percentage of Time the River Flows:

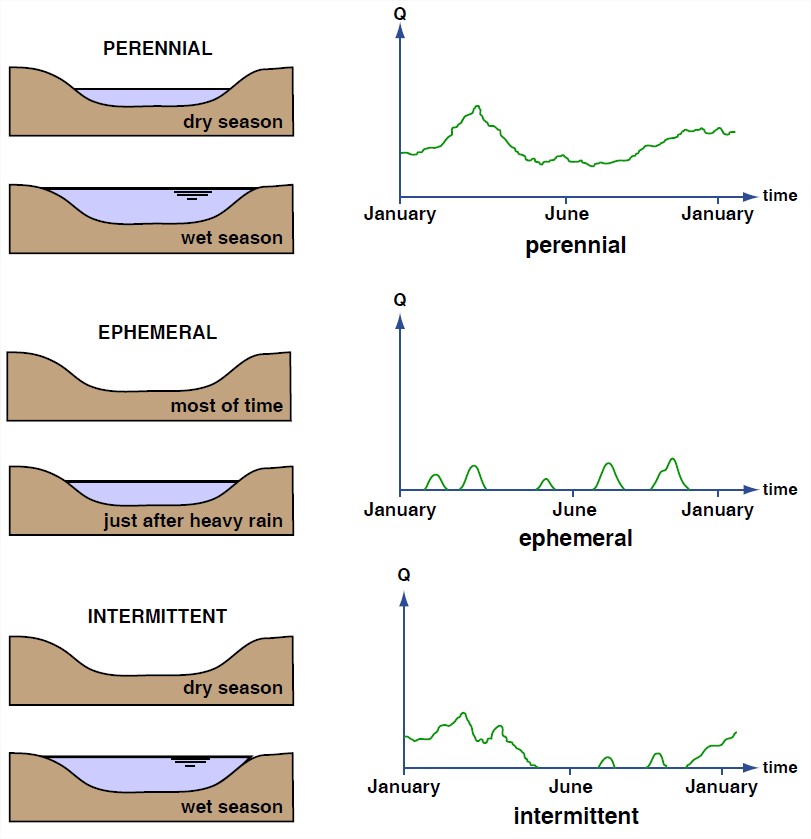
Some rivers show a flow of water all the time, even long after the last rainstorm in the watershed. Such a river is called a perennial stream. Other rivers flow for only a short time after a rainstorm, and for the rest of time, usually most of the time, their beds are dry. Such a river is called an ephemeral stream. Some rivers lie between these two extremes: during the wetter part of the year they flow as a perennial stream, whereas during the drier part of the year they flow as an ephemeral stream. Such a river is called an intermittent stream. Figure 5-26 shows cartoon hydrographs of a perennial stream, an ephemeral stream, and an intermittent stream.

Figure 5-24. A) An influent river. B) An effluent river.

Figure 5-25. The groundwater table can lie entirely below the river bed. A) Before a heavy rain. B) After a heavy rain.

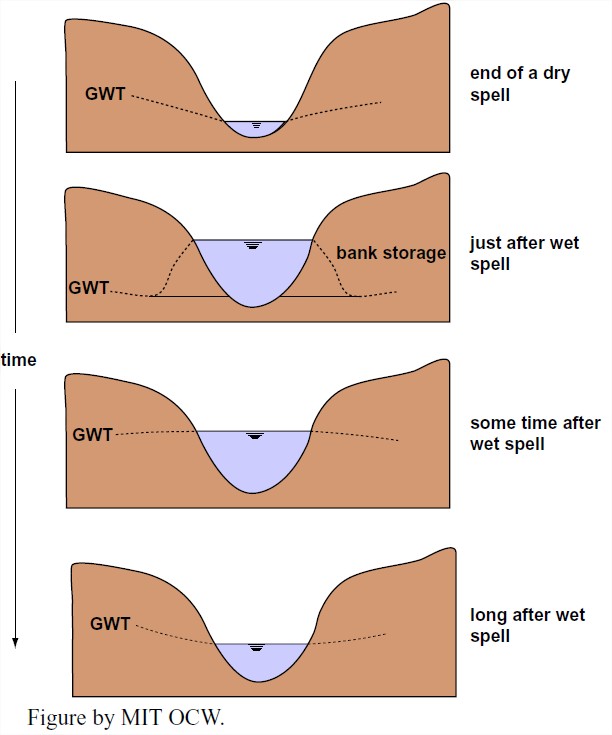
In an ephemeral stream, the water table always lies below the bed of the stream; the stream never receives any water from its bed or banks. In a perennial stream, the situation is more complicated. Think about the relationship between the river level and the groundwater table in some time period that starts in a dry spell, extends through a major rainfall event in the watershed, and ends during another dry spell. At the end of the first dry spell the river level lies below the groundwater table in the river banks (Figure 5-27A). After a heavy rainfall the river stage rises rapidly to lie well above the level of the groundwater table in the banks (Figure 5-27B). Groundwater is stored in the river banks, in the sense that the groundwater table is locally and temporarily higher there than in the surroundings. At the end of the rainy period both the river stage and the groundwater level are of about the same height and are about at their highest (Figure 5-27C). Then (Figure 5-27D) both the river stage and the groundwater table fall back to the dry-spell situation shown in Figure 5-27A. This sequence of events is called the runoff cycle.

Figure 5-26. Perennial, intermittent, and ephemeral streams, and associated representative hydrographs.

Morphology:

The morphology of rivers, especially in plan view, varies enormously. The most common way to classify rivers is on the basis of their plan-view morphology. The morphology of rivers is bound up in a complex way with the nature of the sediment load, so a full appreciation of this section must await Section 8, on the sediment load of rivers.

Figure 5-27. The runoff cycle.

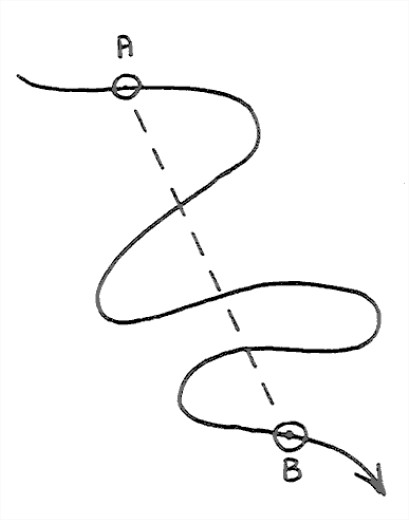
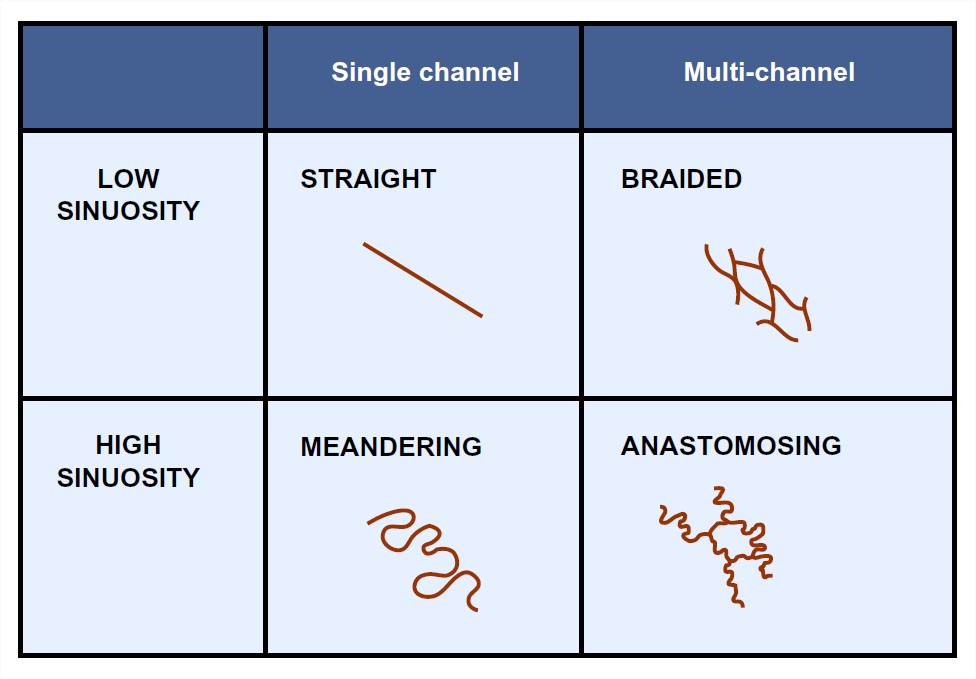
Two characteristics are used in the classification of rivers by morphology: sinuosity and “multichanneledness”. Sinuosity can be defined with respect to two arbitrary points along the river as the ratio of the along-channel distance between the two points and the straight-line distance between the points (Figure 5-28). The minimum sinuosity, for a straight river, is 1; the more sinuous the river, the greater its sinuosity. Very sinuous rivers can have values of sinuosity approaching 4. Multichanneledness, an awkward but useful word, reflects the number of individual flow channels shown by a river in a cross-stream traverse across the entire river system. Many rivers have only one channel, except perhaps where an occasional island divides the channel into two. Other rivers show a large number of channels, all of about the same size and nature, separated by numerous bars and islands. The individual channels of such a river are called anabranches.

Sinuosity and multichanneledness are to a large extent independent of one another, so it’s natural to resort to a two-independent-variable pigeonhole classification with sinuosity along one axis and multichanneledness along the other axis (Figure 5-29). Straight rivers—those with sinuosity not much greater than 1—are surprisingly uncommon in nature. In fact, it’s hard to keep rivers straight: humans straighten them out for their own purposes, and the rivers try to become sinuous again, by erosion and deposition on the banks. Both braided rivers (low-sinuosity, multichanneled) and meandering rivers (high sinuosity, single-channeled) are very common; more on them later. Anastomosing rivers (high-sinuosity, multichanneled) are much less common.

Figure 5-28. The sinuosity of a river.

Figure 5-29. One way of classifying rivers.