2.8: Sediment
- Page ID
- 14255
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)When regolith is mobilized or entrained, by flowing water, or by the wind, or by moving glacier ice, it is called sediment, because, as the term implies (the Latin verb sedere means to sit), it eventually comes to rest again as a deposit. Here I will touch upon only a few aspects of sediments. You will hear much more about sediment movement, and sediment deposits, in later chapters, especially the chapters on rivers, on glaciers, and on coasts.
One of the characteristic things about sediment is that it tends to become fractionated, by size, during transport. The basic reason is that a given transporting agent, like water or the wind, moving at a given speed, can move sediment particles up to a certain size but no larger—although another important effect is that the water or the wind moves finer particles faster than coarser particles. The end result of such fractionation is that the sorting of the particles in the deposit is usually much better than in the original material that was the source of the transported material. (The sorting of a sedimentary deposit describes the spread of particle sizes around the mean particle size: in a well sorted sediment the particle sizes cluster closely around the mean, whereas in a poorly sorted sediment the particle sizes range widely around the mean.)
For convenience and clarity in communication, sedimentary geologists have adopted a series of terms for sediment particles over the entire range of particle sizes (Figure 2-21). Such a subdivision is called a grade scale. It’s based on powers of two, so it’s geometric (or, what comes out mathematically to be the same, logarithmic), rather than arithmetic, for reasons that are obvious but not easy to justify: for example, the difference in behavior between a 1 mm particle and a 2 mm particle is bound to be far greater than the difference in behavior between a 101 mm particle and a 102 mm particle, right? You can see from Figure 1-20 that in geoscience such things as mud, silt, sand, and gravel are not just qualitative concepts but precisely defined entities!
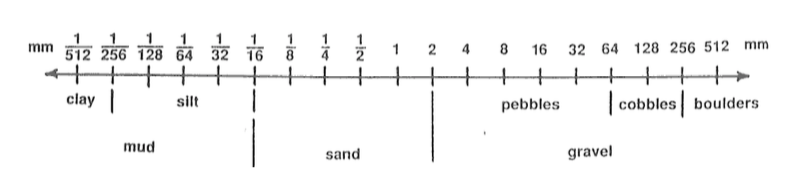
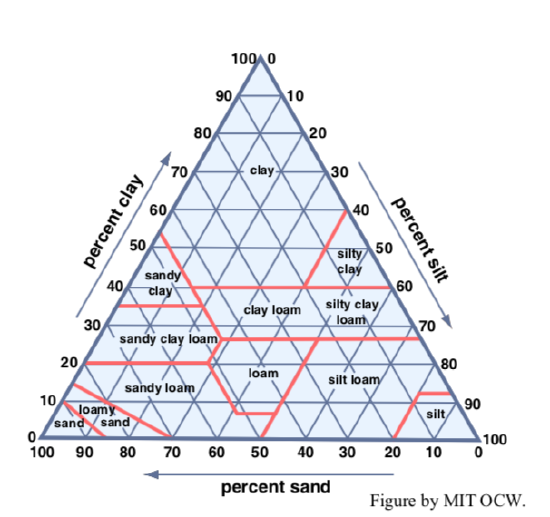
The logic behind the grade scale in Figure 2-20 is fine for well-sorted materials, but how about poorly sorted materials? Various classifications have been proposed, but the one that’s in most common use (with some variations) among regolith specialists is shown in Figure 2-21. Here you see what “loam” (so commonly pronounced “loom” locally!) really is, in the technical sense.
Advanced Topic: Measuring Sediment Size
1. Scientists or engineers who are responsible for studying surface deposits usually have to measure or characterize, in some way, the distribution of particle sizes. For large particles, in the gravel size range, it’s possible to measure the size of each piece separately, with calipers, although that’s a challenging endeavor unless the particles is very well rounded, and even then the matter is not entirely straightforward (as it would be if the particles were perfect triaxial ellipsoids). For smaller particle sizes, in the silt to fine pebble range, the classic way of measuring particle size is by sieving.
2. Here is how particle size is measured by sieving:
- make up a stack of special sieves, from coarsest on the top to finest on the bottom, with a lid on the top and a pan on the bottom;
- put a small sample of deposit in the top sieve;
- shake the stack until the particles have found their size bin;
- weigh each of the fractions on a balance;
- compute, for each sieve size, the total weight percent finer than that size;
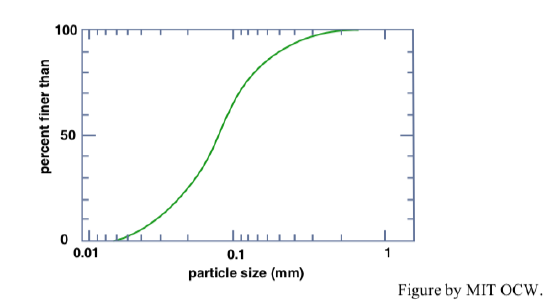
- plot the results on graph paper with size along the horizontal axis, logarithmically, and, along the vertical axis, weight percent finer than, from 0% to 100%, arithmetically.
An example of the result, called a cumulative distribution curve (common in many other applications of statistics as well), is shown in Figure 2-22.
3. The problem with sieving is that it works well only for sizes down to about 0.05 mm. For sediment finer than that, there are various techniques that involve suspension of the sample in water, settling of that suspension, and sampling during settling. The basic principle is that the coarser sizes settle faster than the finer sizes. Devices that are used to measure fine particle sizes in this way are called settling tubes. The governing principle is that each particle has a terminal fall speed that is a function of its size. In recent years, a variety of more sophisticated instruments have been developed to measure particle size, either optically or sonically, by sensing each particle as it passes through a thin tube in suspension. Such instruments are now obtainable “off the shelf”. There is still the tricky matter of intercalibrating between results from such instruments and traditional settling tubes.


