11.05: Polariscope

The polariscope may be one of the most underestimated tools in gemology. Most gemologists use it to quickly determine if the stone at hand is isotropic or anisotropic or, at best, to determine the optic character of gemstones. With some small additions, one can determine both optic character and the optic sign of a gemstone. It is also the preferred tool -- next to the microscope -- for separating synthetic amethyst from its natural counterparts (although with recent synthetics that may prove difficult).
In addition, the polariscope may be very useful for distinguishing solid inclusions from negative inclusions as well as for spotting polysynthetic twinning.

Basic

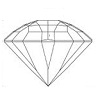
Figure \(\PageIndex{1}\): The polariscope

A polariscope uses polarized light for gem identification. It consists of two polarized filters, one on the top and one on the bottom of the instrument as seen in the picture to the right. Both the polarizer and the analyzer have their own vibrational planes. When the vibrational plane of the polarizer is at right angles to the vibrational direction of the analyzer, the field between them remains dark. This position is known as the "crossed position". In this position, gems can be tested to determine if they are:

• isotropic
• anisotropic
• anomalously double refractive or an
• anisotropic aggregate

The polarizing filters of this instrument are made of polarizing plastic sheets (polyvinyl alcohol containing dichroic molecules - stretched polymers). Older models were created with microscopically oriented crystals of iodoquinine sulfate (herapathite) or tourmaline plates.

Operation of the polariscope and possible observations

With the polarizer and analyzer in the crossed position, turn on the light source and place the gemstone on the rotating platform just above the polarizer (this platform might not always be present, in which case you use your tweezers).
Observing the gemstone through the analyzer while slowly turning the stone will give you 4 possibilities.

1. The stone appears dark throughout a 360° rotation.

The stone is isotropic (single refractive).

2. Throughout a 360° rotation the stone blinks 4 times, light and dark.

The stone is anisotropic (double refractive).

3. The stone will appear light all the time.

The stone is a microcrystalline or cryptocrystalline aggregate (like, for instance, chalcedony).

4. The stone will show anomalous double refraction (ADR).

It is isotropic (single refractive).

The first 3 behaviors should pose no problems for the inexperienced user, but the latter (ADR) can be misinterpreted and cause one to think the stone is double refractive.

Video presentations

Video \(\PageIndex{1}\): Video illustrating the use of the polariscope to determine optic character

Video \(\PageIndex{2}\): Video showing the behavior of double refractive stones and ADR under the polariscope

A possible solution for overcoming the confusion when one suspects ADR is to orientate the stone in its lightest position and then quickly turn the analyzer 90°. If the stone becomes noticeably lighter, it means the gemstone is single refractive and is exhibiting ADR. If it stays more or less the same, the stone is double refractive.

Red stones that are out of the limit of the refractometer (OTL) may be especially difficult to distinguish with the polariscope due to ADR. Some stones in this category are ruby, red spinel and red garnets.

Note

It should be noted that the gem being examined should be transparent to translucent so light can pass through it. If you put a piece of floor tile under the polariscope it would remain dark, but that doesn't mean the tile is single refractive. It just means light can’t pass through it.

An anisotropic gemstone can have one direction or two in which it will stay dark throughout lateral rotation. These directions are the optic axes of the gemstone. Uniaxial stones have one optic axis, biaxial gemstones have two. No double refraction occurs along the directions of optic axes.
Because there may be more than one direction in which some gemstones remain dark, it is useful as a confirmation to view the stone under a different angle when it stays dark.

Advanced

Polarizing microscope

With the aid of a few polarizing sheets, one can turn the gemological microscope into a polarizing microscope for less than USD 30.00.
Simply lay one of the sheets over the transmitting light source and tape the other one in the crossed position below the optics (or find your own way of doing something similar).
This enables us to distinguish between solid and negative crystal inclusions and many other internal features a gemstone might have.

A lot of the following discussion involves such a setup, although most of it can be achieved with the usual gemological polariscope as well.

Conoscopy

Figure \(\PageIndex{2}\): Conoscope

In gemology, we use a conoscope (a strain-free acrylic or glass sphere on a rod) to determine optic character (uniaxial or biaxial) in anisotropic gemstones. The conoscope creates a 2-dimensional image of the 3-dimensional interference in a mineral.
Although determining the optic character with a conoscope is a fairly easy procedure, finding the interference figure itself is not. The interference figures always appear around the optic axes of minerals.

The simplest way to find an interference figure is to rotate the stone under the polariscope, in every possible direction, while looking down the analyzer until one sees a small flash of colors appear on the surface of the gemstone. When that flash of colors is found, fix the stone in that position and hover your conoscope slightly over it. Now, while still looking through the analyzer, you should see the color flash transform into a rounded 2-dimensional image.
This image in uniaxial stones will appear different from the image in biaxial stones, each having its own characteristic pattern.

Using an immersion cell along with the polariscope may enable you to find the flash figures more rapidly.

Due to enantiomorphism, quartz will give a typical uniaxial image but with a large "target" in the middle. That is what is named a "bull's eye" and is typical for quartz (both natural and synthetic).

Because anisotropic minerals appear to be single refractive when viewed down the optic axis, another technique for finding the optic axis can be used. View the stone under the polariscope from all sides to find where the gemstone does not blink light and dark on lateral rotation. That will be the optic axis.
Remember that uniaxial minerals have one optic axis while biaxial gemstones have two optical axes.

Many polariscopes for gemological purposes come with a rather large conoscope that can be swiveled like a gemstone holder. Although one can get reasonably nice images with them, a conoscope rod is preferred and the smaller the sphere, the sharper the image. For the very small spheres, one will need magnification to observe the interference figure.

Interference figure nomenclature

For clarity, the nomenclature of interference figures should be understood. Luckily this is not too difficult.

In uniaxial stones, the "melatope" indicates the center of the dark cross and is the direction of the optic axis (looking down the optic axis).
The dark cross is actually made up of two L-shaped "isogyres" that will always stay in the same position in uniaxial stones.
The colored concentric fringes are named "isochromes".

Biaxial minerals have two optic axes, hence they have two "melatopes" that are in the center or the isogyres.
Again the dark cross is made up of two brushes, named "isogyres".
The colored concentric fringes are named "isochromes".

When the biaxial interference figure is laterally turned, the isogyres detach and transform into hyperbolas. The maximum curvature of these hyperbolic isogyres is at 45° rotation.
The distance between the two melatopes is dependent on the "2V" value of the mineral. When this 2V value is large (about 40-50°), the two isogyres will rarely ever be seen in one image. This also depends on the "numerical aperture" of your microscope.
No knowledge of "2V" or "numerical aperture" is needed for our discussion.

Retardation

In mineralogy, retardation means that one refracted ray of light is lagging behind another ray of light.
When light enters an anisotropic (double refractive) gemstone, it is split into two rays -- a fast ray and a slow ray. Because the fast ray travels faster through the gemstone it will be ahead of the slow ray. When the slow ray leaves the gem, the fast ray would have already traveled an extra distance outside the gemstone. That extra distance is known as "retardation" and is measured in nm (nanometers).

Through a series of calculations, it is shown that this retardation is dependent on the thickness and birefringence of the gemstone.

When the stone is placed between two polarizing filters (a polariscope), the two rays combine at the analyzer and either interfere with each other or cancel each other out, depending upon whether the rays are in phase or out of phase. This produces the typical interference colors.
These colors show a distinct pattern and, again, depend on the thickness and birefringence of the material.

As the thickness of the gemstone increases, the colors shift toward the right.
This knowledge can be useful in gemology as one could also add another mineral on top of the gemstone to mimic increased thickness and thus create a shift in colors when viewed through the conoscope. This shift can either be to the left or to the right.
When the slow ray of the gemstone and the slow ray of the added mineral align, the shift will be to the right. This will create an addition in color on the Newton Color Scale. When the slow ray of the gemstone and the fast ray of the added mineral align, the shift will be to the left and will create a subtraction in color.

When, for instance, a gemstone would create a retardation of 550nm, the starting spectrum would be on the boundary of the first order and second order and go from magenta to blue to blue-green to yellow to red. Then if a mineral with a retardation of 137nm is added, and if the slow ray of the gemstone aligns with the slow ray of the added mineral, the starting color would be blue (at 687nm) instead of magenta.
On the other hand, if in the same example the slow ray of the gemstone would align with the fast ray of the added mineral, there would be a subtraction, and then the starting color would be (550-137) 413nm -- yellow-orange.

Retardation Plates

Normally, we don’t know what the slow ray or the fast ray is for a particular gemstone nor for the added mineral, so this knowledge is of little use because of these two uncertain variables. To remove this uncertainty, "retardation plates" are made.

Retardation plates (as those added minerals are known) have a known retardation, and the vibrational directions of the slow and fast rays are known. This can help us determine the optic sign in gemstones.

Mineralogists generally use 3 kinds of retardation plates:

• quarter wave plates (with a retardation of 137nm) - made from mica
• full wave plates (with a retardation of 550nm) - made from gypsum
• quartz wedges (with an increasing retardation of 0 to 550nm) - made from quartz

All of the above plates can be very expensive since they are usually designed for petrographical microscopes that require special slots in the microscope. Fortunately, modern-day technology has created anisotropic plastic substitutes that cost little and can be held between your fingers. These plastic plates can be used in conjunction with the standard polariscope or with an adapted gemological microscope where polarizing filters are placed just above the light source at the base and just below the optics (you can use tape to hold them in place).
The latter is a setup that transforms your microscope into a polarizing microscope, at low cost, with the great benefit of magnification.

Those plastic retardation plates can be obtained from various sources (like the Daly/Hanneman simulated quartz wedge from Hanneman Gemological Instruments) at low cost. If you are intent on buying a plate, make sure you know how the fast and slow rays are orientated. With a stone of known optic sign you can determine that yourself though.

Figure \(\PageIndex{3}\): Typical polariscope setup with conoscope and retardation plate

Determining optic sign with the use of retardation plates

Determining the optic sign in anisotropic gemstones should pose few problems with the aid of one of the retardation plates. The real challenge, however, is finding the interference figure.
All images below are conoscopic images (with the conoscope in place).

The plates should be placed directly under or directly above the gemstone. When above the gemstone, the plate should be placed between the stone and the conoscope.

The plate itself should be inserted at a 45° angle to the polarizer and analyzer as illustrated in the images below.

Full wave plate on uniaxial stones

Figure \(\PageIndex{4}\)

For convenience, the image above has the area of interest marked, which is the area just around the center of the interference figure (the white circle).
That area is divided into 4 quadrants.

Figure \(\PageIndex{5}\)

The full wave plate is inserted from bottom right to top left at an angle of 45°.
In the direction marked "slow", the slow ray of the wave plate travels. The fast ray travels in the direction of the length of the plate.

When one looks closely (click the image for a clearer, larger view) the colors in the quadrants change.
Quadrants 1 and 3 turn more or less blue (here addition of color occurred), while in quadrants 2 and 4 the colors change to predominantly yellow-orange (here subtraction occurred).

This indicates a uniaxial gemstone with a negative optic sign.

Figure \(\PageIndex{6}\)

The wave plate removed for a clearer view (this is for illustration only and will not work in practice).

The quadrants 1 and 3 clearly have a shift of color to blue. Also, notice that the dark cross (the isogyres) now have a magenta color. This is caused by the magenta color of the full wave plate under crossed polars (the color in natural daylight is transparent white).

Figure \(\PageIndex{7}\)

The opposite of the above. Quadrants 1 and 3 show a yellow-orange color, while quadrants 2 and 4 turn blue.

This indicates a uniaxial stone with a positive optic sign.

Full wave plate on biaxial stones

The full wave plate is best operated on biaxial gemstones which are orientated in a way that the isogyres are at 45° to the polarizing filters.
We concentrate on the areas just around the melatopes in the isogyre.

The areas in question (on the convex and concave sides of the isogyre) are more or less gray. These colors will change when a full wave plate is inserted.

Ideally, a biaxial gemstone will show both isogyres in one image, but alas that is not always the case.
As with the uniaxial stones, the wave plate is inserted at 45° to the polarizing filters.

Figure \(\PageIndex{8}\): Rotation of 45° with isogyres at maximum curvature. Colors on convex and concave sides are of 1st order gray.	Figure \(\PageIndex{9}\): Inserted full wave plate creates blue colors on the convex sides of the isogyres and yellow on the concave sides. This indicates a negative optic sign.	Figure \(\PageIndex{10}\): The wave plate creates yellow 1st order colors on the convex sides and 2nd order blue on the concave sides of the isogyres. This means the stone has a positive optic sign.

Most of the time, you will see only one of the isogyres at one time. When you do, rotate it to maximum curvature as seen in the images below.
When the 2V values are high (close to 90°), the curvature is almost impossible to recognize and you will have a hard time trying to see which direction it curves to (up or down). At other times, the isogyre is a very fuzzy hyperbole which gives the same troubles. With practice, you will be able to recognize it more rapidly.

Same situation as above with the focus on one of the isogyres.
Figure \(\PageIndex{11}\): Without full wave plate inserted	Figure \(\PageIndex{12}\): Optic sign is negative	Figure \(\PageIndex{13}\): Optic sign is positive

Quarter wave plates

Quarter wave plates work in a similar way as full wave plates but will produce different images.
These plates are traditionally made from thin sheets of mica with a retardation of approx. 137 nm (a quarter of a full wave plate). Plastic simulators are available and even cellophane the florist wraps flowers in can act as a quarter wave plate. In the latter case, the fast ray of the cellophane is in the direction of the roll.

Figure \(\PageIndex{14}\)

As discussed previously with the full wave plate, the conoscopic image of this uniaxial stone is divided into 4 imaginary quadrants.

Figure \(\PageIndex{15}\)

The quarter wave plate is inserted at a 45° angle to the polarization filters and two black spots appear in the 1st and 3rd quadrants. This indicates a uniaxial gem with a positive optic sign.
For uniaxial negative stones, the black spots will be seen in the 2nd and 4th quadrants.

As before, the fast ray travels along the length of the wave plate.

Figure \(\PageIndex{16}\)

Same image as above, but enlarged and the waveplate removed for a better view (for illustration purposes only).

Airy Spirals

Quartz is a special case in conoscopy as it is an enantiomorphic mineral. This means that the crystal structure spirals either to the left or to the right along the direction of the optic axis, resulting in the typical "bulls-eye" under the conoscope.
With the addition of a quarter wave plate this bulls-eye transforms in two spirals which spiral either to the left or right, showing their "handedness". These spirals are named "Airy Spirals" after Sir George Biddell Airy who first described this in 1831.
Some quartz (especially amethyst) is both right-handed as left-handed due to Brazil twinning. As a result, it will not show the typical bulls-eye but a combination of the left and right Airy Spirals (4 spirals in total) under the conoscope. This may look very much like the classical bulls-eye in faceted stones, especially when the optic axis cuts through small facets. One doesn't need a retardation plate to observe the latter.

Figure \(\PageIndex{17}\): Bulls-eye in monochromatic light, either left or right-handed.	Figure \(\PageIndex{18}\): With the quarter wave inserted the Airy Spirals spiral to the left. This indicates left-handed quartz.	Figure \(\PageIndex{19}\): With the quarter wave inserted the Airy Spirals spiral to the right. This indicates right-handed quartz.

Quartz Wedges

With quartz wedges, the movement of the isochromes becomes important in determining optic sign.

Quartz wedges are wedges made of the mineral quartz. The increasing thickness of the wedge provides for a continues range of retardation between, usually, 0 and 550 nm. This means that the wedge can be used as a quarter wave plate as well as a full wave plate. The wedge is not used as such mostly in gemology; instead, it is used to hover over an interference pattern and to determine optic sign by observing the movement of the isochromes.

Figure \(\PageIndex{20}\): Quartz wedge in top view between crossed polarizers and side view with the relative retardation wavelengths indicated

As real quartz wedges are very expensive, small and mainly made for use in petrographical microscope this technique was not practiced a lot by gemologists. Luckily one can now buy inexpensive (around USD 40.00) plastic sheets that can do the same. The first (or at least the first reported) one who used these polystyrene plastic simulated quartz wedges was Pat Daly, FGA from England. He constructed the first ones from the clear boxes in which the OPL spectroscopes are shipped in.
Dr. Hanneman made this tool semi-popular and the plastic quartz wedge is now named the "Hanneman-Daly wavelength modifier & simulated quartz wedge".

A few hours of practice should be enough to master this technique and it may come in very handy when you can perform little other tests.

Uniaxial stones and the quartz wedge

Figure \(\PageIndex{21}\)

We now divide the whole conoscopic interference pattern into 4 sections (not just the center).

Although these images show perfect computer generated interference patterns, one can easily find the optic sign on a partial image.

When you don't know the orientation of the polarizer and the analyzer of your polariscope, all you need to do is look at the cross in the uniaxial interference figure. The crosses indicate the vertical and horizontal alignments (also indicated by the white lines).
If the dark cross is not horizontal/vertical that means neither are your polarizers. That isn't very important, just make sure you adjust the position of the wedge (or any other wave plate) accordingly.

Figure \(\PageIndex{22}\)

The simulated Hanneman-Daly quartz wedge is inserted as shown, 45° to the polarizer and analyzer, and one needs to slowly move it along the direction of the arrow over the pattern (from quadrant 3 to quadrant 1). The arrow is only there to show the direction of movement, it isn't on the wedge itself.

It is vital that you insert the wedge in this direction and at that angle or you will not get the results illustrated in the next two images. If you do choose to insert the wedge from the left lower corner, the results are reversed.

One must now pay good attention to how the isochromes (the colored rings) behave. In two, opposite, quadrants they will move outward while in the other quadrants they will move inwards. It is this movement that determines if the optic sign is + (positive) or - (negative).

Figure \(\PageIndex{23}\)

In this image (where the wedge is not shown for better illustration) the arrows in quadrant 2 and 4 move outwards and the isochromes will appear to do the same. This indicates a negative optic sign under the above conditions.
At the same time, the colored rings in the 1st and 3rd quadrants will move inward.

If you now move the wedge back from quadrant 1 to 3, the reverse is observed.

Figure \(\PageIndex{24}\)

Here the isochromes in the 1st and 3rd quadrants will move out and the isochromes in the 2nd and 4th will move inward. This means that the stone is uniaxial +. A positive optic sign.

As with the negative optic sign image, when you move the quartz wedge in the opposite direction (back to quadrant 3), the movement of the isochromes is reversed.

Biaxial stones and the quartz wedge

Figure \(\PageIndex{25}\)

For biaxial stones, this technique becomes more difficult as one needs to find or the maximum curvature of the isogyres, or know where the 2 melatopes are.
The best approach is to turn the stone so it will show maximum curvature. This is done by laterally turning the stone so the isogyres are at 45° to the polarizer and analyzer as in this image.
The way one rotates the stone is not important. Here the interference pattern has the isogyres in the lower right and upper left, but they could be in the lower left and upper right as well. For right-handed people, this setup is probably best as one needs a steady hand to hover the quartz wedge.

It should be noted that this image is an ideal one (two isogyres seen), which is rarely the case.

Figure \(\PageIndex{26}\)

In most cases, you will see only one isogyre and that is all we need for the quartz wedge to work.
Here the curvature is easily seen and from that, we know where the other melatope is located.

Figure \(\PageIndex{27}\)

We now insert the simulated quartz wedge over the stone (with the conoscope in place) from the most concave side of the isogyre towards the most convex side and we need to observe how the isochromes behave.
Hover it back and forth over the interference pattern, but pay attention only to the change in the forward direction. That is the direction indicated by the arrow. The arrow is not actually on the wedge, it is there to show the direction of motion.

Figure \(\PageIndex{28}\)

Here the wedge is removed for illustration.
If the isochromes move towards the other melatope (the one that is outside the view), the stone is biaxial with a positive optic sign.

When one hovers the wedge back and forth over the image, you will see the isochromes moving away and back. Pay attention only to the reaction of the forward motion.

Figure \(\PageIndex{29}\)

When the isochromes move away from the other, out of view, melatope, the stone is biaxial with a negative optic sign.

Another way of thinking could be:

• if the isochromes move along the direction of the movement of the quartz wedge, it indicates a positive optic sign
• if the isochromes move in opposite direction, the stone has a negative optic sign.

Figure \(\PageIndex{30}\)

In some cases, you will see an image resembling what the GIA calls a "bowtie". It can be very hard to see what the concave and the convex sides of the isogyres are.
Although this indicates a biaxial optic character, the optic sign is very hard to obtain from this. This happens when the "2V" value is large (larger than 50°).

When you observe this image carefully, you will notice that the curve endpoints are at the right.

Tips and tricks

Honey

On page 64 of Ruby & Sapphire (1997), Richard Hughes shows a Thai/Cambodian sapphire with tiny droplets of methylene iodide to show the uniaxial interference pattern. The small drops act like tiny conoscopes and when applied correctly, you will have an extra hand free (the one that usually holds the conoscope). Hughes also suggested, through personal communication, the use of a small droplet of honey which works very well.
This technique works best with magnification as the obtained figures are very small.

Monochromatic light

With monochromatic lighting (such as a yellow sodium filter of your refractometer), the interference pattern may stand out more clearly.

Poor man's polarizing microscope

Buy two polarizing sheets (50 x 50 mm) and tape them in crossed position on your microscope. One just above the light source and the other just below the optics. This setup will give you a polariscope with the great benefit of magnification and you will find interference figures much easier to interpret.
Such a setup should not cost you more than USD 30.00 (probably less) if you already have a suitable gemological microscope.

Lucky woman's retardation plate

Florists usually have cellophane plastic in which they wrap their flowers. This cellophane may work as a quarter wave plate. The length of the roll is the fast ray, the cutting edge is the direction of the slow ray.
Simply cut a small piece from it and lay it on the polarizer (between the lower polarizing sheet and the stone) after you found the interference figure (at a 45° angle to the polarizer directions of course).
Other kinds of cellophane like ScotchTape may also work.

Immersion

Sometimes it is very hard to find interference figures. Place the stone is a shallow dish of water (or baby oil) and rotate the stone slowly in it. You will find the interference flashes more easily in certain circumstances.
The dish should be placed between the crossed polars.

Related topics

• Polarization
• Pleochroism
• Dichroscope

Sources

• Guide to Affordable Gemology (2001) - Dr. W. Wm. Hanneman, PH.D.
• Introduction to Optical Mineralogy 3rd edition (2003), Prof. W.D. Nesse
• Ruby & Sapphire (1997) - Richard W. Hughes
• Gem Identification Made Easy 3rd edition (2006) - Antoinette Matlins, A.C. Bonanno
• Edelsteinbestimmung (1984) - Godehard Lenzen, Birgit Günther, Walter Grün ISBN 3-9800292-2-0

External links

• Conoscopy Illustrated (with a special acknowledgment for donating many of the conoscopic images)
• Identification of CVD Synthetic Diamond
• Patent for herapathite polarizers
• Patent description of PVA polarizers
• Virtual Polarizing Microscope