4.2: Paleoclimate: Evidence from the geological record
- Page ID
- 11087
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)There are many different proxies for temperature; all have advantages and drawbacks. Some are physical, like the temperature of water in deep boreholes—water that has been isolated from the surface for a long time and reflects a long history of temperature. Some are biological, like the width and density of tree rings. All these are local or at best regional metrics; there is no global “paleothermometer.”
One particularly useful proxy relies on the physics of condensation and evaporation of water. Water (H2O) is made of one oxygen atom and two hydrogen atoms. A standard oxygen atom consists of a nucleus with 8 protons and 8 neutrons, surrounded by a cloud of 8 electrons. But some oxygen atoms have 9 or 10 neutrons in their nucleus. These variants are called isotopes. Standard oxygen, with 8 neutrons, called 16O to denote the number of protons and neutrons, is by far the most abundant isotope, followed by 18O with 8 protons and 10 neutrons. A tiny percentage of water contains this heavier oxygen isotope, and it turns out that the ratio of the heavy to the light isotope in water contains is a very useful metric.
Ocean water has a particular oxygen isotope ratio. But when seawater evaporates, its molecules containing the lighter isotope evaporate slightly faster than the molecules containing the heavier isotope. So, water vapor is “lighter” than seawater, meaning the ratio of heavy to light isotopes is smaller. Likewise, when the evaporated water begins to condense into clouds, molecules made of the heavier isotope condense first, so that as the cloud rains out, the water vapor left behind becomes progressively “lighter,” as does the precipitation that subsequently forms from it. So the farther away the water vapor is from its source, the “lighter” it is. By “farther” we really mean “colder,” since the amount of water vapor in a cloud falls rapidly as the air cools.
Likewise, standard hydrogen atoms in water have one proton and no neutrons, but a few atoms have one neutron, and there are even a few with two neutrons. A hydrogen atom with one neutron is called deuterium, and the ratio of deuterium to normal hydrogen in water can also be used as a paleothermometer.
Thus the isotope ratios in rain and snow reflect the temperature of the cloud in which the rain or snow formed. In places like Greenland and Antarctica, much of the snow that falls accumulates and is progressively compacted by the weight of the snow on top of it, eventually forming ice. The ice is thus progressively older with depth in these ice sheets. Scientists drill down to collect solid cylinders of ice—ice cores—which they can analyze for many properties of the ice, including its isotopes, as a function of depth, or equivalently, age. The isotope ratios give a measure of the temperature of clouds that produced the snow originally. Modern measurements of the isotope ratios of recent snow show that they are highly correlated with surface air temperature, which is in turn correlated with the temperature of clouds above it. Thus we can use the isotope ratios as paleothermometers.
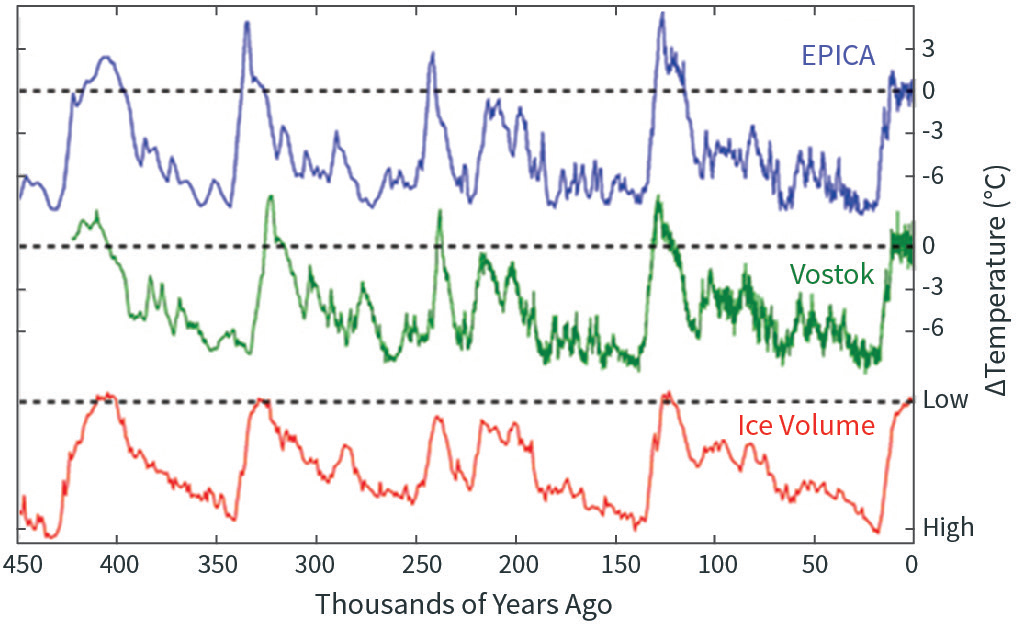
Figure \(\PageIndex{1}\): Temperature inferred from the deuterium ratios in two Antarctic ice cores (green and blue curves), and ice volume inferred from the oxygen isotope ratios of marine microfossils in ocean floor sediments (red curve). Note that the ice volume curve is flipped, so that high is on the bottom and low on the top, to make it easier to compare with temperature. Source: https://commons.wikimedia.org/wiki/F...?uselang=en-gb.
Figure \(\PageIndex{1}\) shows the record of temperature inferred from two ice cores in Antarctica, going back 450,000 years, as well as the volume of ice on the planet. You might be wondering how we know how much ice there was on Earth 450,000 years ago. Recall that as seawater evaporates, the lighter isotopes evaporate faster, and thus ice sheets, which form from condensed water vapor, have a higher concentration of lighter isotopes than seawater. As ice sheets grow, the heavier isotopes get left behind in the ocean, and so the ratio of heavier to lighter isotopes in seawater steadily increases. Thus the isotopic composition of seawater is a measure of how much land ice there is on the planet. Marine microorganisms incorporate these isotopic signatures in their shells, and when they die some of them settle to the seafloor, where they get incorporated in sediments. We can analyze these sediment cores to get isotope ratios as a function of depth, and by other means determine the age of the sediments. Thus we can obtain a record of global ice volume with time.
You can see in Figure \(\PageIndex{1}\) that the lower the temperature, the higher the volume of ice on the planet, and vice versa. This makes sense! That the two curves—obtained from entirely different sources of data—agree so well testifies to the basic quality of the data underlying each.
It is plainly obvious that on the 100,000-year time scale, temperature is cyclic. These cycles are the great ice ages and interglacial periods, and the right edge of Figure \(\PageIndex{1}\) shows that we are in an interglacial period right now. The last ice age ended about 10,000 years ago—a geologic blink of the eye.
The figure also shows that the Antarctic temperature varied about 9 K (16 °F) between the warmest and coldest periods. Other proxy estimates, models, and theory indicate that the tropics varied quite a bit less, so that the global mean temperature probably varied by about 5 K (9°F) between peaks and valleys.

