4.2: Structures Formed by Unidirectional Currents

We moved from sediment transport directly into sedimentary structures because these structures are imparted to the sediment during the transport process and tell us about what was going on at the time of deposition. While transport is happening, the sediment may be organized into a three-dimensional bedform. If preserved (or partially preserved), that bedform becomes a sedimentary structure in the rock. Sometimes the two go by the same name and sometimes they don’t; when we first introduce the terms we will try to make it clear what we are referring to.

Bedform Stability Diagram

Figure \(\PageIndex{1}\): Bedform stability diagram showing grain size versus flow velocity for unidirectional currents in water at 10 ⁰C and 0.25-0.40 m deep (Page Quinton via Wikimedia Commons; CC BY-SA 4.0). Diagram after Southard and Boguchwal (1990) and Potter and Pettijohn (1977).

Before we launch into a more detailed description about the different types of sedimentary structures that form in response to unidirectional flows, it is worth taking a moment to point out that:

• Flume studies reveal that you can predict what bedform will be present/stable given a certain grain size and flow velocity (provided that you hold all other variables constant)
• A systematic relationship exists between bedforms and that if you change the velocity, a systematic progression in bedforms will occur. 

 

Figure \(\PageIndex{2}\): Bedforms and sedimentary structures formed in response to unidirectional flows. In all cases the flow direction was/is from right to left. (Page Quinton via Wikimedia Commons; CC BY-SA 4.0).

Lower Plane Beds

Relatively course-grained sand (above about 0.6 mm diameter) will not form ripples under low flow velocities. Instead, rolling and tumbling of grains will form simple horizontal layers known as lower plane beds (term refers to the bedform and resulting sedimentary structure).

Asymmetric Ripples & Ripple Cross-Lamination

Asymmetric ripples (aka current ripples) are bedforms that are less than 10 cm tall; they have a pronounced asymmetric shape with a gently dipping upstream (stoss) side and a relatively steeply dipping downstream (lee) side. They form as sediment, and the bedform itself) migrates in a downstream direction. Silt- and sand-sized particles move up the stoss side through bedload transport and then accumulate as downstream-dipping layers on the sheltered stoss side.

Asymmetric ripples are only rarely preserved in their three-dimensional form, instead the downstream dipping laminae deposited on the stoss side are preserved as ripple cross-laminae. Ripple cross-laminae are just smaller versions of cross-beds and consist of subhorizontal layers with internally dipping laminae that are tangential with the bottom of the bed and truncated at a higher angle at the top of the bed. If the sedimentation rates is very high, climbing ripples may form, these features preserve both the ripple crest and the downstream-dipping laminae.

Figure \(\PageIndex{3}\): Ripples and ripple cross-laminae all images from (Michael C. Rygel via Wikimedia Commons; CC BY-SA 3.0 or CC BY-SA 4.0). A) Modern asymmetric ripples exposed in the intertidal zone. Flow was from left to right; footprint near bottom center of the image for scale. B) Cross-sectional exposure of a ripple cross-laminated sandstone; Although not fully preserved in three dimensions, these structures are strongly aggradational and could be considered climbing ripples. C) Cross-section view of a ripple cross-laminated sandstone. Paleoflow was from left to right. D) Bedding plane exposure of ripple cross-laminated sandstone; individual cross-laminae are often covered with fine fragments of plant materials (black) and they dip into the bed toward the left indicating that paleoflow was from right to left.

Dunes and Cross-Beds

Given a constant grain size, an increase in velocity will cause ripples/lower plane beds to transition into dunes (a bedform). The main difference between ripples and dunes is size; by definition dunes are greater than 10 cm tall. Other than that, the processes and terminology are largely the same … they migrate in a downstream direction by deposition of inclined layers on the steeper downstream side.

Based on the extent and morphology, we can subdivide these bedforms into sinuous-crested dunes which have curved and often laterally discontinuous crests and straight-crested dunes with have a linear crest and may be continuous for tens of meters.

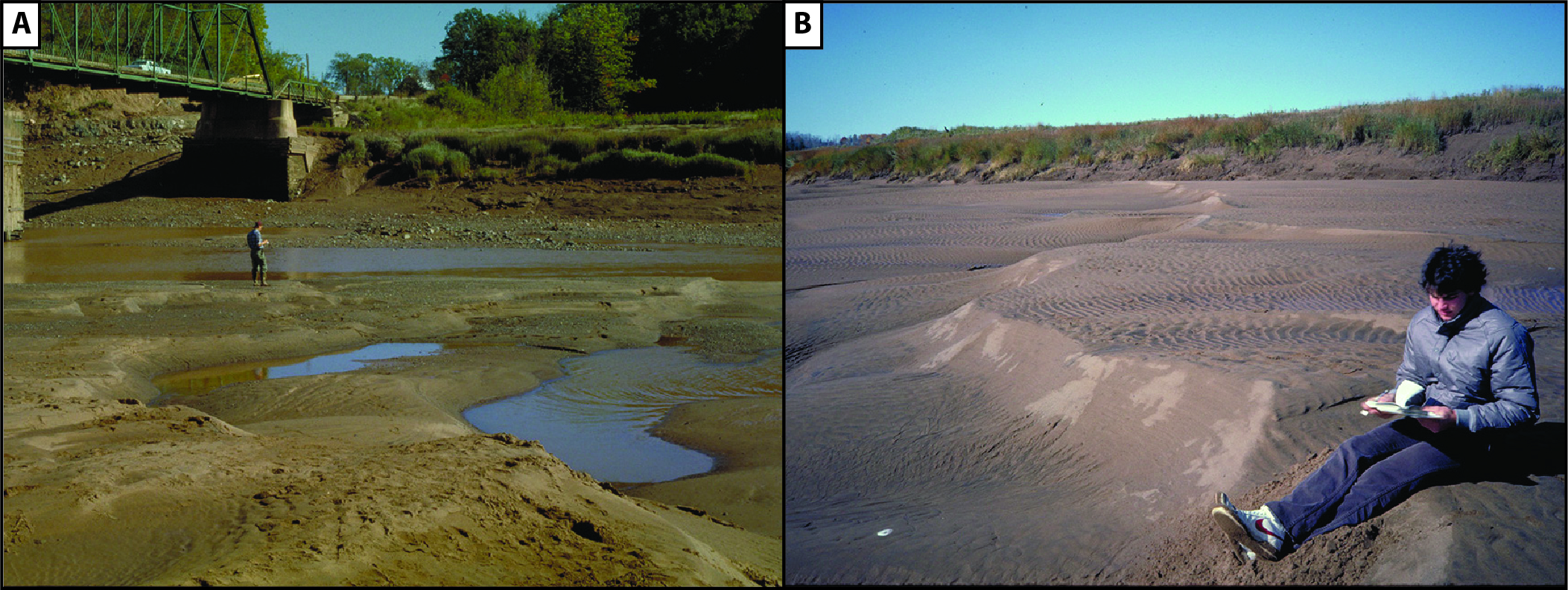Figure \(\PageIndex{4}\): Dune crest shape. A) Sinuous-crested dunes from the Kennetcook River. B) Straight-crested dunes from the Kennetcook River estuary (both images courtesy John Waldron via University of Alberta; CC BY-NC-SA 4.0).

Dunes are preserved as cross-beds, which have broadly horizontal bedding planes separating beds that have inclined laminae internally. Trough cross-beds are the product of sinuous-crested dunes. We are using the terminology of Potter and Pettijohn (1977) who differentiate trough and planar cros-beds based on the morphology of the bounding surfaces between individual foreset beds (individual cross-bedded layers). Trough cross-beds have curved bounding surfaces that appear u-shaped when viewed in an up- or down-flow direction and scoop-shaped when viewed perpendicular to flow direction. When viewed from above, foresets in trough cross beds appear u-shaped and open in a downstream direction. Tabular cross-beds are the product of straight-crested dunes. They too have downstream-dipping foresets but the bounding surfaces are much more planar and continuous than in trough-cross beds.

 

Figure \(\PageIndex{5}\): Photographs of cross beds. A) Cross-sectional view of trough cross beds; paleoflow is almost directly into the face. B) Slightly oblique bedding plane view of trough cross-beds; paleoflow is into the image toward about 11 o'clock. The u-shaped foreset laminae open in a downstream direction and dip downstream. C) Cross-sectional view of trough cross-beds; paleoflow is from right to left. Note that cross-laminae become tangential with the lower bounding surface and that there is modest relief along the bounding surface. D) Planar cross-beds with foreset laminae that intersect the lower bounding surface at a steep a steep angle (close to the angle of repose) and that the lower bounding surface is nearly planar. Paleoflow direction is from left to right. E) Planar cross beds with tangential foreset laminae but planar bounding surfaces. Paleoflow direction is from left to right. Although there is some difference of opinion about whether to use foreset or bounding surface morphology to classify cross-beds, the descriptive terminology doesn't ultimately matter all that much. F) Planar cross-beds with wedge-shaped bounding surfaces. Paleoflow direction is generally from left to right. Parts A, B, C, and E from Michael C. Rygel via Wikimedia Commons, CC BY-SA 3.0 or CC BY-SA 4.0; D from Anne Burgess via Wikimedia Commons, CC BY SA 2.0; E from James St. John via Wikimedia Commons, CC BY 2.0.

Upper Plane Beds and Laminated Sandstone with Primary Current Lineation

A continued increase in velocity will cause dunes to wash out and the formation of a flat sediment surface where individual grains are rolling or streaming along the bed. These horizontally laminated upper plane beds (bedform) are nearly identical to lower plane beds except they can occur in much finer grained sand and that bedding plane surfaces are ornamented with primary current lineation. This distinctive linear fabric forms parallel to paleoflow direction as sand grains align behind one another via micro-vortices (just how bicyclists draft behind one another when racing).

Figure \(\PageIndex{6}\): A) Modern primary current lineation in a modern beach sand. The linear fabric is caused by the alignment of sand grains. The accumulation of sand behind larger pebbles indicates that paleoflow was from top to bottom. B) Ancient primary current lineation on a bedding plane in a laminated sandstone. Paleoflow was either from top to bottom or bottom to top. (Michael C. Rygel via Wikimedia Commons; CC BY-SA 3.0)

Antidunes

As the name implies, antidunes (name for bedform and sedimentary structure) are, in many ways, the opposite of regular dunes. They form under fast shallow flow conditions (Fr > 1) and the bedform migrates in an upstream direction along with the standing wave that sits atop it (see video below). They do this as sediment is plastered onto the steeply-dipping upstream face of the bedform; the result is that laminae dip in an upstream direction. They’d be easy to confuse with regular cross-beds, but don’t fret because they are only rarely preserved because as flow velocity wanes the sediment is commonly reworked into upper plane beds and dunes. When preserved, they are closely associated with wavy- or undulatory laminae formed with the transition to upper plane beds.

Figure \(\PageIndex{7}\): Video of active antidunes and standing waves.

Figure \(\PageIndex{8}\): Possible antidunes and undulatory laminae formed in association with upper flow regime conditions (Michael C. Rygel via Wikimedia Commons; CC BY-SA 4.0).

Additional Resources

• Potter, P.E. and Pettijohn, F.J., 1977, Paleocurrents and Basin Analysis (2nd); Springer-Verlag, NY, 425 p. - https://link.springer.com/book/10.1007/978-3-642-61887-1
• Southard, J.B., and Boguchwal, L.A., 1990, Bed configurations in steady unidirectional water flows. Part 2. Synthesis of flume data, Journal of Sedimentary Petrology v. 60, no. 5, p. 658-679