16.11: Homework Exercises
- Page ID
- 10510
16.11.1. Broaden Knowledge & Comprehension
B1. a. Search for satellite images and movies for a few recent tropical cyclones. Discuss similarities and differences of the tropical cyclone appearance.
b. Same, but for radar images during landfall.
c. Same, but for photos from hurricane hunters.
B2. Search for web sites that show tropical cyclone tracks for: a. the current tropical cyclone season.
b. past tropical cyclone seasons.
B3. What names will be used for tropical cyclones in the next tropical cyclone season, for the ocean basin assigned by your instructor?
B4. How many NWP models are available for forecasting tropical cyclone track, & how are they used?
B5. What is the long range forecast for the number of tropical cyclones for the upcoming season, for an ocean basin assigned by your instructor. (Or, if the season is already in progress, how are actual numbers and intensities comparing to the forecast.)
B6. Find maps of “tropical cyclone potential”, “tropical cyclone energy”, “intensity”, or “sea-surface temperature”. What are these products based on, and how are they used for tropical cyclone forecasting?
B7. Search the web for photos and info on hot convective towers. How do they affect tropical cyclones?
16.11.2. Apply
A1. At 10° latitude, find the absolute angular momentum (m2 s–1) associated with the following radii and tangential velocities:
| R (km) | Mtan (m s–1) | |
| a. | 50 | 50 |
| b. | 100 | 30 |
| c. | 200 | 20 |
| d. | 500 | 5 |
| e. | 1000 | 0 |
| f. | 30 | 85 |
| g. | 75 | 40 |
| h. | 300 | 10 |
A2. If there is no rotation in the air at initial radius 500 km and latitude 10°, find the tangential velocity (m s–1 and km h–1) at radii (km):
| a. 450 | b. 400 | c. 350 | d. 300 |
| e. 250 | f. 200 | g. 150 | h. 100 |
A3. Assume ρ = 1 kg m–3, and latitude 20° Find the value of gradient wind (m s–1 and km h–1) for:
| R (km) | ∆P/∆R (kPa/100 km) | |
| a. | 100 | 5 |
| b. | 75 | 8 |
| c. | 50 | 10 |
| d. | 25 | 15 |
| e. | 100 | 10 |
| f. | 75 | 10 |
| g. | 50 | 20 |
| h. | 25 | 25 |
However, if a gradient wind is not possible for those conditions, explain why.
A4. For the previous problem, find the value of cyclostrophic wind (m s–1 and km h–1).
A5. Plot pressure vs. radial distance for the max pressure gradient that is admitted by gradient-wind theory at the top of a tropical cyclone for the latitudes (°) listed below. Use z = 17 km, Po = 8.8 kPa.
| a. 5 | b. 7 | c. 9 | d. 11 | e. 13 | f. 17 | g. 19 |
| h. 21 | i. 23 | j. 25 | k. 27 | m. 29 | n. 31 | o. 33 |
A6. At sea level, the pressure in the eye is 93 kPa and that outside is 100 kPa. Find the corresponding pressure difference (kPa) at the top of the tropical cyclone, assuming that the core (averaged over the tropical cyclone depth) is warmer than surroundings by (°C):
| a. 5 | b. 2 | c. 3 | d. 4 |
| e. 1 | f. 7 | g. 10 | h. 15 |
A7. At radius 50 km the tangential velocity decreases from 35 m s–1 at the surface to 10 m s–1 at the altitude (km) given below:
| a. 2 | b. 4 | c. 6 | d. 8 |
| e. 10 | f. 12 | g. 14 | h. 16 |
Find the radial temperature gradient (°C/100km) in the tropical cyclone. The latitude = 10°, and average temperature = 0°C.
A8. Find the total entropy (J·kg–1·K–1) for:
| P (kPa) | T (°C) | r (g kg–1) | |
| a. | 100 | 26 | 22 |
| b. | 100 | 26 | 0.9 |
| c. | 90 | 26 | 24 |
| d. | 80 | 26 | 0.5 |
| e. | 100 | 30 | 25 |
| f. | 100 | 30 | 2.0 |
| g. | 90 | 30 | 28 |
| h. | 20 | –36 | 0.2 |
A9. On a thermo diagram of the Atmospheric Stability chapter, plot the data points from Table 16-5. Discuss.
A10. Starting with saturated air at sea-level pressure of 90 kPa in the eye wall with temperature of 26°C, calculate (by equation or by thermo diagram) the thermodynamic state of that air parcel as it moves to:
- 20 kPa moist adiabatically, and thence to
- a point where the potential temperature is the same as that at 100 kPa at 26°C, but at the same height as in part (a). Thence to
- 100 kPa dry adiabatically and conserving humidity. Thence to
- Back to the initial state.
- to h: Same as a to d, but with initial T = 30°C.
A11. Given the data from Table 16-5, what would be the mechanical energy (J) available if the average temperature at the top of the tropical cyclone were
| a. –18 | b. –25 | c. –35 | d. –45 |
| e. –55 | f. –65 | g. –75 | h. –83 |
A12. For the previous problem, find the minimum possible eye pressure (kPa) that could be supported.
A13. Use P∞ = 100 kPa at the surface. What maximum tangential velocity (m s–1 and km h–1) is expected for an eye pressure (kPa) of:
| a. 86 | b. 88 | c. 90 | d. 92 |
| e. 94 | f. 96 | g. 98 | h. 100 |
A14. For the previous problem, what are the peak velocity values (m s–1 and km h–1) to the right and left of the storm track, if the tropical cyclone translates with speed (m s–1):
| (i) 2 | (ii) 4 | (iii) 6 | (iv) 8 | (v) 10 |
| (vi) 12 | (vii) 14 | (viii) 16 | (ix) 18 | (x) 20 |
A15. For radius (km) of:
| a. 5 | b. 10 | c. 15 | d. 20 |
| e. 25 | f. 30 | g. 50 | h. 100 |
find the tropical cyclone-model values of pressure (kPa), temperature (°C), and wind components (m s–1), given a pressure in the eye of 95 kPa, critical radius of Ro = 20 km, and Ws = –0.2 m s–1. Assume the vertically-averaged temperature in the eye is 0°C.
A16. (§) For the previous problem, plot the radial profiles of those variables between radii of 0 to 200 km.
A17. Use P∞ = 100 kPa at the surface. Find the pressure-head contribution to rise of sea level (m) in the eye of a tropical cyclone with central pressure (kPa) of:
| a. 86 | b. 88 | c. 90 | d. 92 |
| e. 94 | f. 96 | g. 98 | h. 100 |
A18. Find the Ekman transport rate [km3/(h·km)] and surge slope (m km–1) if winds (m s–1) of:
| a. 10 | b. 20 | c. 30 | d. 40 |
| e. 50 | f. 60 | g. 70 | h. 80 |
in advance of a tropical cyclone are blowing parallel to the shore, over an ocean of depth 50 m. Use CD = 0.005 and assume a latitude of 30°.
A19. What is the Kelvin wave speed (m s–1 and km h–1) in an ocean of depth (m):
| a. 200 | b. 150 | c. 100 | d. 80 |
| e. 60 | f. 40 | g. 20 | h. 10 |
A20. For the previous problem, find the growth rate of the Kelvin wave amplitude (m h–1) if the tropical cyclone tracks south parallel to shore at the same speed as the wave.
A21. Find the wind-wave height (m) and wavelength (m) expected for wind speeds (m s–1) of:
| a. 10 | b. 15 | c. 20 | d. 25 |
| e. 30 | f. 40 | g. 50 | h. 60 |
A22. For the previous problem, give the:
(i) Beaufort wind category, and give a modern description of the conditions on land and sea
(ii) Saffir-Simpson tropical cyclone Wind cate- gory, its corresponding concise statement, and describe the damage expected.
16.11.3. Evaluate & Analyze
E1. In Fig. 16.2, if the thin ring of darker shading represents heavy precipitation from the eyewall thunderstorms, what can you infer is happening in most of the remainder of the image, where the shading is lighter grey? Justify your inference.
E2. Thunderstorm depths nearly equal their diameters. Explain why tropical cyclone depths are much less than their diameters. (Hint, consider Fig. 16.3)
E3. If you could see movie loops of satellite images for the same storms shown in Figs. 16.1 and 16.4 in the Northern Hemisphere, would you expect these satellite loops to show the tropical cyclone clouds to be rotating clockwise or counterclockwise? Why?
E4. Speculate on why tropical cyclones can have eyes, but mid-latitude supercell thunderstorms do not?
E5. If a tropical cyclone with max sustained wind speed of 40 m s–1 contains tornadoes that do EF4 damage, what Saffir-Simpson Wind Scale category would you assign to it? Why?
E6. Why don’t tropical cyclones or typhoons hit the Pacific Northwest coast of the USA and Canada?
E7. The INFO box on tropical cyclone-induced Currents in the ocean shows how Ekman transport can lower sea level under a tropical cyclone. However, we usually associate rising sea level with tropical cyclones. Why?
E8. Consider Fig. 16.12. Should there also be a jet along the north edge of the hot Saharan air? If so, explain its characteristics. If not, explain why.
E9. Fig. 16.14a shows wind moving from east to west through an easterly wave. Fig. 16.14b shows the whole wave moving from east to west. Can both these figures be correct? Justify your answer.
E10. In the Extratropical Cyclone chapter, troughs were shown as southward meanders of the polar jet stream. However, in Fig. 16.14 the troughs are shown as northward meanders of the trade winds. Explain this difference. Hint, consider the General Circulation.
E11. Compare and contrast a TUTT with mid-latitude cyclone dynamics as was discussed in the Extratropical Cyclone chapter.
E12. Consider Fig. 16.16. If a typhoon in the Southern Hemisphere was blown by the trade winds toward the Northern Hemisphere, explain what would happen to the tropical cyclone as it approaches the equator, when it is over the equator, and when it reaches the Northern Hemisphere. Justify your reasoning.
E13. Fig. 16.17 suggests that a cold front can help create a tropical cyclone, while Fig. 16.23 suggests that a cold front will destroy a tropical cyclone. Which is correct? Justify your answer. If both are correct, then how would you decide on tropical cyclogenesis or cyclolysis?
E14. In the life cycle of tropical cyclones, Mesoscale Convective Systems (MCSs) are known to be one of the possible initial stages. But MCSs also occur over the USA, as was discussed in the Thunderstorm chapter. Explain why the MCSs over the USA don’t become tropical cyclones.
E15. Based on the visible satellite image of Fig. 16.19, compare and contrast the appearance of the tropical cyclone at (c) and the tropical storm at (d). Namely, what clues can you use from satellite images to help you decide if the storm has reached full tropical cyclone strength, or is likely to be only a tropical storm?
E16. What are the other large cloud areas in Fig. 16.19 that were not identified in the caption? Hint, review the Satellites & Radar chapter.
E17. The Bermuda High (or Azores High) as shown in Fig. 16.20 forms because the ocean is cooler than the surrounding continents. However, tropical cyclones form only when the sea-surface temperature is exceptionally warm. Explain this contradiction.
E18. If global warming allowed sea-surface temperatures to be warmer than 26.5°C as far north as 60°N, could Atlantic tropical cyclones reach Europe? Why?
E19. Could a tropical cyclone exist for more than a month? Explain.
E20. a. What is the relationship between angular momentum and vorticity?
b. Re-express eq. (16.1) as a function of vorticity.
E21. From the Atmospheric Forces & Winds chapter, recall the equation for the boundary-layer gradient wind. Does this equation apply to tropical cyclone boundary layers? If so what are it’s limitations and characteristics.
E22. The top of Fig. 16.24 shows weak low pressure in the eye surrounding by high pressure in the eyewall at the top of the storm. Explain why the storm cannot have high pressure also in the eye.
E23. Fig. 16.25 shows the wind vector M parallel to the acceleration vector Fnet. Is that realistic? If not, sketch the likely vectors for M and Fnet. Explain.
E24. The words “supergradient winds” means winds faster that the gradient wind speed. Are the outflow winds at the top of a tropical cyclone supergradient? Justify your answer.
E25.(§) Using relationships from Chapter 1, plot an environmental pressure profile vs. height across the troposphere assuming an average temperature of 273 K. Assume the environmental sea-level pressure is 100 kPa. On the same graph, plot the pressure profile for a warm tropical cyclone core of average temperature (K): a. 280 b. 290 c. 300 assuming a sea-level pressure of 95 kPa. Discuss.
E26. Suppose that the pressure-difference magnitude between the eye and surroundings at the tropical cyclone top is only half that at the surface. How would that change, if at all, the temperature model for the tropical cyclone? Assume the sea-level pressure distribution is unaltered.
E27. Create a table that has a column listing attributes of mid-latitude cyclones, and another column listing attributes of tropical cyclones. Identify similarities and differences.
E28. The circles in Fig. 16.29 illustrate the hypsometric situation applied to the warm core of a tropical cyclone. Why cannot the hypsometric equation explain what drives subsidence in the eye?
E29. In Fig. 16.30b, why does the Td line from Point 1 to Point 2 follow a contour that slopes upward to the right, even though the air parcel in Fig 16.30a is moving horizontally, staying near sea level?
E30. a. Re-express eq. (16.7) in terms of potential temperature.
b. Discuss the relationship between entropy and potential temperature.
E31. What factors might prevent a tropical cyclone from being perfectly efficient at extracting the maximum possible mechanical energy for any given thermodynamic state?
E32. For the tropical cyclone model given in this chapter, describe how the pressure, tangential velocity, radial velocity, vertical velocity, and temperature distribution are consistent with each other, based on dynamic and thermodynamic relationships. If they are not consistent, quantify the source and magnitude of the discrepancy, and discuss the implications and limitations. Consider the idealizations of the figure below. (ABL = atmos. boundary layer.)
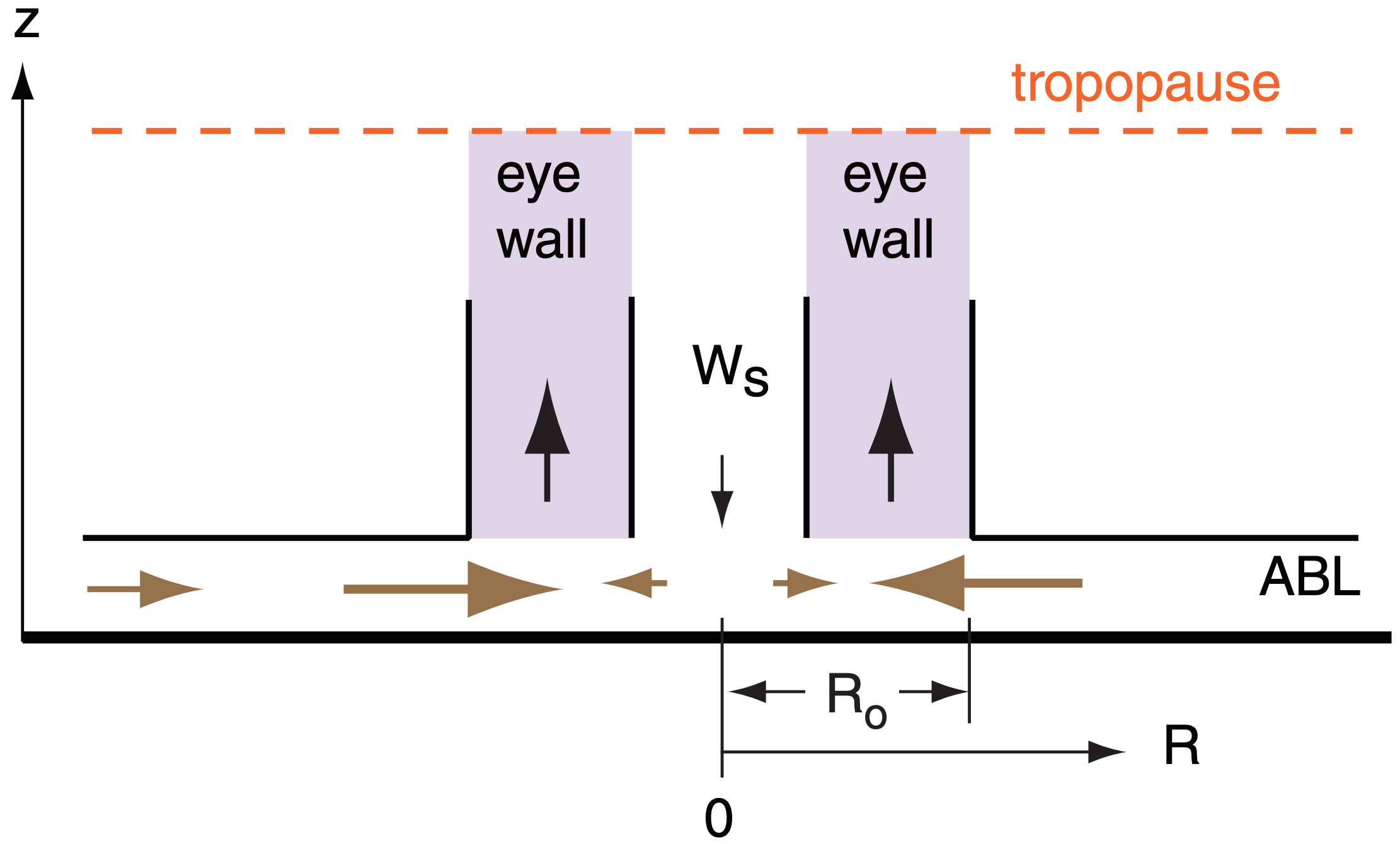
Hints: a. Use the cyclostrophic relationship to show that tangential velocity is consistent with the pressure distribution.
b. Use mass conservation for inflowing air trapped within the boundary layer to show how radial velocity should change with R, for R > Ro.
c. Assuming wave drag causes radial velocity to be proportional to tangential velocity squared, show that the radial velocity and tangential velocity equations are consistent. For R < Ro, use the following alternate relationship based on observations in tropical cyclones: Mtan/Mmax = (R/Ro)2.
d. Rising air entering the bottom of the eye wall comes from two sources, the radial inflow in the boundary layer from R > Ro, and from the subsiding air in the eye, which hits the ground and is forced to diverge horizontally in order to conserve mass. Combine these two sources of air to compute the average updraft velocity within the eye wall.
e. Use mass continuity in cylindrical coordinates to derive vertical velocity from radial velocity, for R < Ro. (Note, for R > Ro, it was already assumed in part (a) that air is trapped in the ABL, so there is zero vertical velocity there.)
f. Use the hypsometric relationship, along with the simplifications described in the temperature subsection of the tropical cyclone model, to relate the radial temperature distribution (averaged over the whole tropical cyclone depth) to the pressure distribution.
E33. An article by Willoughby and Black (1996: tropical cyclone Andrew in Florida: dynamics of a disaster, Bull. Amer. Meteor. Soc., 77, 543-549) shows tangential wind speed vs. radial distance.
a. For their Figs. 3b and 3d, compare their observations with the tropical cyclone model in this chapter.
b. For their Figs. 3e - 3g, determine translation speed of the tropical cyclone and how it varied with time as the storm struck Florida.
E34. To reduce fatalities from tropical cyclones, argue the pros and cons of employing better mitigation technology and the pros and cons of population control. Hint: Consider the whole world, including issues such as carrying capacity (i.e., the finiteness of natural resources and energy), sustainability, politics and culture.
E35. In Fig. 16.41, one relative maximum (A) of storm-surge height is directly in front of the storm’s path, while the other (B) is at the right front quadrant. Explain what effects could cause each of these surges, and explain which one might dominate further from shore while the other might dominate when the tropical cyclone is closer to shore.
E36. Fig. 16.43 for a storm surge caused by Ekman transport is for a tropical cyclone just off the east coast of a continent. Instead, suppose there was a tropical cyclone-force cyclone just off the west coast of a continent at mid-latitudes.
a. Would there still be a storm surge caused by Ekman transport?
b. Which way would the resulting Kelvin wave move (north or south) along the west coast?
E37. Devise a mathematical relationship between the Saffir-Simpson tropical cyclone Wind scale, and the Beaufort wind scale.
E38. Estimate CDP using the data in Fig. 16.34.
16.11.4. Synthesize
S1. What if the Earth rotated twice as fast. Describe changes to tropical cyclone characteristics, if any.
S2. What if the average number of tropical cyclones tripled. How would the momentum, heat, and moisture transport by tropical cyclones change the global circulation, if at all?
S3. Some science fiction novels describe “supercanes” with supersonic wind speeds. Are these physically possible? Describe the dynamics and thermodynamics necessary to support such a storm in steady state, or use the same physics to show why they are not possible.
S4. Suppose the tropical tropopause was at 8 km altitude, instead of roughly 16 km altitude. How would tropical cyclone characteristics change, if at all?
S5. What if the Earth’s climate was such that the tropics were cold and the poles were hot, with sea surface temperature greater than 26°C reaching from the poles to 60° latitude. Describe changes to tropical cyclone characteristics, if any
S6. Suppose that the sea surface was perfectly smooth, regardless of the wind speed. How would tropical cyclone characteristics change, if at all?
S7. Is it possible to have a tropical cyclone without a warm core? Be aware that in the real atmosphere, there are tropical cyclone-force cyclones a couple times a year over the northern Pacific Ocean, during winter.
S8. Suppose that the thermodynamics of tropical cyclones were such that air parcels, upon reaching the top of the eye wall clouds, do not loose any heat by IR cooling as they horizontally diverge away from the top of the tropical cyclone. How would the Carnot cycle change, if at all, and how would that affect tropical cyclone intensity?
S9. Is it possible for two tropical cyclones to merge into one? If so, explain the dynamics and thermodynamics involved. If so, is it likely that this could happen? Why?