10.1.7: Symmetry Is a Property

Last updated
Save as PDF

Page ID: 18472

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Mineralogists often speak of a crystal’s symmetry elements – the different kinds of symmetry a crystal has – in quantitative terms. We can count mirror planes, rotation axes, and inversion centers. If objects have only a few symmetry elements, we say they have low symmetry. Those that display many have high symmetry. In addition, we say that objects with 6-fold rotation axes have higher symmetry than those with 4-fold, 3-fold, or 2-fold axes, and so on. References to high and low symmetry are necessarily vague because symmetry manifests itself in many different ways.

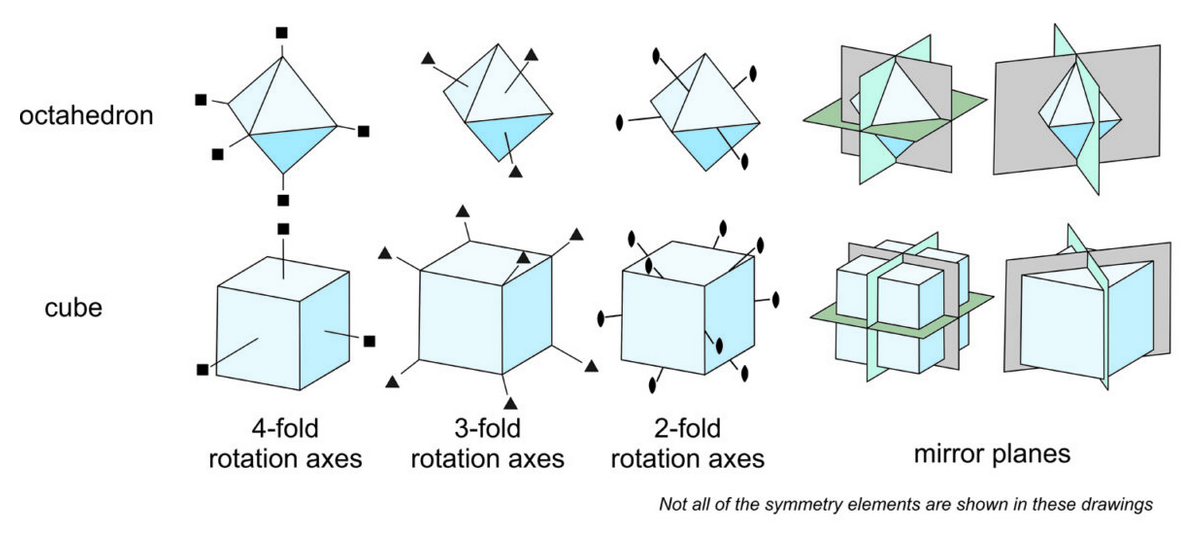
10.17.png — Figure 10.17: Symmetry of an octahedron and of a cube. In this and other drawings in this chapter, squares, triangles, and lens shapes designate 4-fold, 3-fold, and 2-fold rotation axes. When present, a hexagon designates a 6-fold axis.

Cubes have the most symmetry possible for crystals: three 4-fold axes, four 3-fold axes, six 2-fold axes, nine mirror planes, and an inversion center. All this symmetry is shown in Figure 10.17. But, octahedra have the same symmetry (also shown in Figure 10.17). Consider the three 4-fold rotation axes. In a cube they are perpendicular to faces. In an octahedron, they come out at the corners. But, in both, these axes are at 90^o to each other. And the four 3-fold axes exit the cube at the corners, but exit the octahedron in the centers of the faces. The 2-fold axes come out of the centers of edges in both crystals. The right side of the figure shows only some of mirror planes because showing them all is too complicated. If you look closely, you will see that the ones shown have identical orientations in both crystals.

A cube has 12 edges, 8 corners and 6 faces. An octahedron has 12 edges, 8 faces and 6 corners. It is as if the faces and corners have switched places. And, both crystals have exactly the same symmetry. Cube faces are at 90^o to each other; octahedron faces are at about 55° to each other, but the mirror planes, rotation axes, and the inversion center are identical for both.

Comparison of the cube and octahedron emphasizes that symmetry is not a physical characteristic. It is a property that objects may possess. With an object in hand, a mineralogist can discuss its symmetry, but a description of an object’s symmetry does not unambiguously reveal its appearance. Many objects have mirror planes, yet their overall shapes are quite different. While symmetry does not determine appearance, it does include all aspects of a crystal.